Evaluate ∫x5x2−2dx Solution in Tamil
The correct Answer is:D
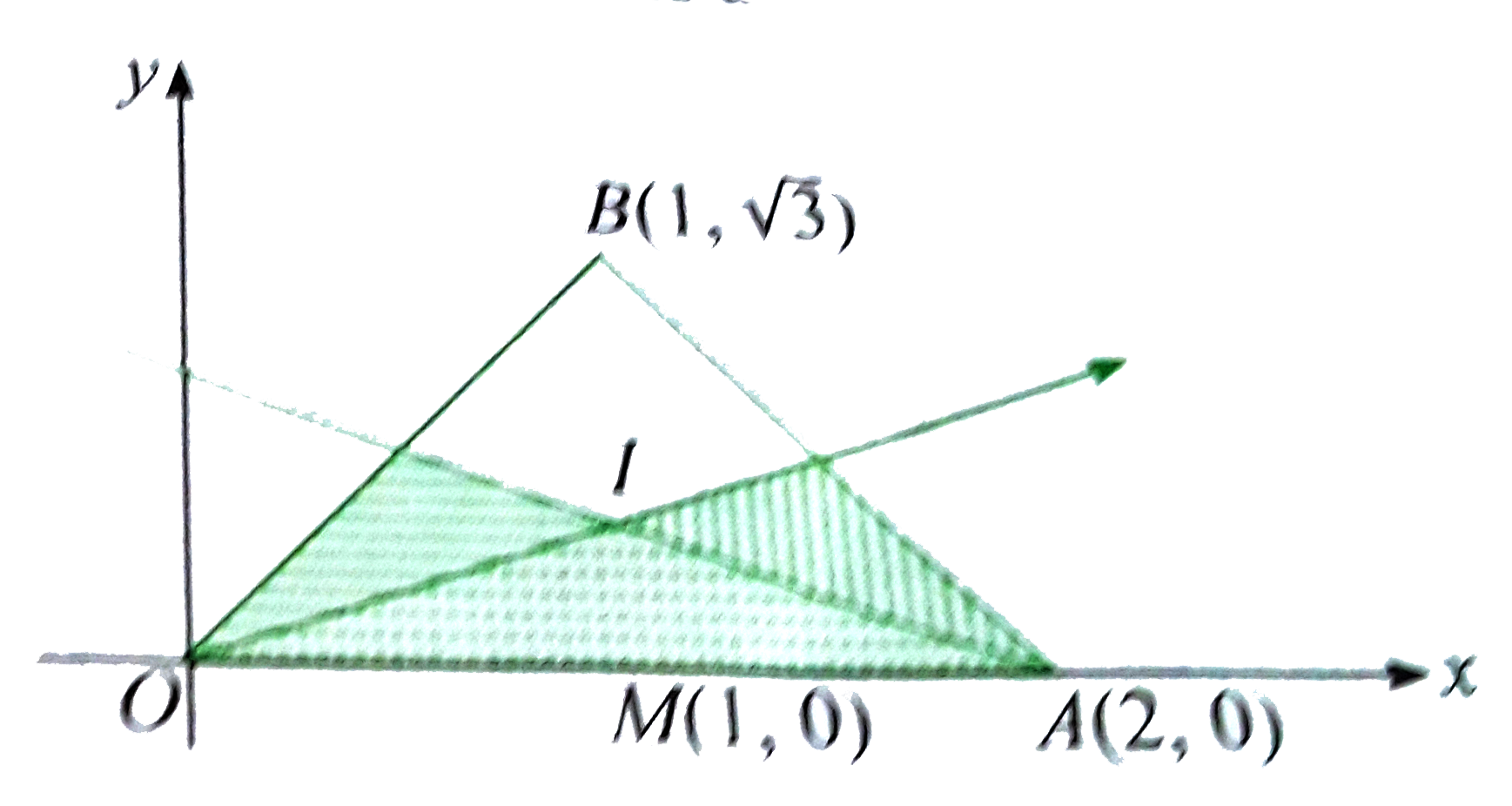
d(P,OA)≤min[d(P,OB),d(P,AB)]
or d(P,OA)≤(P,OB)
and d(P,OA0)≤d(P,AB)
When d(P,OA)P is equidistant from OA and OB, or P lies on the bisector of lines OA and OB.
Hence, when d(P,OA)≤d(P,OB), point P is nearer to OA than to OB or lies below the angle bisector of ∠AOB. Similarly, when d(P,OA)≤d(P,AB)P is nearer to OA than to AB, or P lies below the bisector of ∠OABandAB. Therefore, the required area is equal to the area of ΔOIA. Now,
tan∠BOA=√31=√3
or ∠BOA=60∘
Hence, the triangle is equilateral. then I coincides. with the centroid which is (1,1/√3).
Therefore,the area of ΔOIA is
12OA×IM=12×2×1√3=1√3sq.units
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Matrix)
4 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Numerical)
12 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Multiple)
13 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Consider the triangle whose vertices are (-1,0),(5,-2) and (8,2). Find the centroid of the triangle.
View SolutionLine AB passes through point (2,3) and intersects the positive x and y-axes at A(a,0) and B(0,b) respectively. If the area of ΔAOB is 11. then the value of 4b2+9a2 is
View SolutionIf (−6,−4),(3,5),(−2,1) are the vertices of a parallelogram, then the remaining vertex can be (0,−1) (b) 7,9) (−1,0) (d) (−11,−8)
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionEvaluate:
(i) ∫x2+5x+2x+2
(ii) ∫x3x−2 dx
View SolutionEvaluate ∫x2+1x2−5x+6dx
View SolutionFind ∫x2+1x2−5x+6dx
View SolutionFind ∫x2+1x2−5x+6dx
View SolutionFind ∫x2+1x2−5x+6dx
View SolutionFind ∫x2+1x2−5x+6dx
View SolutionEvaluate : ∫sinx(2+5x)dx.
View SolutionEvaluate : ∫x+2√x2+5x+6dx.
View SolutionEvaluate ∫5x−2dx
View SolutionEvaluate: (i) ∫x2+5x+2x+2 (ii) ∫x3x−2 dx
View SolutionEvaluate: ∫x2+1x2−5x+6dx
View Solution
