Point A and B are in the first quadrant; point O is the origin. If the slope of OA is 1, the slope of OB is 7, and OA=OB, then the slope of AB is a.−15 (b) −14 (c) −13 (d) −12Solution in Tamil
−1/5
−1/4
−1/3
−1/2
The correct Answer is:D
tanθ=7
OA=OB=r
sinθ=75√2cosθ=15√2
Now, mAB=1/2.
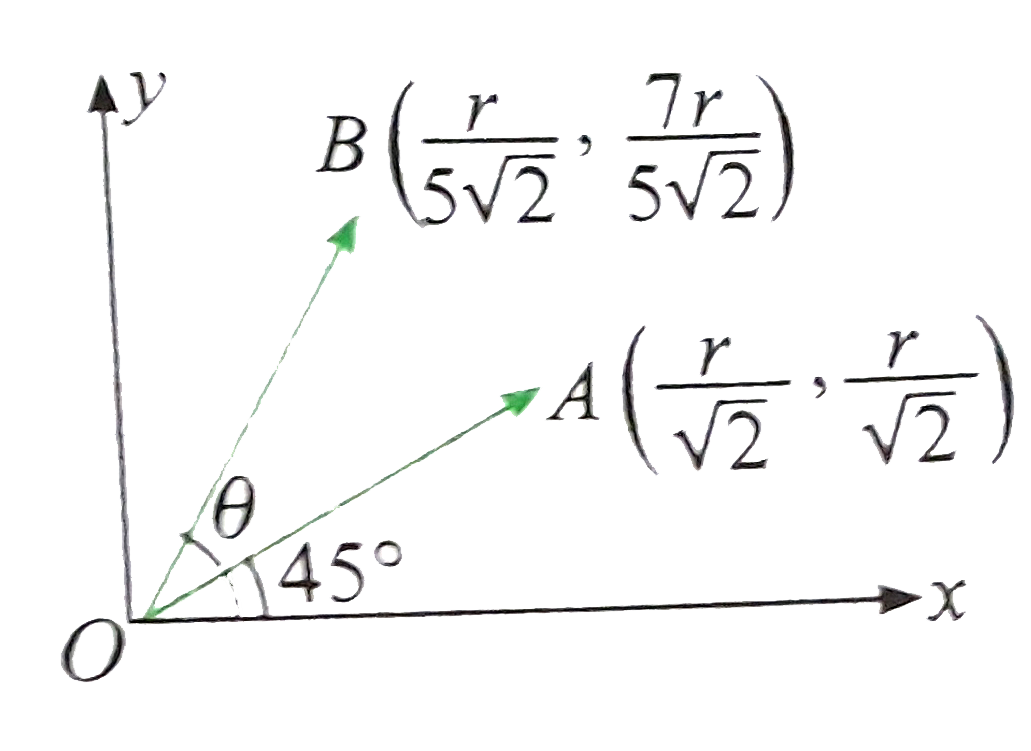
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Multiple)
13 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Comprehension)
10 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.6
9 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
If (−6,−4),(3,5),(−2,1) are the vertices of a parallelogram, then the remaining vertex can be (0,−1) (b) 7,9) (−1,0) (d) (−11,−8)
View SolutionStudy the diagram. The line l is perpendicular to line m Does PE bisect CG?
View SolutionFind the locus of a point whose distance from (a, 0) is equal to its distance from the y-axis.
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionPoint A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is: a. -1/5 b. -1/4 c. -1/3 d. -1/2
View SolutionPoint A and B are in the first quadrant; point O is the origin. If the slope of OA is 1, the slope of OB is 7, and OA=OB, then the slope of AB is −15 (b) −14 (c) −13 (d) −12
View SolutionFind the value of λ so that the points P,Q,R and S on the sides OA,OB,OC and AB, respectively, of a regular tetrahedron OABC are coplanar. It is given that OPOA=13,OQOB=12,OROC=13 and OSAB=λ. (A) λ=12 (B) λ=−1 (C) λ=0 (D) for no value of λ
View SolutionABCD IS A PARALLELOGRAM AND O is any point in its interior. Prove that: ar (AOB)+ ar (COD)= ar (BOC)+ ar(AOD) ar (AOB)+ar (COD)=12 ar(^(gm)ABCD) Given: A parallelogram ABCD and O is a point in its interior. To Prove: ar ( AOB)+ ar(COD)=ar (BOC)+ar(AOD)
View SolutionIf O is the origin and A(2, 3, 1), B(1, 1, -5) are two given points then show that the straight line OA is perpendicular to the straight line OB.
View Solutionयदि A और B दो बिंदुओं के निर्देशांक क्रमशह (−1,3,4) और (1,−1,1) हो और O मूल बिंदु हो, तो सिद्ध कीजिए की OA और OB परस्पर लम्ब है।
View SolutionA and B are two points on the hyperbola O is the centre. If OA is perpendicular to OB then 1(OA)2+1(OB)2 is equal to
View SolutionPoint A and B are in the first quadrant; point O is the origin. If the slope of OA is 1, the slope of OB is 7, and OA=OB, then the slope of AB is −15 (b) −14 (c) −13 (d) −12
View SolutionFind the value of λ so that the points P,Q,R and S on the sides OA,OB,OC and AB, respectively, of a regular tetrahedron OABC are coplanar. It is given that OPOA=13,OQOB=12,OROC=13 and OSAB=λ. (A) λ=12 (B) λ=−1 (C) λ=0 (D) for no value of λ
View SolutionPoint A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is: a. -1/5 b. -1/4 c. -1/3 d. -1/2
View SolutionIn Figure, AOOC=BOOD=12 and AB=5cm . Find the value of DC.
View Solution
