A line of fixed length 2 units moves so that its ends are on the positive x-axis and that part of the line x+y=0 which lies in the second quadrant. Then the locus of the midpoint of the line has equation. a.x2+5y2+4xy−1=0 b. x2+5y2+4xy+1=0 c.x2+5y2−4xy−1=0 d.4x2+5y2+4xy+1=0Solution in Tamil
x2+5y2+4xy−1=0
x2+5y2+4xy+1=0
x2+5y2−4xy−1=0
x2+5y2−4xy−1=0
The correct Answer is:A
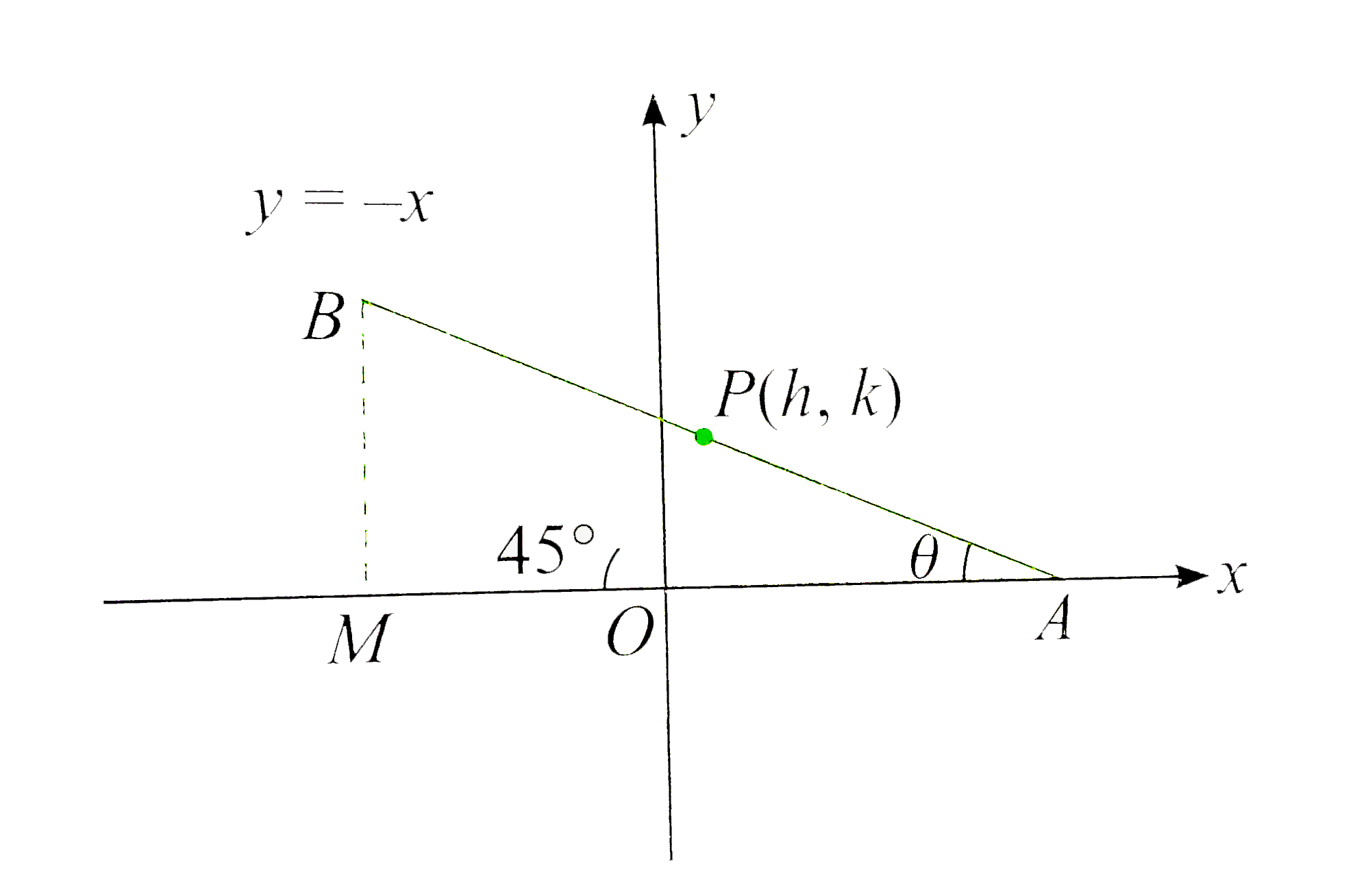
As shown in the figure, line segment AB, of fixed length 2 units, slides such that one of its ends A is on x-axis and other end B is on line x+y=0.
Let P(h,k) be midpoint of AB.
Also, let ∠BAO=θ.
So, in triangle AMB, BM = 2sinθ and AM=2cosθ.
In triangle BMO, MO=MB = 2sinθ
∴A≡(2cos θ−2sinθ,0) and B≡(−2 sinθ,2sin θ)
Now, P(h,k) is the midpoint of AB.
∴2h=2cos θ−2sinθ+(−2 sinθ) and 2k=2sin θ
⇒h=cos θ−2sinθ and k=sin θ
⇒h+2k=cos θ and k=sin θ
Squaring and adding, we get
(h+2k)2+k2=1
or x2+5y2+4xy−1=0
This is the required locus.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Multiple)
30 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Comprehension)
27 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.6
5 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
If P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
View SolutionLet L be the line belonging to the family of straight lines (a+2b) x+(a-3b)y+a-8b =0, a, b ∈R, which is the farthest from the point (2, 2).
Area enclosed by the line L and the coordinate axes is
View SolutionIf aandb are two arbitrary constants, then prove that the straight line (a−2b)x+(a+3b)y+3a+4b=0 will pass through a fixed point. Find that point.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionA line of fixed length 2 units moves so that its ends are on the positive x-axis and that part of the line x+y=0 which lies in the second quadrant. Then the locus of the midpoint of the line has equation. (a) x2+5y2+4xy−1=0 (b) x2+5y2+4xy+1=0 (c) x2+5y2−4xy−1=0 (d) 4x2+5y2+4xy+1=0
View SolutionThe equation of the circle passing through the point of intersection of the circle x2+y2=4 and the line 2x+y=1 and having minimum possible radius is (a)5x2+5y2+18x+6y−5=0 (b) 5x2+5y2+9x+8y−15=0 (c) 5x2+5y2+4x+9y−5=0 (c)5x2+5y2−4x−2y−18=0
View SolutionIf common chord of cicles x2+y2+5kx+2y+k=0 and x2+y2+kx+y2+12=0 is 4x−5y−k=0
then number of values of k is
View Solution(x^(2)+4x)^(2)-5(x^(2)+4x)-y(x^(2)+4x)+5y
View SolutionA circle touching the line x+y−2=0 at (1,1) and cuts the circle x2+y2+4x+5y−6=0 at P and Q. Then
View SolutionThe equation of the diameter of the circle x2+y2−4x−6y+3=0 that is perpendicular 2x+5y−6=0 is
View SolutionIf the combined equation of the diagonals of the square formed by the pairs of lines xy + 4x - 5y - 20 = 0 and xy - 5x + 4y - 20 =0 is x2−y2−kx+ly=0 , then k+l =
View SolutionFind the equation of the line passing through the points of intersection of 4x-y-3=0 and x+y-2=0 and perpendicular to 2x-5y+3=0.
View SolutionA line of fixed length 2 units moves so that its ends are on the positive x-axis and that part of the line x+y=0 which lies in the second quadrant. Then the locus of the midpoint of the line has equation. (a) x2+5y2+4xy−1=0 (b) x2+5y2+4xy+1=0 (c) x2+5y2−4xy−1=0 (d) 4x2+5y2+4xy+1=0
View SolutionA line of fixed length 2 units moves so that its ends are on the positive x-axis and that part of the line x+y=0 which lies in the second quadrant. Then the locus of the midpoint of the line has equation.
View Solution
