In △ABC, vertex A is (1, 2). If the internal angle bisector of ∠B is 2x−y+10=0 and the perpendicular bisector of AC is y = x, then find the equation of BC Solution in Tamil
The image D of A in the bisector of ∠B lies on BC.
Now, the image of A(1,2) in the line 2x-y-10=0 is given by
x1−12=y1−2−1=−2(2−2+10)4+1
. ∴D(x1,y1)≡D(−7,6)
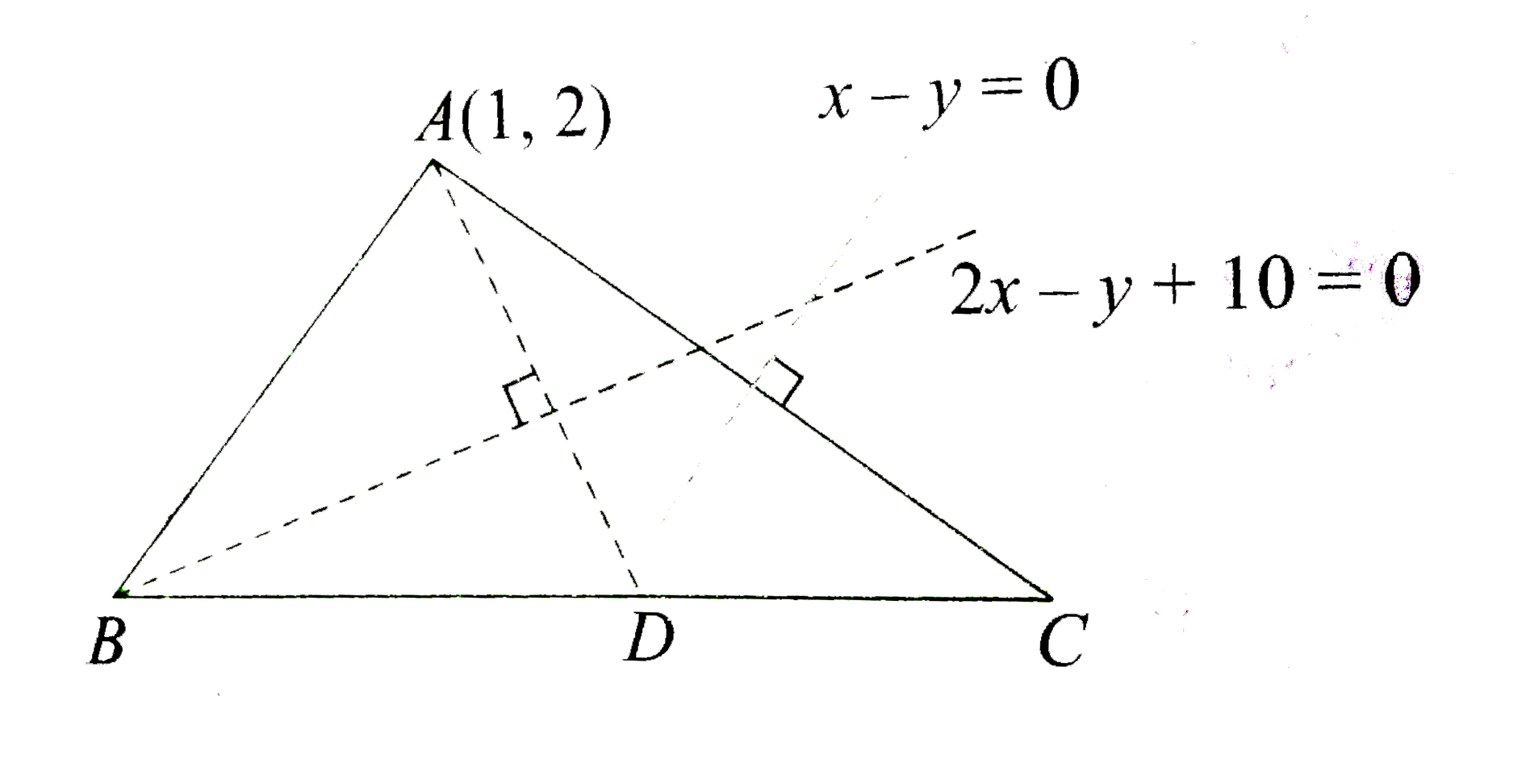
Also, C is the image of A in the perpendicular bisector of AC. The image of A(1,2) in y=x is C(2,1).
Hence the equation of line BC is
. y−1=6−1−7−2(x−2)
or 5x+9y-19=0
Topper's Solved these Questions
- STRAIGHT LINESBook:CENGAGEChapter:STRAIGHT LINESExercise:Exercise 2.1
Explore 23 Videos
- STRAIGHT LINESBook:CENGAGEChapter:STRAIGHT LINESExercise:Exercise 2.2
Explore 4 Videos
- STRAIGHT LINEBook:CENGAGEChapter:STRAIGHT LINEExercise:Multiple Correct Answers Type
Explore 8 Videos
- THEORY OF EQUATIONSBook:CENGAGEChapter:THEORY OF EQUATIONSExercise:JEE Advanced Previous Year
Explore 9 Videos
Similar Questions
Find the equation of the right bisector of the line segment joining the points (3,4) and (−1,2).
View SolutionFind the points on the line x + y = 1 that lie at a distance 3 units from the line 5x+12y = 3.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionIn /_ABC, vertex A is (1,2). If the internal angle bisector of /_B is 2x-y+10=0 and the perpendicular bisector of AC is y=x ,then find the equation of BC
View SolutionThe equations of the perpendicular bisector of the sides AB and perpendicular bisector of the sides AB and AC of a /_ABC are x-y+5=0 and x+2y=0 respectively,if the point Ais (1,-2), then the equation ofthe line BC is
View SolutionLet A,B,C be angles of triangles with vertex A≡(4,−1) and internal angular bisectors of angles B and C be x−1=0 and x−y−1=0 respectively.
Slope of BC is
View SolutionLet the equations of side BC and the internal angle bisector of angle B of ΔABC are 2x−5y+a=0 and y+x=0 respectively. If A=(2,3), then the value of of a is equal to
View SolutionIf the equations of the perpendicular bisectors of the sides AB and AC of a Δ ABC are x - y + 5 = 0 and x + 2y =0 respectively and if A is (1,-2), then the equation of the perpendicular bisector of the side BC is
View SolutionIf the equations of the perpendicular bisectors of the sides Ab and AC of a ΔABC are x−y+5=0andx+2y=0 respectively and if A is (1,−2), then the equation of the perpendicular bisector of the side BC is,
View SolutionThe equation of perpendicular bisectors of AB and AC of a triangle ABC are x-y-5=0 and x+2y=0 respectively. If A=(1,-2) then the equation of ¯¯¯¯¯¯BC is
View SolutionFind the equations of the bisectors of the angles formed by the following pairs of lines
x+2y+3=0 and 2x+y−2=0
View SolutionIn △ABC, vertex A is (1, 2). If the internal angle bisector of ∠B is 2x−y+10=0 and the perpendicular bisector of AC is y = x, then find the equation of BC
View SolutionIn △ABC, vertex A is (1, 2). If the internal angle bisector of ∠B is 2x−y+10=0 and the perpendicular bisector of AC is y = x, then find the equation of BC
View SolutionLet A,B,C be angles of triangles with vertex A≡(4,−1) and internal angular bisectors of angles B and C be x−1=0 and x−y−1=0 respectively.
Slope of BC is
View Solution
