Draw the graph of the function f:R−{−1,1}→R.f(x)=x1−|x|.Solution in Tamil

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

y=f(x)=x1−|x|={x1−x, x≥0, x≠1x1+x, x<0, x≠−1
y=x1−x=11−x−1
So (y+1)(x−1)=1, which is a rectangular hyperbola having centre at (1,-1).
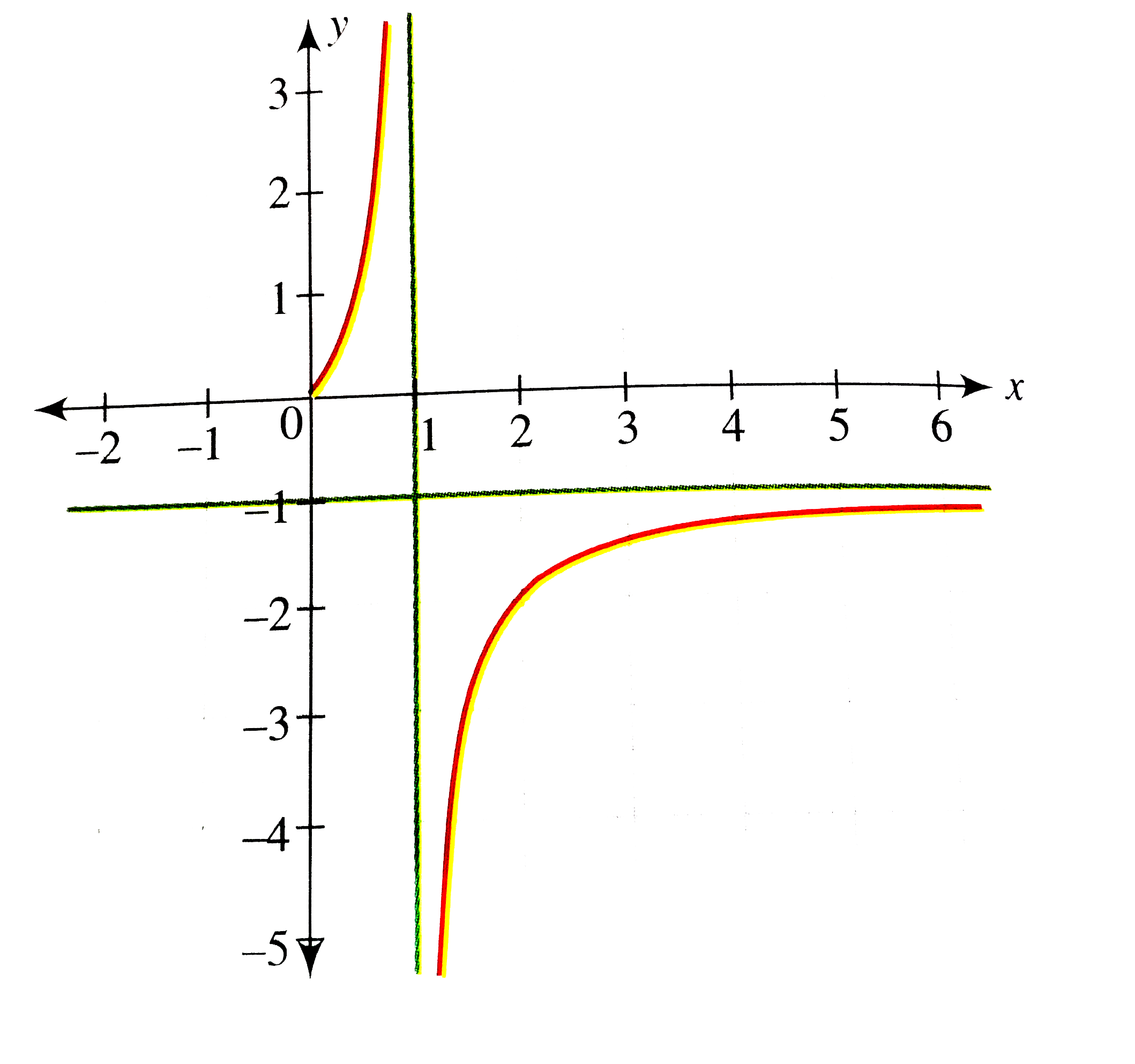
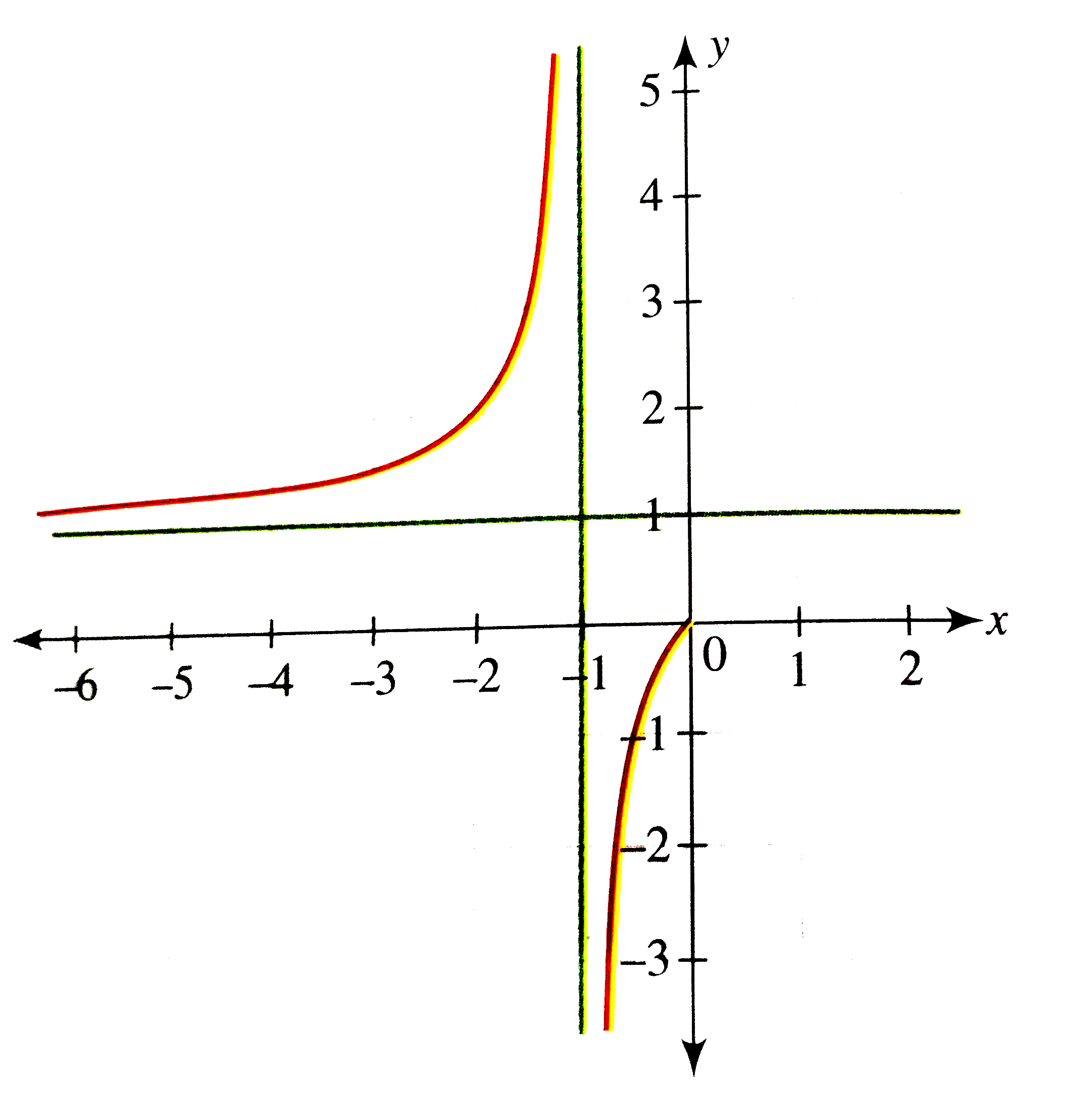
ocr_image
y=x1+x=1−11+x
So (y−1)(x+1)=1 , which is a rectangular having centre at (-1,1).
The graph is as shown in the following figure.
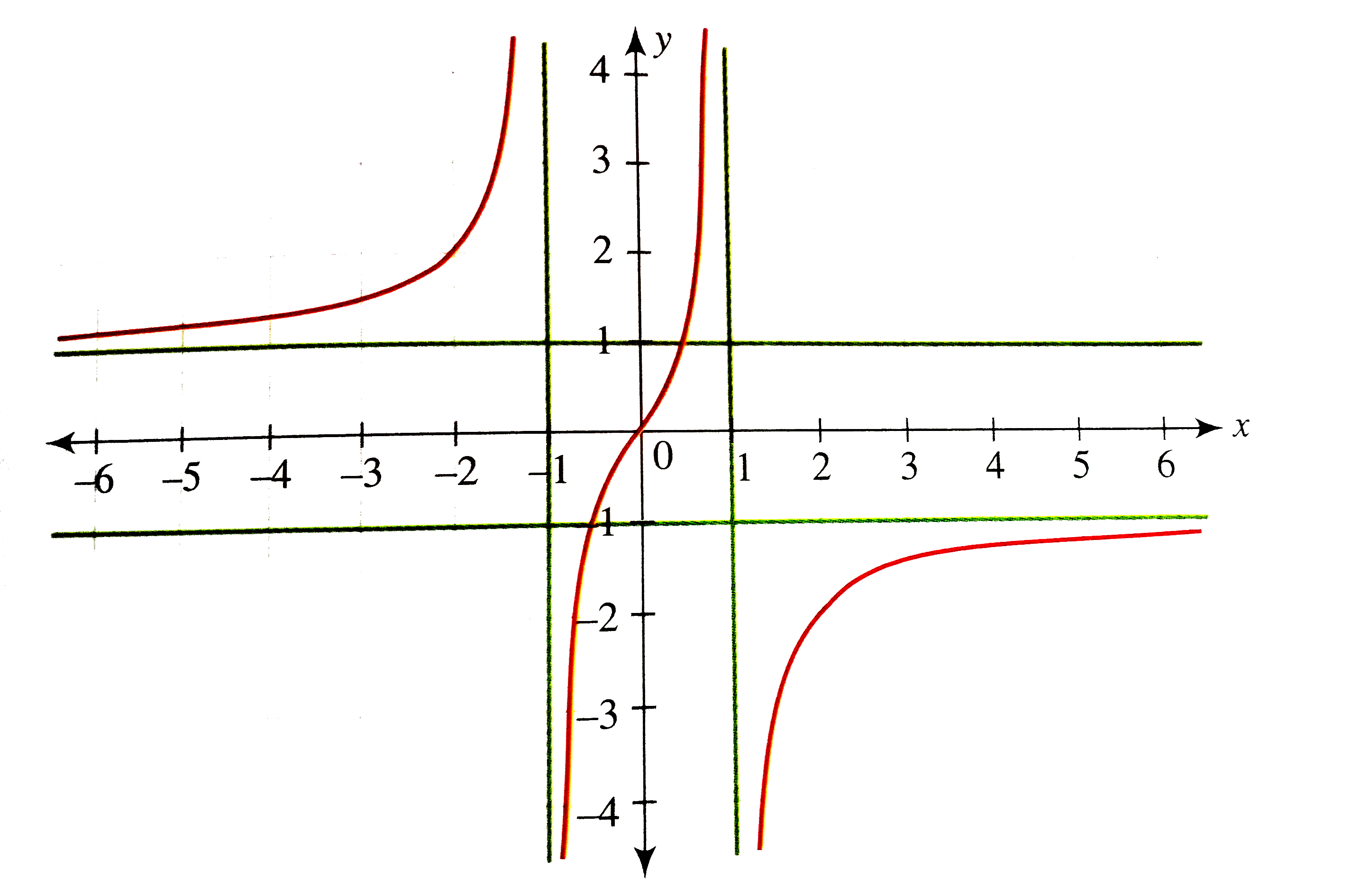
ocr_image
Combining two cases, we get the following graph.
ocr_image
Alternative method
y=f(x)=x1−|x|={x1−x, if x≥0, x≠1x1+x, if x<0, x≠−1
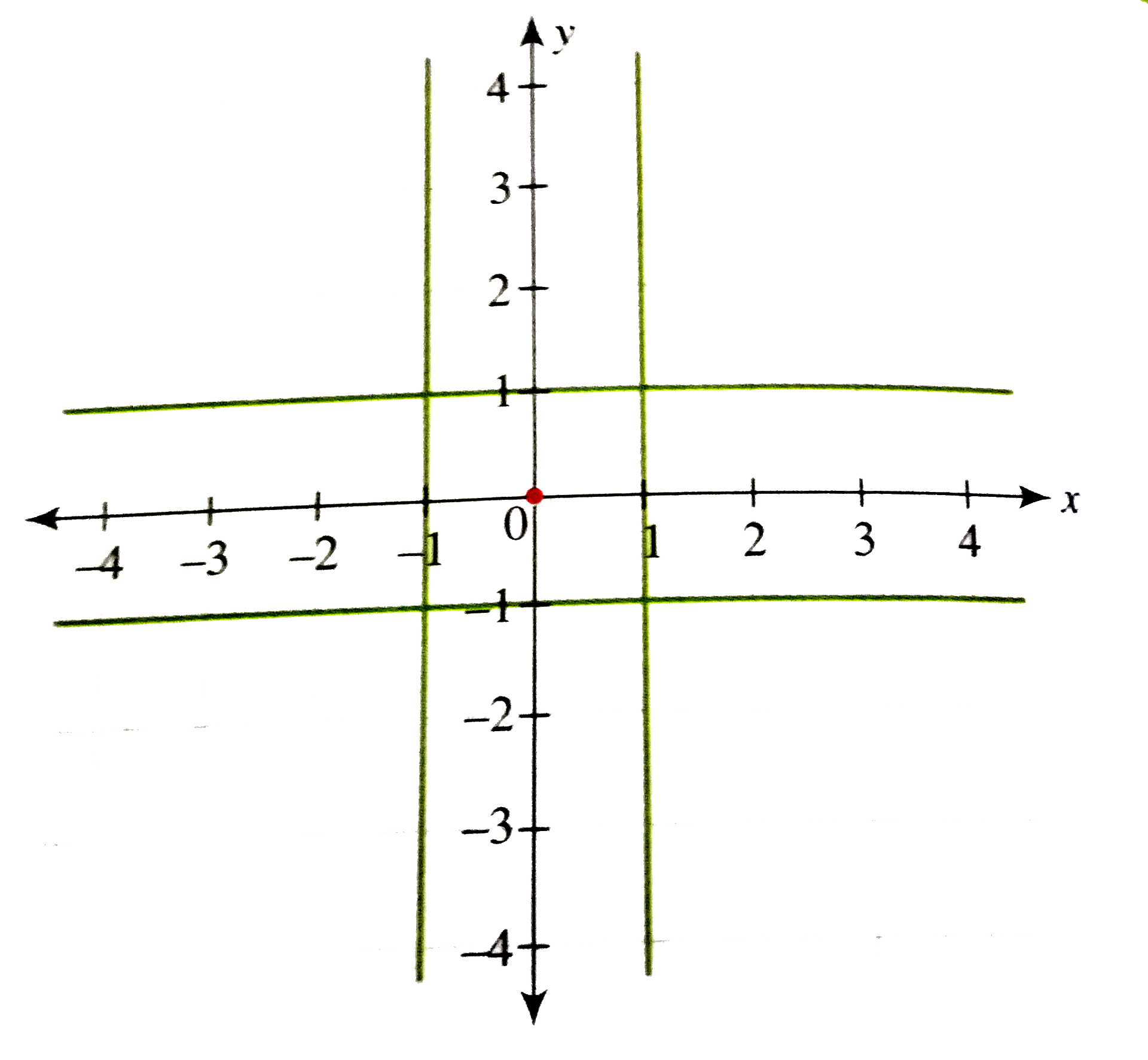
The function has vertical asymptotes as 1−|x|=0, or x=±1.
Also the function has horizontal asymptotes.
For x>0
y=limx→∞x1−x=limx→∞1x1x−1=−1
For x<0
y=limx→∞x1+x=limx→∞1x1x+1=1
Graph intersect axis at (0,0)
f'(x)=⎧⎪⎨⎪⎩1(1−x)2, x>0, x≠11(1+x)2, x<, x≠−1
∴ f'(x)>0,∀x∈R−{−1,1}
Hence f(x) is an increasing function.
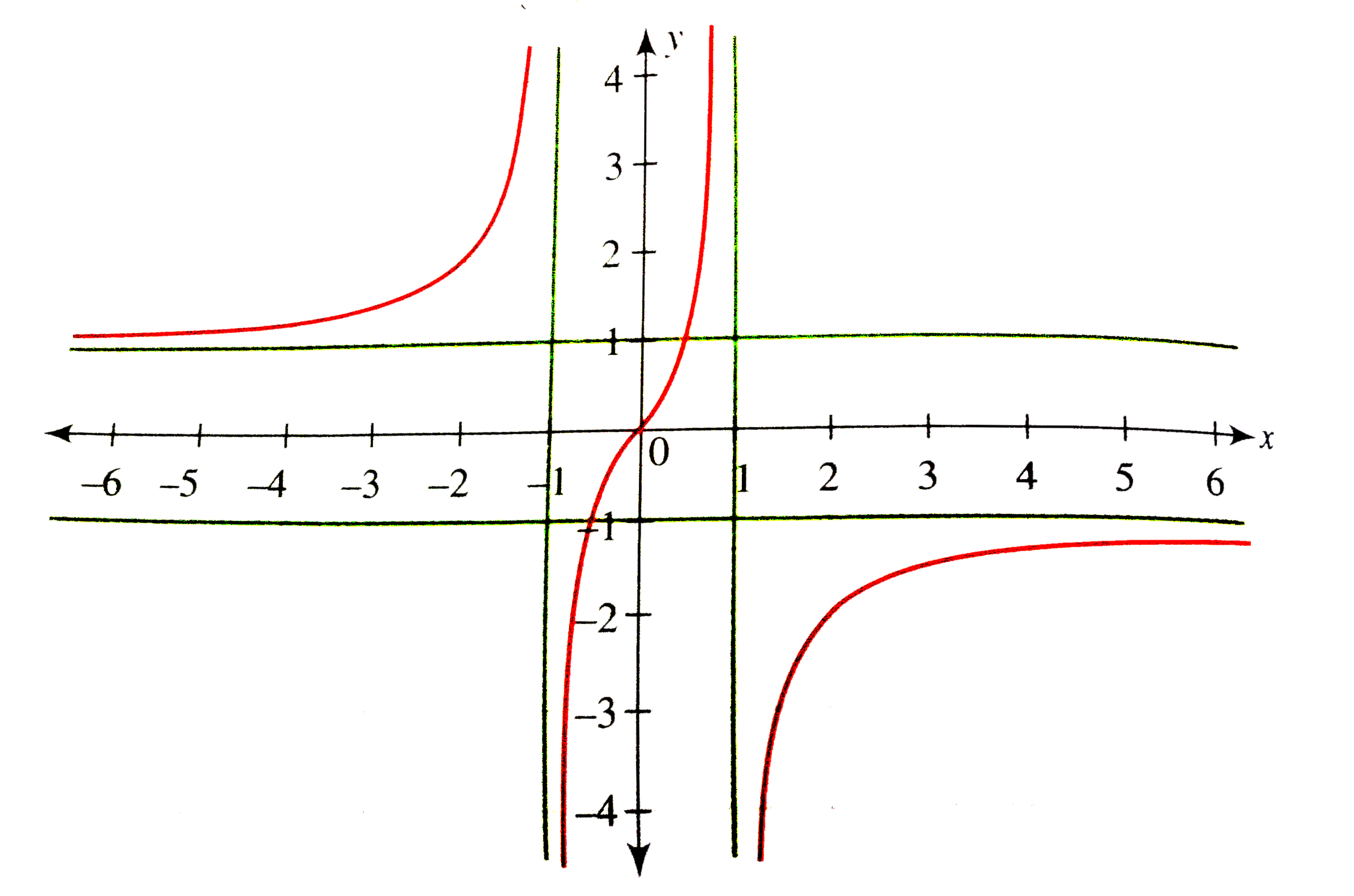
From the above discussion, we have the following reference points and lines.
ocr_image
In the above figure, we have three regions, x∈(−∞,−1),(−1,1),(1,∞).
In each region, f(x) in increasing.
limx→−1−x1−|x|→∞
limx→−1+x1−|x|→−∞ andlimx→1−x1−|x|→∞,f(0)=0
limx→1+x1−|x|→−∞ and limx→∞x1−|x|→1
Hence the graph for x∈(−∞,−1)∪(−1,1)∪(1,∞)is as shown in the following figure.
ocr_image

Updated on:21/07/2023
|
Class 12MATHSQUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions