The equivalent capacitances of the combination between points A and B in the given figure is 4μF.
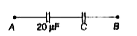
(i) Calculate capacitance of the capacitor C.
(ii) Calculate charge on each capacitor, if a 12 V battery is connected across terminals A and B.
(iii) What will be the potential drop across each capacitor ? Solution in Odia
According to the question, 20μF and C are connected in series.
(i) The equivalent capacitances
(4μF)=(20μF)×C20+C
(20+C)=5C
or 4C = 20
∴ C =5μF
(ii) Both capacitors are connected in series, so charge on both of them will be same and will be equal to the total charge.
∴ Charge on each capacitor,
Q=CV=4×12
=48μC
Same charge 48μC lines on both the capacitors
(iii) Potential drop across 20μF capacitor is given by
V1=QC1=48μC20=2.4V
Potential drop across 5μF capacitor is given by
V2=QC2=48μC5=9.6V
Similar Questions
Two capacitors of capacitances 4muF and 5muF are connected across a battery of 12 volts as shown in the adjoining figure (i). Calculate the total charge drawn from the battery.
View SolutionFour capacitors of capacitances 2 muF and 1muF are connected across a battery of 10V as shown in the figure (q). Calculate the net capacitance of the combination and net charge across each capacitor.
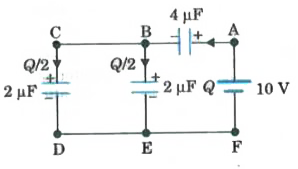
View SolutionThree capacitors of capacitances 2μF,2μFand4μF are connected to a battery of 10V as shown in the figure. Calculate the effective capacitance of the circuit and charge on each capacitor.
View SolutionTwo capacitors of capacitance 600 pF and 900 pF are connected in series across a 200 V supply. Calculate (i) the effective capacitance of the combination, (ii) the pd across each capacitor and (iii) the total charge stored in the system.
View SolutionThe equivalent capacitance of the combination between A and B in the given figure is 4 mu F . Calculate charge on each capacitor if a 12 V battery is connected across terminals A and B .
View SolutionThe equivalent capacitance of the combination between A and B in the given figure is 4 mu F . What will be the potential drop across each capacitor ?
View SolutionThe equivalent capacitance of the combination between A and B in the given Fig. is 4μF
(i) Calculate capacitance of capacitor C.
(ii) Calculate charge on each capacitor if a 12Vbattery is connected across terminals A and B.
(iii) What will be the potential drop across each capacitor ?

View SolutionA capacitor or capacitance C1 is charge to a potential V and then connected in parallel to an uncharged capacitor of capacitance C2. The fianl potential difference across each capacitor will be
View Solution
