Show that the height of a closed right circular cylinder of given surface and maximum volume, is equal to the diameter of its base.

Video Solution

Text Solution

Verified by Experts

Let r be the radius of base and h be the height of cylinder.
Therefore, its total surface area
$S = 2 π r h + 2 π r^{2}$ …(1)
If volume is V, then
$V = π r^{2} h$
$= π r^{2} (\frac{S - 2 π r^{2}}{2 π r})$ [From equation (1)]
$\frac{S}{2} r - π r^{3}$
$\Rightarrow \frac{d V}{d r} = \frac{S}{2} - 3 π r^{2}$
For maxima/minima $\frac{d V}{d r} = 0$
$\frac{S}{2} - 3 π r^{2} = 0$
$\Rightarrow \frac{2 π r h + 2 π r^{2}}{2} - 3 π r^{2} = 0$
$\Rightarrow π r h + π r^{2} - 3 π r^{2} = 0$
$\Rightarrow π r h = 2 π r^{2}$
$\Rightarrow h = 2 r$
and $\frac{d^{2} V}{d r^{2}} = 0 - 6 π r = - 6 π r < 0$
Therefore, volume is maximum at h = 2r.

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The height and radius of the right circular cylinder below are given in meters. What is the volume, in cubic meters, of the cylinder?

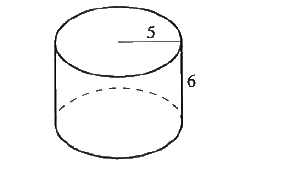A $30 π$
B $31 π$
C $150 π$
D $180 π$

NAGEEN PRAKASHAN ENGLISH-APPLICATIONS OF DERIVATIVES-Exercise 6.5

Home
Ask Doubt
Profile