Question

In a ${\displaystyle \Delta ABC}$ the value of ${\displaystyle \angle A}$ is given by 5 CosA - 3 = 0 then the equation whose roots are sinA and tanA is :

Explanation

We have,
`5cosA-3=0 impliescosA=(3)/(5)=("base")/("hypotenuse")`
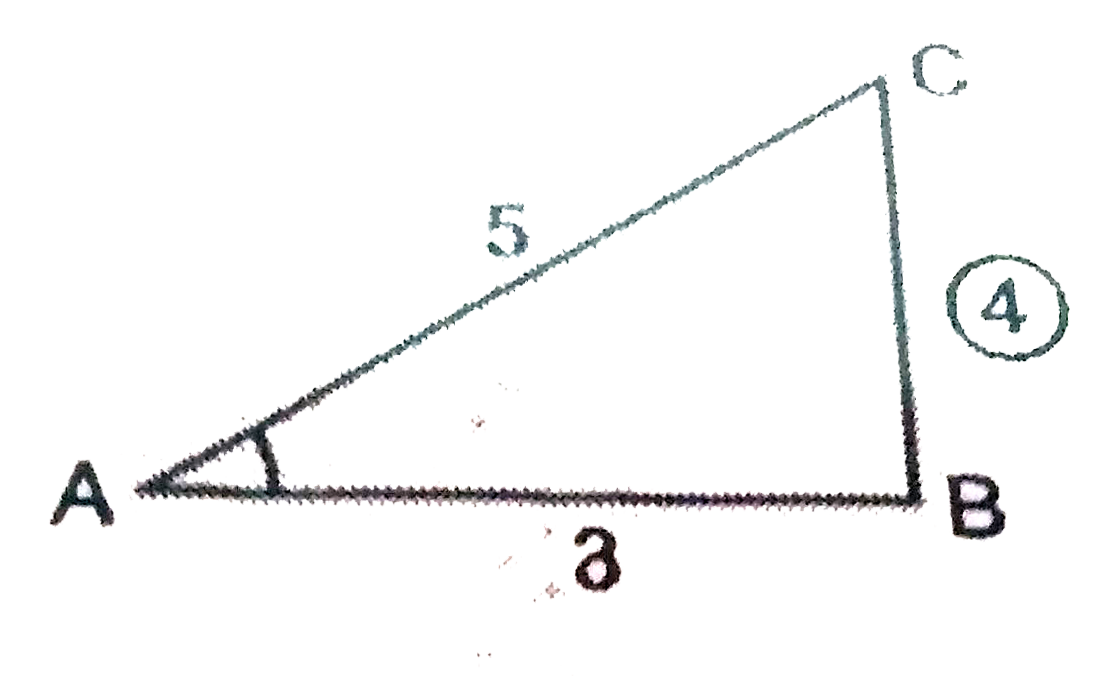
So `angleA` must be acute, Now make a base AB such that `angleB=90^(@)` and find the perpendicular by Pythagoras theorem.
`:.sinA(4)/(5)andtanA=(4)/(3)`
:. Quadratic equation whose roots are sin A and tan A, are
`x^(2)-(sinA+tenA)x+(sinA.tenA)=0" "(":'""equation is "x^(2)-S.x+P=0)`
`impliesx^(2)-((4)/(5)+(4)/(3))x+((4)/(5)xx(4)/(3))=0`
`impliesx^(2)-((12+20)/(15))x+(16)/(15)=0`
`implies15x^(2)-32x+16=0`