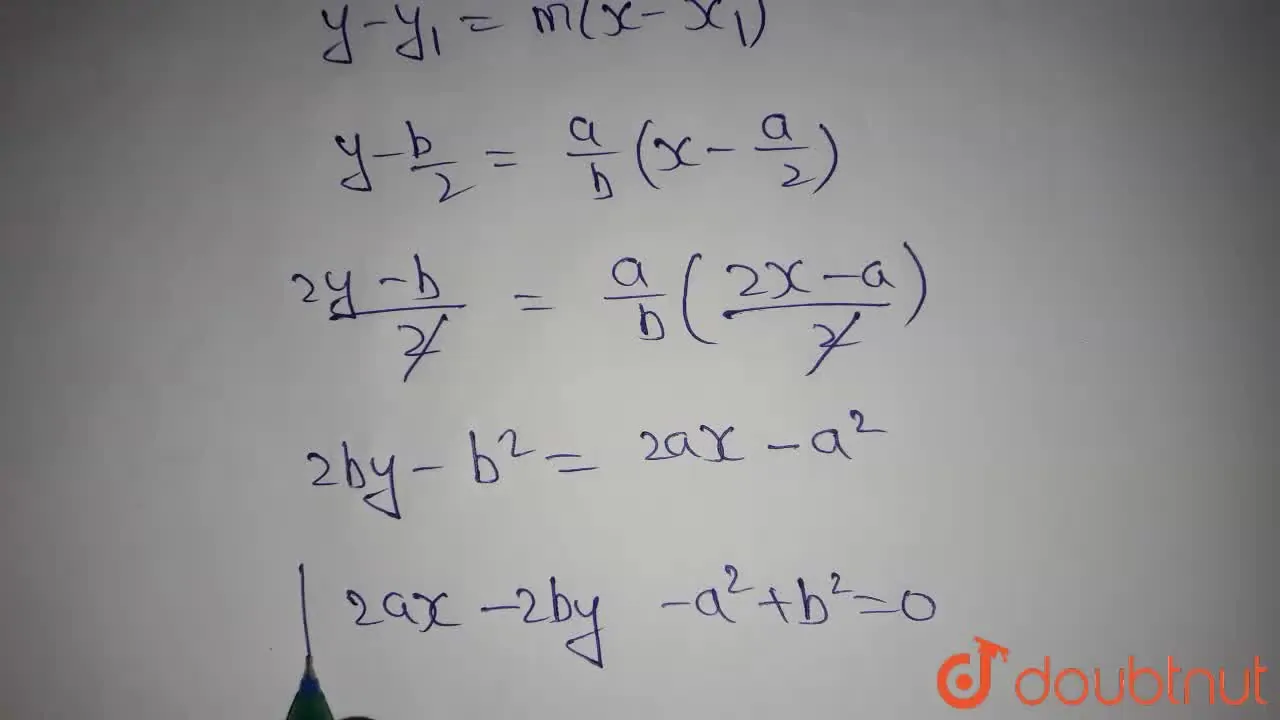Find the equation of a line perpendicular to the line xa+yb−1 and passes through the mid-point of the line segment lying between the axes of the given line.
Given straight line is
xa+yb=1
This line cuts the axes at points A(a,0) and B(0,b).
Co-ordinates of mid point of AB=(a+02,0+b2)
=(a2,b2)
Now, slope of given line
m1=−Coefficient of xCoefficient ofy=−1/a1/b=−ba
Slope of perpendicular line
m2=−1m1=−1b/a=ab
∴ Equation of line passes through (a2,b2) and whose slope is ab is
y−b2=ab(x−a2)
⇒2by−b2=2ax−a2
⇒2ax−2by=a2−b2.
Related Playlists
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Exercise207videos
STRAIGHT LINES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - MISCELLANEOUS EXERCISE7videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIGNOMETRIC FUNCTIONSEXERCISE - MISCELLANEOUS EXERCISE10videos
TRIGNOMETRIC FUNCTIONS
Similar Questions
Find the equation of a line perpendicular to the line x−2y+3=0and passing through the point (1,2).
View SolutionFind the equation of a line perpendicular to the line x−2y+3=0 and passing through the point (1,−2).
View SolutionFind the equation of the line perpendicular to the line xa−yb=1 and passing through a point at which it cuts the x-axis.
View SolutionFind the equation of the line perpendicular to the line xa−yb=1 and passing through a point at which it cuts the x-axis.
View SolutionThe equation of the line which perpendicular to the line x−2y+4=0 and passing through (1,2)
View SolutionFind the equation of the straight line perpendicular to 5x−2y=8 and which passes through the mid point of the line segment joining (2,3) and (4,5).
View SolutionFind the equation of a straight line drawn perpendicular to the line xa+yb=1 through the point where it meets the y-axis.
View SolutionFind the equation of the line which is perpendicular to the line xa−yb=1 at the point where this line meets y-axis.
View SolutionFind the equation to the line which is perpendicular to the line xa−yb=1 at the point where it meets the x-axis.
View SolutionFind the equation of the line parallel to the line 3x+2y=8 and passing through the point (0,1).
View Solution