Find the mean deviation about the median for the data : 36,72,46,42,60,45,53,46,51,49
To find the mean deviation about the median for the data set: 36,72,46,42,60,45,53,46,51,49, we will follow these steps:
Step 1: Arrange the data in ascending order
First, we need to arrange the given data in ascending order.
Data in ascending order:
36,42,45,46,46,49,51,53,60,72
Step 2: Find the median
Since there are 10 observations (an even number), the median is calculated as the average of the 5th and 6th observations.
- 5th observation = 46
- 6th observation = 49
Median calculation:
\(
\text{Median} = \frac{46 + 49}{2} = \frac{95}{2} = 47.5
\)
Step 3: Calculate the deviations from the median
Next, we calculate the deviations of each data point from the median (47.5).
\(
\begin{align*}
36 - 47.5 & = -11.5 \\
42 - 47.5 & = -5.5 \\
45 - 47.5 & = -2.5 \\
46 - 47.5 & = -1.5 \\
46 - 47.5 & = -1.5 \\
49 - 47.5 & = 1.5 \\
51 - 47.5 & = 3.5 \\
53 - 47.5 & = 5.5 \\
60 - 47.5 & = 12.5 \\
72 - 47.5 & = 24.5 \\
\end{align*}
\)
Step 4: Calculate the absolute deviations
Now, we take the absolute values of these deviations.
\(
\begin{align*}
| -11.5 | & = 11.5 \\
| -5.5 | & = 5.5 \\
| -2.5 | & = 2.5 \\
| -1.5 | & = 1.5 \\
| -1.5 | & = 1.5 \\
| 1.5 | & = 1.5 \\
| 3.5 | & = 3.5 \\
| 5.5 | & = 5.5 \\
| 12.5 | & = 12.5 \\
| 24.5 | & = 24.5 \\
\end{align*}
\)
Step 5: Sum the absolute deviations
Now, we sum all the absolute deviations.
\(
\text{Sum} = 11.5 + 5.5 + 2.5 + 1.5 + 1.5 + 1.5 + 3.5 + 5.5 + 12.5 + 24.5 = 70
\)
Step 6: Calculate the mean deviation
Finally, we calculate the mean deviation by dividing the sum of absolute deviations by the number of observations.
\(
\text{Mean Deviation} = \frac{\text{Sum of absolute deviations}}{\text{Number of observations}} = \frac{70}{10} = 7
\)
Final Answer
The mean deviation about the median for the given data is 7.
---
First, we need to arrange the given data in ascending order.
36,42,45,46,46,49,51,53,60,72
Since there are 10 observations (an even number), the median is calculated as the average of the 5th and 6th observations.
- 6th observation = 49
\(
\text{Median} = \frac{46 + 49}{2} = \frac{95}{2} = 47.5
\)
Next, we calculate the deviations of each data point from the median (47.5).
\begin{align*}
36 - 47.5 & = -11.5 \\
42 - 47.5 & = -5.5 \\
45 - 47.5 & = -2.5 \\
46 - 47.5 & = -1.5 \\
46 - 47.5 & = -1.5 \\
49 - 47.5 & = 1.5 \\
51 - 47.5 & = 3.5 \\
53 - 47.5 & = 5.5 \\
60 - 47.5 & = 12.5 \\
72 - 47.5 & = 24.5 \\
\end{align*}
\)
Now, we take the absolute values of these deviations.
\begin{align*}
| -11.5 | & = 11.5 \\
| -5.5 | & = 5.5 \\
| -2.5 | & = 2.5 \\
| -1.5 | & = 1.5 \\
| -1.5 | & = 1.5 \\
| 1.5 | & = 1.5 \\
| 3.5 | & = 3.5 \\
| 5.5 | & = 5.5 \\
| 12.5 | & = 12.5 \\
| 24.5 | & = 24.5 \\
\end{align*}
\)
Now, we sum all the absolute deviations.
\text{Sum} = 11.5 + 5.5 + 2.5 + 1.5 + 1.5 + 1.5 + 3.5 + 5.5 + 12.5 + 24.5 = 70
\)
Finally, we calculate the mean deviation by dividing the sum of absolute deviations by the number of observations.
\text{Mean Deviation} = \frac{\text{Sum of absolute deviations}}{\text{Number of observations}} = \frac{70}{10} = 7
\)
The mean deviation about the median for the given data is 7.
Topper's Solved these Questions
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - MISCELLANEOUS EXERCISE
7 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE
20 Videos
- SETSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SETSEXERCISE - MISC Exercise
16 Videos
- STRAIGHT LINESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Exercise
207 Videos
Similar Questions
Find the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the median for the data in Question

View SolutionFind the mean deviation about median for the following data:

View SolutionFind the mean deviation about mean for the following data:
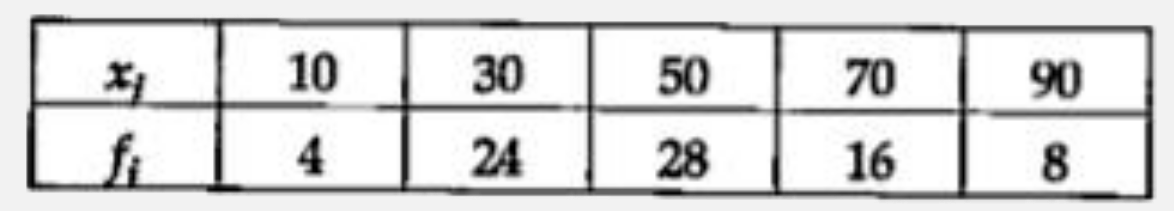
View SolutionFind the mean deviation about the mean for the following data.
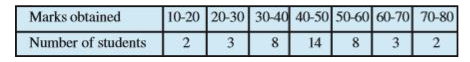
View SolutionFind the mean deviation about the median for the data : 13,17,16,14,11,13,10,16,11,18,12,17
View SolutionFind the mean deviation about the mean for the data : 4,7,8,9,10,12,13,17
View SolutionFind the mean deviation about the mean for the data is Question:
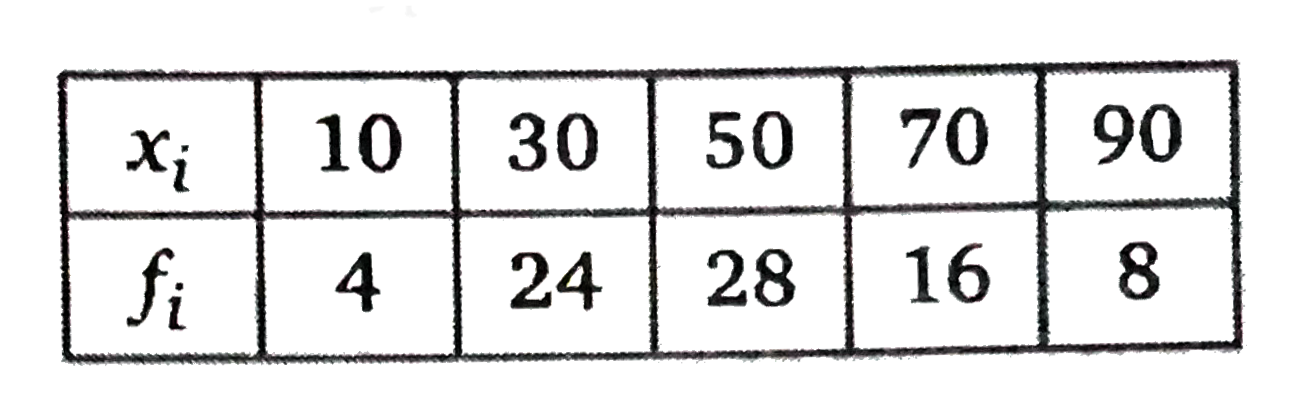
View Solution
