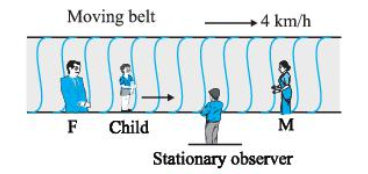
A long belt is moving horizontally with a speed of 5 km/h. A child is running on belt to and fro with a speed of 9 km/h with respect to belt between his father and mother located 50 m apart on the belt. For an observer on a stationary platform outside, what is the
speed of the child running opposite to the direction of the belt ?

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to determine the speed of the child running opposite to the direction of the belt as observed by a stationary observer. Here’s a step-by-step solution:
Step 1: Understand the given information
- The speed of the belt (V_b) = 5 km/h (moving in a horizontal direction).
- The speed of the child with respect to the belt (V_c) = 9 km/h.
- The child runs to and fro between his father and mother, who are 50 m apart on the belt.
Step 2: Determine the speed of the child running in the same direction as the belt
When the child runs in the same direction as the belt, the speeds add up. Therefore, the speed of the child as observed by a stationary observer (V_observed) is given by:
$V_{o b s e r v e d} = V_{b} + V_{c}$
Substituting the values:
$V_{o b s e r v e d} = 5 km/h + 9 km/h = 14 km/h$
Step 3: Determine the speed of the child running opposite to the direction of the belt
When the child runs opposite to the direction of the belt, the speed of the belt will subtract from the speed of the child. Thus, the speed of the child as observed by a stationary observer when running opposite to the belt is:
$V_{o b s e r v e d} = V_{c} - V_{b}$
Substituting the values:
$V_{o b s e r v e d} = 9 km/h - 5 km/h = 4 km/h$
Final Answer
The speed of the child running opposite to the direction of the belt, as observed by a stationary observer, is 4 km/h.
---

On a long horizontally moving belt, a child runs to an fro with a speed $9 k m h^{- 1}$ ( with respect to the belt) between his father and mother located 50m a part on the moving belt. The belt moves with a speed of 4 $k m h^{- 1}$ . For an observer on a stationery platform outside, what is the
(i) speed of the child running in the direction of motion of the belt,
(ii) speed of the child running opposited to the direction of motion of the belt , and (iii) time taken by child in (i) and (ii) ?
which of the answers alter if motion is viewed by one of the parents?
A $4 k m h^{- 1}, 5 k m h^{- 1}$
B $5 k m h^{- 1}, 9 k m h^{- 1}$
C $9 k m h^{- 1}, 4 k m h^{- 1}$
D $13 k m h^{- 1}, 5 k m h^{- 1}$
In the question number 97, the speed of the child running opposite to the direction of motion of the belt is
A $4 k m h^{- 1}$
B $5 k m h^{- 1}$
C $9 k m h^{- 1}$
D $13 k m h^{- 1}$