Freundlich equation for adsorption of gases (in amount of Xg) on a solid (in amount od mg) at constant temperature can be expressed as
log(xm)=logp+1nlogk
log(xm)=logk+1nlogp
(xm)∝pn
(xm)=logp+1nlogk
To derive the Freundlich equation for the adsorption of gases on a solid at constant temperature, we can follow these steps:
1. Understanding the Freundlich Isotherm:
The Freundlich isotherm describes the adsorption of gases on solids and is based on the assumption that adsorption occurs on a heterogeneous surface. It is applicable to monolayer adsorption.
2. Basic Relationship:
The Freundlich equation can be expressed in terms of the amount of gas adsorbed (X) per unit mass of the solid (m) and the pressure (P) of the gas. The relationship is given by:
Xm∝P1n
where Xm is the specific mass of the gas adsorbed, P is the pressure, and n is a constant that indicates the degree of non-linearity of the adsorption.
3. Introducing a Constant:
We can introduce a constant k such that:
Xm=k⋅P1n
4. Taking the Logarithm:
To linearize the equation, we take the logarithm of both sides:
log(Xm)=log(k⋅P1n)
5. Using Logarithmic Properties:
By applying the properties of logarithms, we can separate the terms:
log(Xm)=log(k)+log(P1n)
log(Xm)=log(k)+1nlog(P)
6. Final Form of the Freundlich Equation:
Rearranging the terms gives us the final form of the Freundlich isotherm:
log(Xm)=log(k)+1nlog(P)
Conclusion:
The Freundlich equation for adsorption can be expressed as:
log(Xm)=log(k)+1nlog(P)
This matches with option 2 from the question.
The Freundlich isotherm describes the adsorption of gases on solids and is based on the assumption that adsorption occurs on a heterogeneous surface. It is applicable to monolayer adsorption.
The Freundlich equation can be expressed in terms of the amount of gas adsorbed (X) per unit mass of the solid (m) and the pressure (P) of the gas. The relationship is given by:
Xm∝P1n
where Xm is the specific mass of the gas adsorbed, P is the pressure, and n is a constant that indicates the degree of non-linearity of the adsorption.
We can introduce a constant k such that:
Xm=k⋅P1n
To linearize the equation, we take the logarithm of both sides:
log(Xm)=log(k⋅P1n)
By applying the properties of logarithms, we can separate the terms:
log(Xm)=log(k)+log(P1n)
log(Xm)=log(k)+1nlog(P)
Rearranging the terms gives us the final form of the Freundlich isotherm:
log(Xm)=log(k)+1nlog(P)
The Freundlich equation for adsorption can be expressed as:
log(Xm)=log(k)+1nlog(P)
This matches with option 2 from the question.
Topper's Solved these Questions
- SURFACE CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - LEVEL-2
65 Videos
- SURFACE CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - JEE Main (Archive)
14 Videos
- SURFACE CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - PRACTICE EXERCISE
9 Videos
- STRUCTURE OF ATOMBOOK - VMC MODULES ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - IN-CHAPTER EXERCISE-F
7 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - EXERCISE-J
10 Videos
Similar Questions
Draw a graph of logP and log(1/V) for a fixed amount of gas at constant temperature.
View SolutionDraw a graph of log P vs log (1/V) for a fixed amount of a gas at constant temperature.
View Solution(a) In reference to Freundlich adorption isotherm write the expression for adsorption of gases on solids in the form of an equation.
(b) Write an important characteristic of lyophilic solc.
(c) Based on type of particles of dispersed phase, give one example each of associated colloid and multimolecular colloid.
View SolutionAssertion : For a certain amount of an ideal gas, PV is always constant at constant temperature.
Reason : This is the statement of Boyle's Law.
View SolutionThe amount of heat required to change the state of 1 kg of substance at constant temperature is called
View SolutionObserve the graph given below and answer the following questions:
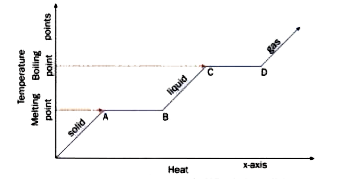
During melting of a solid, certain amount of heat is absorbed without any change in temperature. Which part of the graph shows this change?
View SolutionA definite amount of solid NH4HS is placed in a flask aleady containing ammoina gas at a certain temperature and 0.50 atm pressure. NH4HS decomposes to give NH3 and H2S and at equilibrium total pressure in flask is 0.84 atm. The equilibrium constant for the reaction is:
View SolutionA: For a certain amount of gas, PV is always constant at constant temperature.
R: On increasing temperature PV increases for fixed amount of ideal gas.
View SolutionWhat is the type of graph between log P and log(1V) at constant temperature for a given amount of gas ?
View SolutionAn amount of heat 50J of heat energy is removed from 4 moles of a monatomic ideal gas at constant volume.The temperature drops by
View Solution
