If −(dNdt)o is the initial activity and −(dNdt) is the activity at time t in a radioactive disintegration then :
−(dNdt)o=−(dNdt)e−kt
−(dNdt)o=(dNdt)oe−kt
−(dNdt)o=−(dNdt)oe−kt
−(dNdt)o=(dNdt)oe−kt
The correct Answer is:C
To solve the problem regarding the relationship between the initial activity and the activity at time t in a radioactive disintegration, we can follow these steps:
1. Understand the Reaction Order:
- Radioactive disintegration is a first-order reaction. This means that the rate of disintegration is proportional to the number of radioactive particles present.
2. Define Initial Activity:
- The initial activity, denoted as −dNdt∣∣∣0, is the rate of disintegration at time t=0. For a first-order reaction, this can be expressed as:
−dNdt∣∣∣0=k⋅N0
where k is the rate constant and N0 is the initial number of radioactive particles.
3. Define Activity at Time t:
- The activity at time t, denoted as −dNdt, can be expressed in terms of the remaining number of particles N at that time:
−dNdt=k⋅N
4. Relate Remaining Particles to Time:
- For a first-order reaction, the number of particles remaining at time t is given by the equation:
N=N0e−kt
5. Substitute N into Activity Equation:
- Substitute the expression for N into the activity equation:
−dNdt=k⋅(N0e−kt)
6. Express Activity in Terms of Initial Activity:
- Now, we can relate this to the initial activity:
−dNdt=−dNdt∣∣∣0⋅e−kt
where −dNdt∣∣∣0=k⋅N0.
7. Final Relationship:
- Thus, we arrive at the relationship between the initial activity and the activity at time t:
−dNdt=−dNdt∣∣∣0⋅e−kt
- Radioactive disintegration is a first-order reaction. This means that the rate of disintegration is proportional to the number of radioactive particles present.
- The initial activity, denoted as −dNdt∣∣∣0, is the rate of disintegration at time t=0. For a first-order reaction, this can be expressed as:
−dNdt∣∣∣0=k⋅N0
where k is the rate constant and N0 is the initial number of radioactive particles.
- The activity at time t, denoted as −dNdt, can be expressed in terms of the remaining number of particles N at that time:
−dNdt=k⋅N
- For a first-order reaction, the number of particles remaining at time t is given by the equation:
N=N0e−kt
- Substitute the expression for N into the activity equation:
−dNdt=k⋅(N0e−kt)
- Now, we can relate this to the initial activity:
−dNdt=−dNdt∣∣∣0⋅e−kt
where −dNdt∣∣∣0=k⋅N0.
- Thus, we arrive at the relationship between the initial activity and the activity at time t:
−dNdt=−dNdt∣∣∣0⋅e−kt
Topper's Solved these Questions
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - Level-2 ( Numerical Value Type for JEE Main )
15 Videos
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - JEE Main (Archive)
56 Videos
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - Level-1
75 Videos
- CHEMICAL EQUILIBRIUMBOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - IN-CHAPTER EXERCISE - G
10 Videos
- CHEMICAL THERMODYNAMICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - IN - CHAPTER EXERCISE - L
10 Videos
Similar Questions
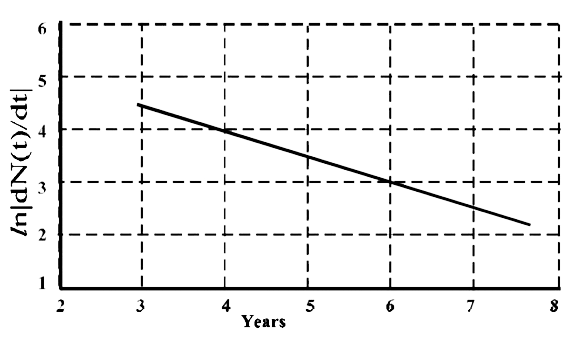
To determine the half life of a radioactive element , a student plot a graph of in ∣∣∣dN(t)dt∣∣∣ versus t , Here ∣∣∣dN(t)dt∣∣∣ is the rate of radiation decay at time t , if the number of radioactive nuclei of this element decreases by a factor of p after 4.16year the value of p is
View SolutionWhich has maximum radioactive activity :
View SolutionTo determine the half life of a radioactive element , a student plote a graph of in ∣∣∣dN(t)dt∣∣∣versust,Here∣∣∣dN(t)dt∣∣∣ is the rate of radioatuion decay at time t , if the number of radoactive nuclei of this element decreases by a factor of p after 4.16 year the value of p is

View Solution∫etsintdt
View SolutionFor a radioactive material, its activity A and rate of change of its activity of R are defined as A=−dNdt and R=−dAdt, where N(t) is the number of nuclei at time t. Two radioactive source P (mean life τ) and Q (mean life 2τ) have the same activity at t=0. Their rates of activities at t=2τ are Rp and RQ, respectively. If RPRQ=ne, then the value of n is:
View SolutionAt a given, t = 0, two radioactive substances A and B have equal activities. The ratio RBRA of their activities after time t itself decays with time t as e−3t, If the half-life of A is ln2, the half-life of B is :
View SolutionDefine the activity of a radio nuclide. Write its S.I. unit. Give a plot of the activity of a radioactive species versus time.
How long will a radioactive isotope, whose half life is T years, take for its activity to reduce to 1/8th of its initial value ?
View SolutiondNdt=rN
How does the increase and decrease in the value of 'r' affect the population size.
View SolutionRadioactive disintegration is a first order reaction and its rate depends only upon the nature of nucleus and does not depend upon external factors like temperature and pressure. The rate of radioactive disintegration (Activity) is represented as
−dNdt=λN Where λ= decay constant, N= number of nuclei at time t, N0 =intial no. of nuclei. The above equation after integration can be represented as
λ=2.303tlog(N0N)
Calculate the half-life period of a radioactive element which remains only 1/16 of its original amount in 4740 years:
View SolutionRadioactive disintegration is a first order reaction and its rate depends only upon the nature of nucleus and does not depend upon external factors like temperature and pressure. The rate of radioactive disintegration (Activity) is represented as
−dNdt=λN Where λ= decay constant, N= number of nuclei at time t, N0 =intial no. of nuclei. The above equation after integration can be represented as
λ=2.303tlog(N0N)
Half-life period of U2.5×105 years. In how much thime will the amount of U237 remaining be only 25% of the original amount ?
View Solution
