Consider the charge configuration and spherical Gaussian surface as shown in the figure. When calculating the flux of the electric field over the spherical surface the electric field will be due to
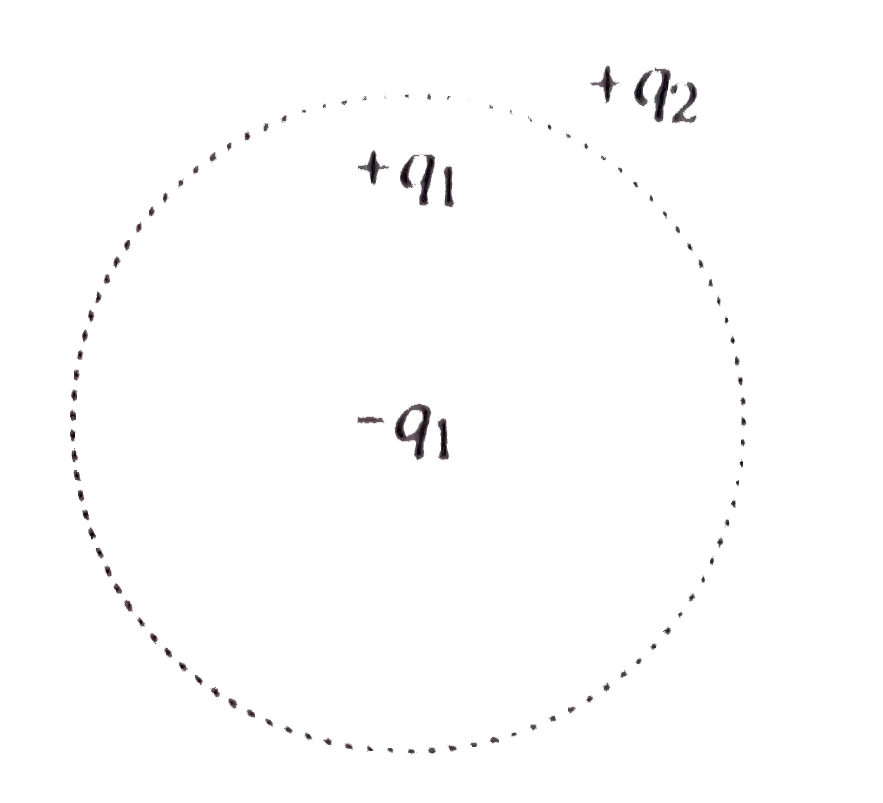
q2
only the positive charges
all the charges
+q1 and q2
The correct Answer is:C
At any point over the spherical Gaussian surface, net electric field is the vector sum of electric fields due to +q1,−q1andq2. Don’t confuse with the electric flux which is zero (net) passing over the Gaussian surface as the net charge enclosing the surface is zero. Hence, the correct option is (C).
Topper's Solved these Questions
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE MAIN
65 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE
52 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
1 Videos
Similar Questions
Consider the charge configuration and a spherical Gaussian surface as shown in the figure. When calculating the flux of the electric field over the spherical surface, the electric field will be due to.
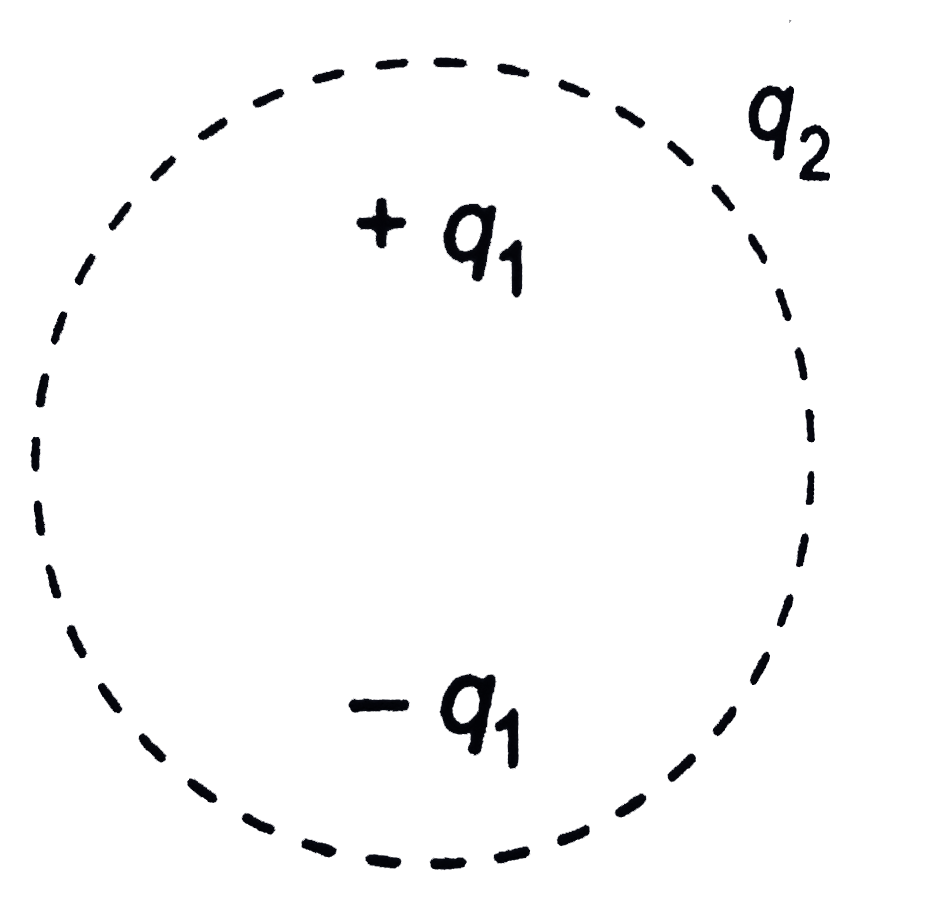
View SolutionConsider the charge configuration and a spherical Gaussian surface as shown in the figure. When calculating the flux of the electric field over the spherical surface, the electric field will be due to.

View SolutionIf the flux of the electric field through a closed surface is zero,
View SolutionElectric flux through a spherical surface shown in the figure is
 _______
_______
View SolutionA Gausisan surface in the figure is shown by dotted line.
The electric field on the surface will be
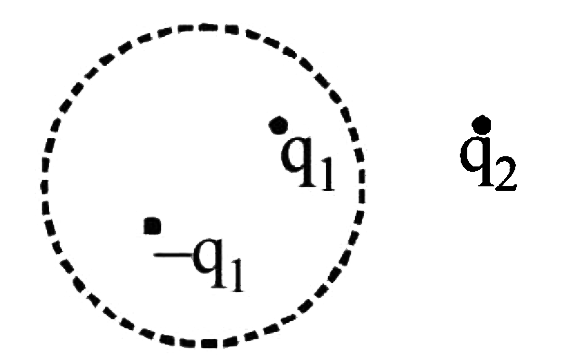
View SolutionA charge Q is placed at the centre of a cube. Find the flux of the electric field through the six surfaced of the cube.
View SolutionFind the flux due to the electric field through the curved surface (R is radius of curvature)

View SolutionThe Gaussian surface for calculating the electric field due to a charge distribution is
View SolutionThe electric field inside a spherical shell of uniform surface charge density is
View SolutionA small metal sphere carrying charge +Q is located at the centre of a spherical cavity inside a large uncharged metallic spherical shell as shown in the figure. Use Gauss’s law to find the expressions for the electric field at points p1 and p2 . Also draw the pattern of electric field lines in this arrangement.

View Solution
