Two point charges +4q and +q are placed 30cm apart. At what point on the line joining them is the electric field zero
15 cm from charge q
20 cm from charge 4 q
7.5 cm from charge q
5cm from charge q
The correct Answer is:B
To find the point where the electric field is zero between two point charges +4q and +q separated by a distance of 30 cm, we can follow these steps:
1. Identify the Charges and Their Positions:
- Let the charge +4q be at point A (0 cm) and charge +q be at point B (30 cm).
- The distance between the charges is given as 30 cm.
2. Set Up the Problem:
- We need to find a point P on the line joining the two charges where the electric field is zero.
- Let the distance from charge +4q to point P be x cm. Therefore, the distance from charge +q to point P will be (30 - x) cm.
3. Understand Electric Field Direction:
- The electric field due to a positive charge is directed away from the charge.
- At point P, the electric field due to charge +q will be directed away from +q (to the left) and the electric field due to charge +4q will be directed away from +4q (to the right).
4. Write the Expression for Electric Fields:
- The electric field Eq due to charge +q at point P is given by:
Eq=k⋅q(30−x)2
- The electric field E4q due to charge +4q at point P is given by:
E4q=k⋅4qx2
5. Set the Electric Fields Equal:
- For the electric field to be zero at point P, the magnitudes of the electric fields due to both charges must be equal:
Eq=E4q
- Therefore, we can write:
k⋅q(30−x)2=k⋅4qx2
6. Cancel Common Terms:
- We can cancel k and q from both sides (assuming q≠0):
1(30−x)2=4x2
7. Cross Multiply:
- Cross multiplying gives:
x2=4(30−x)2
8. Expand and Rearrange:
- Expanding the right side:
x2=4(900−60x+x2)
x2=3600−240x+4x2
- Rearranging gives:
0=3x2−240x+3600
9. Solve the Quadratic Equation:
- Dividing the entire equation by 3:
x2−80x+1200=0
- Using the quadratic formula x=−b±√b2−4ac2a:
x=80±√6400−48002
x=80±√16002
x=80±402
- This gives two solutions:
x=60 cm (not valid, outside the range)andx=20 cm
10. Conclusion:
- The point where the electric field is zero is 20 cm from charge +4q.
Final Answer:
The electric field is zero at a point 20 cm from charge +4q.
- Let the charge +4q be at point A (0 cm) and charge +q be at point B (30 cm).
- The distance between the charges is given as 30 cm.
- We need to find a point P on the line joining the two charges where the electric field is zero.
- Let the distance from charge +4q to point P be x cm. Therefore, the distance from charge +q to point P will be (30 - x) cm.
- The electric field due to a positive charge is directed away from the charge.
- At point P, the electric field due to charge +q will be directed away from +q (to the left) and the electric field due to charge +4q will be directed away from +4q (to the right).
- The electric field Eq due to charge +q at point P is given by:
Eq=k⋅q(30−x)2
- The electric field E4q due to charge +4q at point P is given by:
E4q=k⋅4qx2
- For the electric field to be zero at point P, the magnitudes of the electric fields due to both charges must be equal:
Eq=E4q
- Therefore, we can write:
k⋅q(30−x)2=k⋅4qx2
- We can cancel k and q from both sides (assuming q≠0):
1(30−x)2=4x2
- Cross multiplying gives:
x2=4(30−x)2
- Expanding the right side:
x2=4(900−60x+x2)
x2=3600−240x+4x2
- Rearranging gives:
0=3x2−240x+3600
- Dividing the entire equation by 3:
x2−80x+1200=0
- Using the quadratic formula x=−b±√b2−4ac2a:
x=80±√6400−48002
x=80±√16002
x=80±402
- This gives two solutions:
x=60 cm (not valid, outside the range)andx=20 cm
- The point where the electric field is zero is 20 cm from charge +4q.
The electric field is zero at a point 20 cm from charge +4q.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - LEVEL - 2
60 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE MAIN
65 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - LEVEL - 0 (LONG ANSWER TYPE )
8 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE
52 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
1 Video
Similar Questions
Two point charges 4Q, Q are separated by 1m in air. At what point on the line joining the charges is the electric field intensity zero ?
Also calculate the electrostatic potential energy of the system of charges, taking the value of charge, Q=2×10−7C.
View SolutionTwo point charge - 5μC and + 3μC are placed 64 cm apart. At what points on the line joining the two charges is the electric potential zero. Assume the potential at infinity to be zero.
View SolutionTwo charges 4q and q are placed 30 cm. apart. At what point the value of electric field will be zero
View SolutionTwo charges 3×10−8Cand−2×10−8C are located 15 cm apart. At what point on the line joining the two charges is the electric potential zero ? Take the potential at infinity to be zero.
View SolutionTwo charges 5×10−8Cand−3×10−8C are located 16 cm apart. At what points on the line joining the two charges is the electric potential zero ? Take the potential at infinity to be zero.
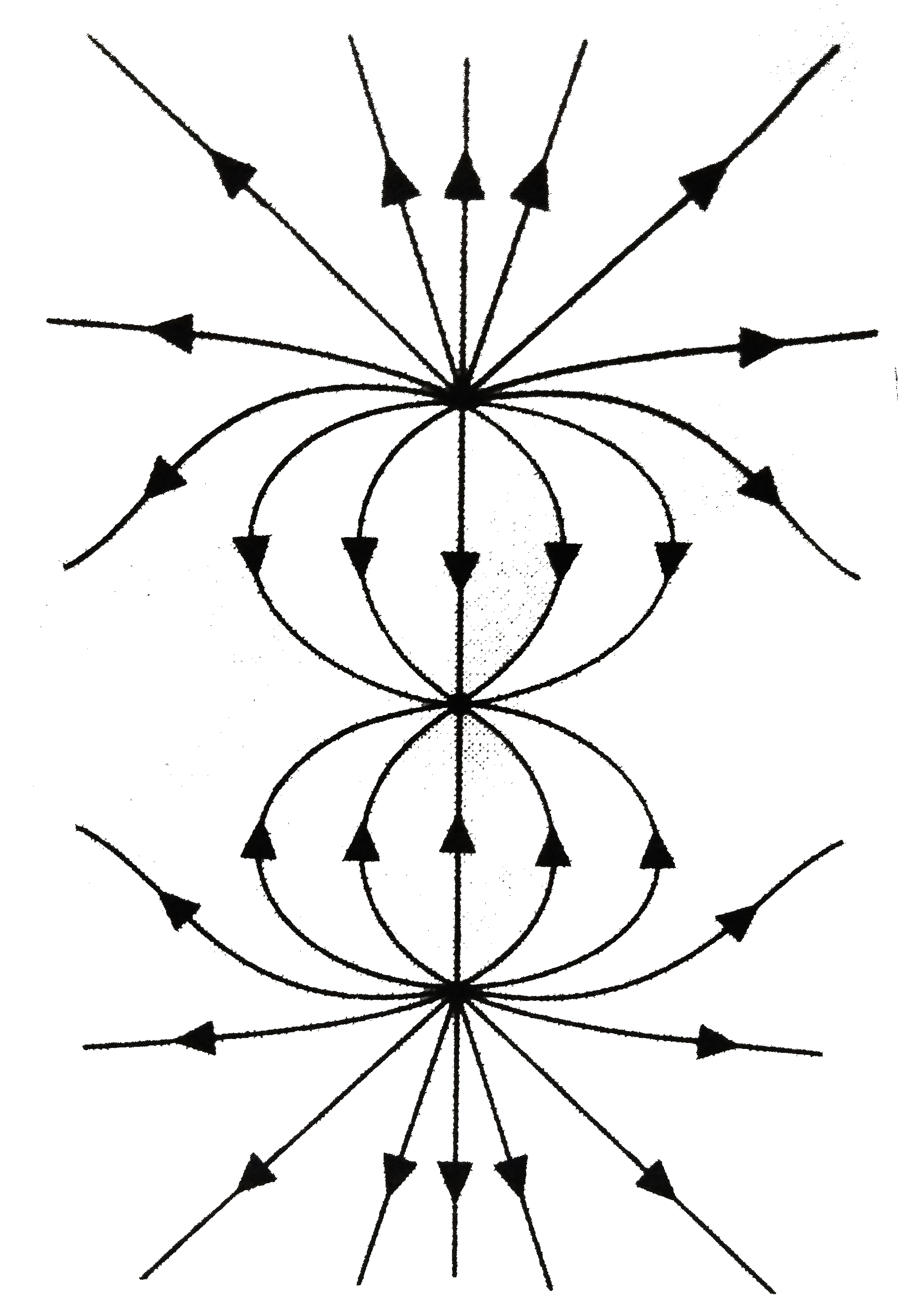
View SolutionFig. shows some of the electric field lines due to three point charges arranged along the vertical axis. All three charges have the same magnitude.
(a) What are the signs of each of the three charges? Explain your reasoning.
(b) At what points(s) is the magnitude of the electric field the smallest? Explain your reasoning. Explain how the fields produced by each individual point charge combine to give a small net field at this point or points.
(c ) Two point charges q and -q are placed at a distance d apart. What are the points at which the resultant electric field is parallel to the line joining the two charge?
 .
.
View SolutionTwo electric charges 12μC and −6μC are placed 20cm apart in air. There will be a point P on the line joining these charges and outside the region between them, at which the electric potential is zero. The distance of P from 6μC charge is
View SolutionTwo point masses of mass 10 kg and 1000 kg are at a distance 1 m apart. At which points on the line joining them, will the gravitational field intensity be zero ?
View SolutionTwo charge +aq and -q are kept apart. Then , at any point on the right bisector of line joining the two charge
View SolutionTwo point charges 4μCand−2μC are separated by a distance of 1m in air. Calculate at what point on the line joining the two charges is the electric potential zero ?
View Solution
