A circular loop of area 0.01m2 carrying a current of 10A, is held perpendicular to a magnetic field of intensity 0.1T. The torque acting on the loop is
(a)0.01 Nm
(b)0.001 Nm
(c)zero
(d)0.8 Nm
The correct Answer is:C
To solve the problem of finding the torque acting on a circular loop in a magnetic field, we can follow these steps:
1. Identify the given values:
- Area of the loop, A=0.01m2
- Current flowing through the loop, I=10A
- Magnetic field intensity, B=0.1T
2. Calculate the magnetic moment (μ) of the loop:
The magnetic moment (μ) for a current-carrying loop is given by the formula:
μ=I×A
Substituting the values:
μ=10A×0.01m2=0.1A⋅m2
3. Determine the angle (θ) between the magnetic moment and the magnetic field:
Since the loop is held perpendicular to the magnetic field, the angle θ is 90∘.
4. Use the formula for torque (τ):
The torque (τ) acting on the loop in a magnetic field is given by:
τ=μ×B×sin(θ)
Substituting the values we have:
τ=0.1A⋅m2×0.1T×sin(90∘)
5. Calculate sin(90∘):
We know that sin(90∘)=1.
6. Calculate the torque:
τ=0.1A⋅m2×0.1T×1=0.01N⋅m
Final Answer:
The torque acting on the loop is 0.01N⋅m.
---
- Area of the loop, A=0.01m2
- Current flowing through the loop, I=10A
- Magnetic field intensity, B=0.1T
The magnetic moment (μ) for a current-carrying loop is given by the formula:
μ=I×A
Substituting the values:
μ=10A×0.01m2=0.1A⋅m2
Since the loop is held perpendicular to the magnetic field, the angle θ is 90∘.
The torque (τ) acting on the loop in a magnetic field is given by:
τ=μ×B×sin(θ)
Substituting the values we have:
τ=0.1A⋅m2×0.1T×sin(90∘)
We know that sin(90∘)=1.
τ=0.1A⋅m2×0.1T×1=0.01N⋅m
The torque acting on the loop is 0.01N⋅m.
Topper's Solved these Questions
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - LEVEL 2
50 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Main (Archive)
75 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - LEVEL 0 (LONG ANSWER TYPE )
3 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
- MOCK TEST 1BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 1EXERCISE - PART I : PHYSICS (SECTION-2)
10 Videos
Similar Questions
A circular loop of area 1cm2 , carrying a current of 10 A , is placed in a magnetic field of 0.1 T perpendicular to the plane of the loop. The torque on the loop due to the magnetic field is
View SolutionA 50 turn circular coil of radius 2.0 cm carrying a current of 5.0 A is rotated in a magnetic field of strength 0.20 T. (a) What is the maximum torque that acts on the coil? (b) in a particular position of the coil, the torque acting on it is half of this maximum. What is the angle between the magnetic field and the plane of the coil?
View SolutionA rectangular loop of area 5m2, has 50 turns and carries a current of 1A. It is held in a uniform magnetic field of 0.1T, at an angle of 30∘. Calculate the torque experienced by the coil.
View SolutionA rectangular loop of sides 20 cm and 10 cm carries a current of 5.0 A. A uniform magnetic field of magnituded 0.20 T exists parallel ot the longer side of the loop (a) What is the force acting on the loop? (b) what is the torque acting on the loop?
View SolutionA square loop of sides 10cm carries a current of 10A.A uniform magnetic field of magnitude 0.20T exists parallel to one of the side of the loop.(a)What is the force acting on the loop? (b)What is the torque acting on the loop?
View SolutionA triangular loop of side l carries a current l. It is placed in a magnetic field B such that the plane of the loop is in the direction of B. The torque on the loop is
View SolutionA triangular loop of side l carries a current I. It is placed in a magnetic field B such that the plane of the loop is in the direction of B. The torque on the loop is
View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View SolutionGiven figure shows a coil bent with all edges of length 1m and carrying a current of 1A. There exists in space a uniform magnetic field of 2T in positive y-direction. Find the torque on the loop.
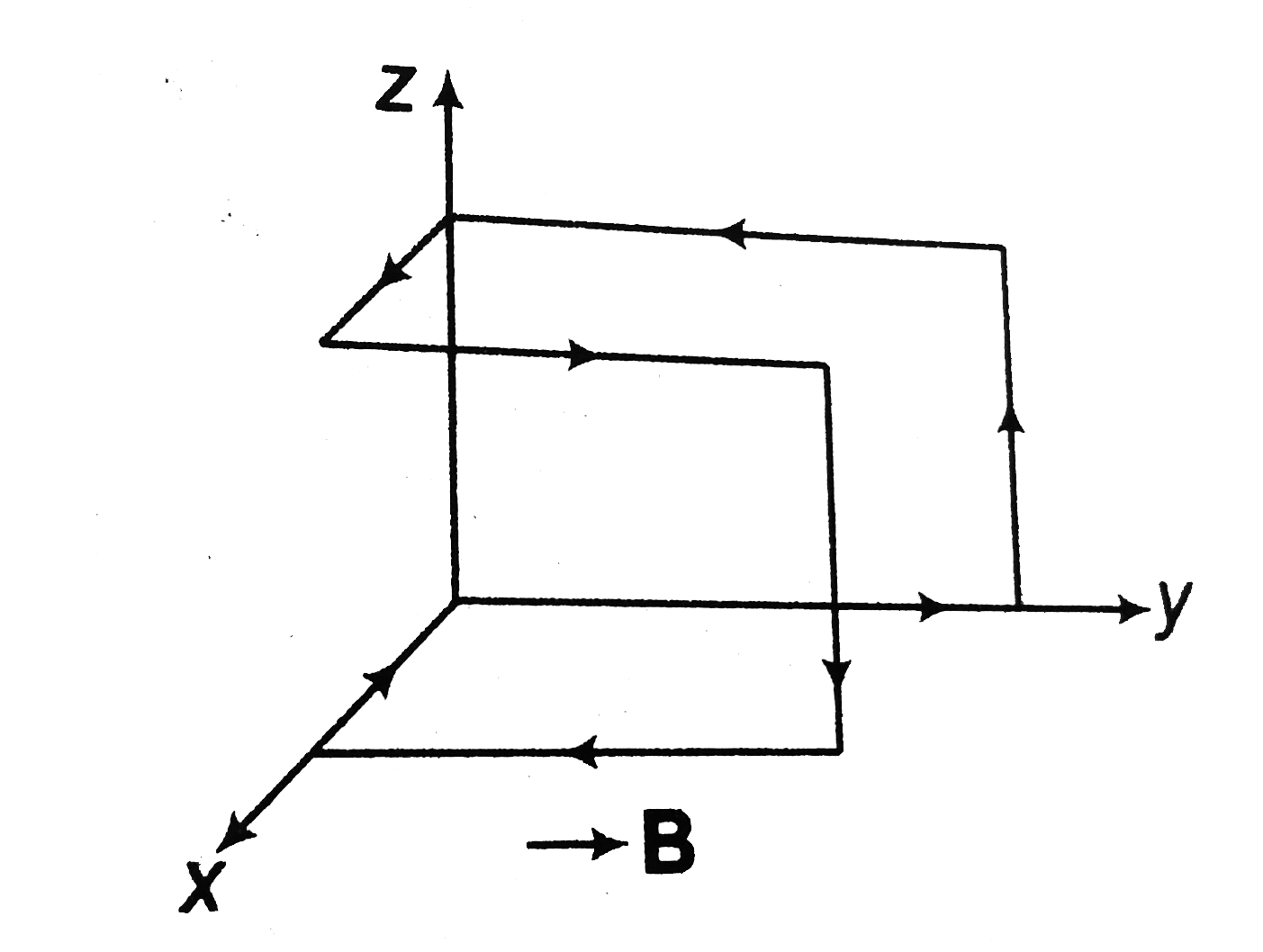
View SolutionA circular loop has a radius of 5cm and it is carrying a current of 0.1amp. It magneitc moment is
View Solution

