A tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then

Video Solution

Text Solution

Verified by Experts

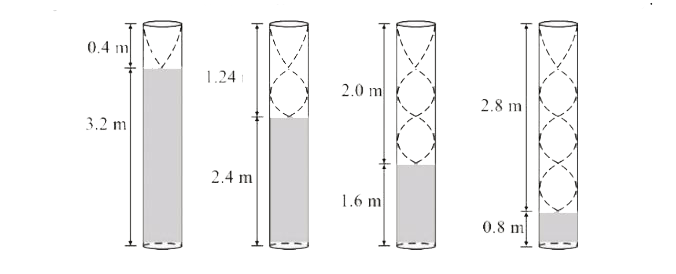
(i) 3.2m, 2.4m, 1.6m and 0.8m (ii) $\frac{- d h}{d t} = (1.11 \times 10^{- 2}) \sqrt{H}$ (b) 43 sec
Let $1_{0}$ be the length of air column corresponding to thr fundamental frequency
$1_{0} = \frac{λ}{4}$ or $λ = 41_{0}$
Also $v = f λ$ , Hence $f = \frac{v}{λ}$
Then $\frac{v}{41_{0}} = 212.5 or$ 1_0=(v)/(4(212.5))=(340)/(4(212.5))=0.4m
In closed pipe only odd harmonic are Now let $1_{1}, 1_{2}, 1_{3}, 1_{4},$ etc. be the length corresponding to the $3^{r d}$ harmonic
$3^{r d} h a r m o n i c, 3 (\frac{v}{41_{1}}) = 212.5 \Rightarrow 1_{1} = 1.2 m, 5^{t h} h a r m o n i c, 5 (\frac{v}{41_{2}}) = 212.5 \Rightarrow 1_{2} = 2.0 m$
$7^{t h}, h a r m o n i c 7 (\frac{v}{41_{3}}) = 212.5 \Rightarrow 1_{3} = 2.8 m, 9^{t h} h a r m o n i c, 9 (\frac{v}{41_{4}}) = 212.5 \Rightarrow 1_{4} = 3.6 m$
or heights of water level are (3.6-0.4)m,(3.6-1.2)m, (3.6-2.0)m, and (3.6-2.6)m
Therefore,heights of water level are 3.2m,2.4m,1.6m and 0.8m from bottom. Let A and a be the area of cross-sections of the pipe and hole respectively.
Then $A = π {(2 \times 10^{- 2})}^{2} = 1.26 \times 10^{- 3} m^{2} and a = π {(10^{- 3})}^{2} = 3.14 \times 10^{- 6} m^{2}$
Velocity of efflux $a \sqrt{2 g H}$
Continuity equation at top of the tube and onifice $a \sqrt{2 g H} = A (\frac{- d H}{d t})$
Therefore rate of fall of water level in the pipe . $(\frac{- d H}{d t}) = \frac{a}{A} \sqrt{2 g H}$
Subsituting the values,we get $\frac{- d H}{d t} = \frac{314 \times 10^{- 6}}{1.26 \times 10^{- 3}} \sqrt{2 \times 10 \times H} \Rightarrow \frac{- d H}{d t} = (1.11 \times 10^{- 2}) \sqrt{H}$
Between first two resonance,the water level falls from 3.2m to 2.4m
$∴ \frac{d H}{\sqrt{H}} = - 11 \times 10^{- 2} d t \Rightarrow \int_{3.2}^{2.4} \frac{d H}{\sqrt{H}} = - (1.11 \times 10^{- 2}) \int_{0}^{t} d t$
$\Rightarrow [\sqrt{2.4} - \sqrt{3.2}] = - (1.11 \times 10^{- 2}) t \Rightarrow t = 43 sec$

Updated on:21/07/2023

A large open tank has two holes in the wall. One is square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth of 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then R is equal to
A $L / \sqrt{(2 π)}$
B $2 π L$
CL
D $L / 2 π$