A Carnot engine operating between temperature T1andT2 has efficiency 1/6. When T2 is lowered by 62K its efficiency increase to 1/3. Then T1andT2 are, respectively:
372 K and 310 K
372 K and 330 K
330 K and 268 K
310 K and 248 K
The correct Answer is:A
To solve the problem, we will use the efficiency formula of a Carnot engine and set up equations based on the given conditions.
Step 1: Write the efficiency equation for the first case
The efficiency (η) of a Carnot engine is given by:
η=1−T2T1
For the first case, we know the efficiency is 16:
16=1−T2T1
Rearranging this, we get:
T2T1=1−16=56
This gives us our first equation:
T2=56T1(Equation 1)
Step 2: Write the efficiency equation for the second case
In the second case, T2 is lowered by 62 K, and the new efficiency becomes 13:
13=1−T2−62T1
Rearranging this, we have:
T2−62T1=1−13=23
This gives us our second equation:
T2−62=23T1(Equation 2)
Step 3: Substitute Equation 1 into Equation 2
Now we can substitute T2 from Equation 1 into Equation 2:
56T1−62=23T1
To eliminate the fractions, we can multiply the entire equation by 6:
5T1−372=4T1
Step 4: Solve for T1
Rearranging gives:
5T1−4T1=372
T1=372K
Step 5: Find T2
Now we can find T2 using Equation 1:
T2=56T1=56×372=310K
Final Answer
Thus, the temperatures T1 and T2 are:
T1=372K,T2=310K
The efficiency (η) of a Carnot engine is given by:
η=1−T2T1
For the first case, we know the efficiency is 16:
16=1−T2T1
Rearranging this, we get:
T2T1=1−16=56
This gives us our first equation:
T2=56T1(Equation 1)
In the second case, T2 is lowered by 62 K, and the new efficiency becomes 13:
13=1−T2−62T1
Rearranging this, we have:
T2−62T1=1−13=23
This gives us our second equation:
T2−62=23T1(Equation 2)
Now we can substitute T2 from Equation 1 into Equation 2:
56T1−62=23T1
To eliminate the fractions, we can multiply the entire equation by 6:
5T1−372=4T1
Rearranging gives:
5T1−4T1=372
T1=372K
Now we can find T2 using Equation 1:
T2=56T1=56×372=310K
Thus, the temperatures T1 and T2 are:
T1=372K,T2=310K
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE ADVANCED (ARCHIVE )
Explore 111 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:Level - 2
Explore 40 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
Explore 1 Video
- GRAVITATIONBook:VMC MODULES ENGLISHChapter:GRAVITATIONExercise:JEE Advance (Archive) TRUE/FALSE
Explore 1 Video
Similar Questions
If the temperature of the sink of a Carnot engine having an efficiency 16 is reduced by 62∘C, then its efficiency is doubled. Find the temperature of the sink and source respectively.
View SolutionA cannot engine operates between two reservoirs of temperature T1 and T2 .The efficiency of engine is 25 %. The engine performs 100 J of work percycle. The heat energy (in J) delivered by the engine to the low temperature reservoir in a cycle is _______
View SolutionA cannot engine operates between two reservoirs of temperature T1 and T2 .The efficiency of engine is 25 %. The engine performs 100 J of work percycle. The heat energy (in J) delivered by the engine to the low temperature reservoir in a cycle is _______
View SolutionA Carnot engine has an efficiency of 1/6. When the temperature of the sink is reduced by 62∘C, its efficiency is doubled. The temperature of the source and the sink are, respectively.
View SolutionThe efficiency of a heat engine is defined as the ratio of the mechanical work done by the engine in one cycle to the heat absorbed from the high temperature source . η=WQ1=Q1−Q2Q1 Cornot devised an ideal engine which is based on a reversible cycle of four operations in succession: isothermal expansion , adiabatic expansion. isothermal compression and adiabatic compression.
_E01_236_Q01.png)
For carnot cycle Q1T1=Q2T2. Thus η=Q1−Q2Q1=T1−T2T1 According to carnot theorem "No irreversible engine can have efficiency greater than carnot reversible engine working between same hot and cold reservoirs"._E01_236_Q02.png)
A carnot engine whose low temperature reservoir is at 7∘C has an efficiency of 50%. It is desired to increase the efficiency to 70%. By how many degrees should the temperature of the high temperature reservoir be increased?
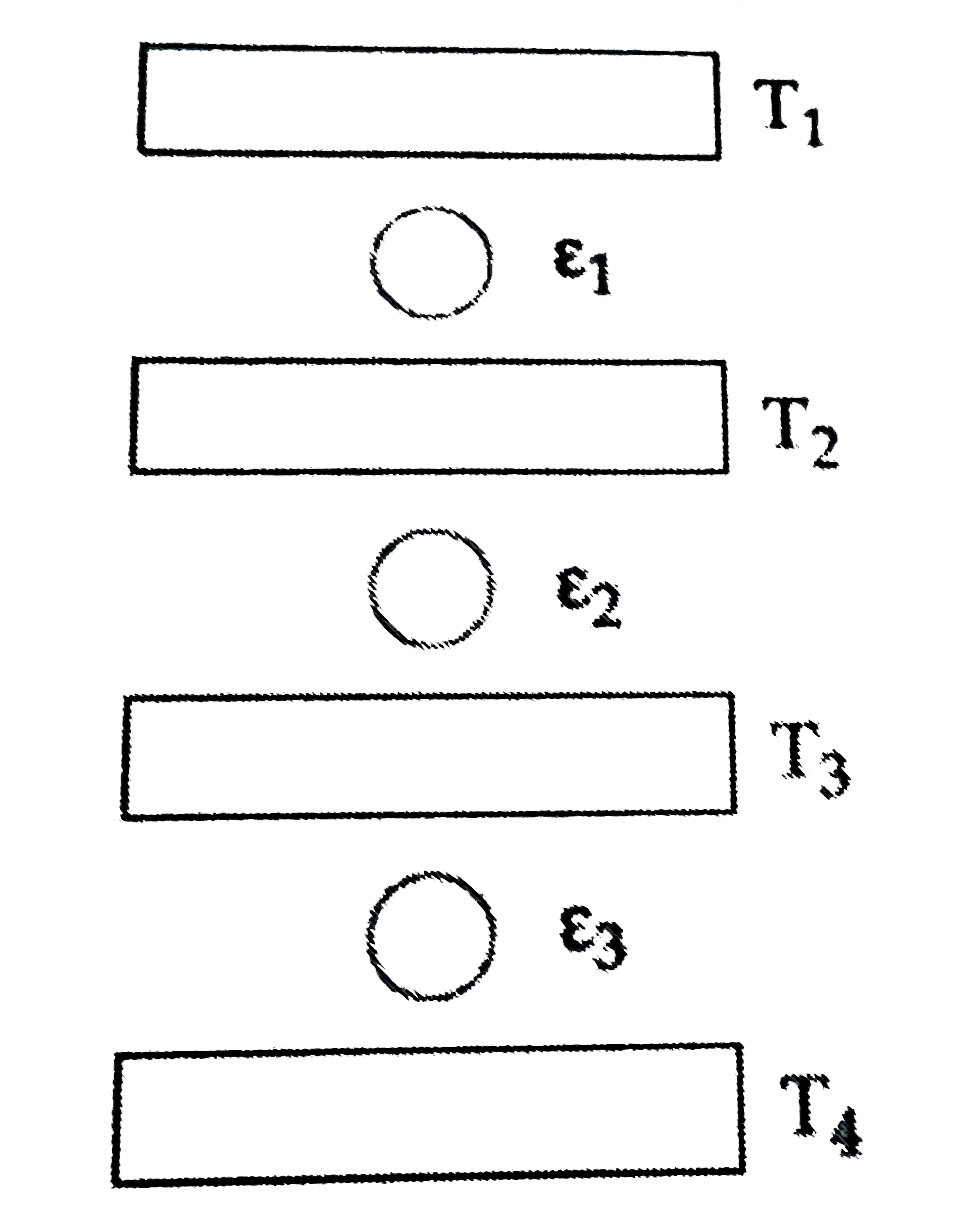
View SolutionThree Carnot engines operate in series between a heat source at a temperature T1 and a heat sink at temperature T4 (see figure). There are two other reservoirs at temperature T2andT3, as shown, with T1>T2>T3>T4. The three engines are equally efficient if :
View SolutionAn engine has an efficiency of 16. When the temperature of sink is reduced by 62∘C, its efficiency is doubled. Temperature of the source is
View SolutionAn engine has an efficiency of 16. When the temperature of sink is reduced by 62∘C, its efficiency id doubled. Temperature of the source is
View SolutionA carnot engine has an efficiency of 1/6 .On reducing the sink temperature by 65 C ,the efficiency becomes 1/2 .the source temperature is given by ?
View SolutionTwo ideal Carnot engines operate in cascade (all heat given up by one engine is used by the other engine to produce work) between temperatures, T1andT2. The temperature of the hot reservoir of the first engine is T1 and the temperature of the cold reservoir of the second engine is T2. T is temperature of the sink of first engine which is also the source for the second engine. How is T related to T1andT2, if both the engines perform equal amount of work?
View Solution