The velocity of a body of mass 2kg as a function of t is given by υ(t)=2tˆi+t2ˆj Find the momentum and force acting on it at time t=2s.
To solve the problem step by step, we will calculate the momentum and force acting on the body at time t=2 seconds.
Step 1: Identify the given data
- Mass of the body, m=2kg
- Velocity as a function of time, υ(t)=2t^i+t2^j
Step 2: Calculate the velocity at t=2 seconds
Substituting t=2 into the velocity function:
υ(2)=2(2)^i+(2)2^j=4^i+4^j
Step 3: Calculate the momentum
Momentum p is given by the formula:
p=m⋅υ
Substituting the values:
p=2kg⋅(4^i+4^j)=8^i+8^jkg m/s
Step 4: Calculate the magnitude of the momentum
The magnitude of the momentum can be calculated using the formula:
|p|=√(8)2+(8)2=√64+64=√128=8√2kg m/s
Step 5: Calculate the force
Force F can be calculated using the formula:
F=dpdt=m⋅dυdt
First, we need to find the derivative of the velocity function:
dυdt=ddt(2t^i+t2^j)=2^i+2t^j
Now substituting t=2:
dυdt=2^i+2(2)^j=2^i+4^j
Now substituting back into the force equation:
F=2kg⋅(2^i+4^j)=4^i+8^jN
Step 6: Calculate the magnitude of the force
The magnitude of the force can be calculated as:
|F|=√(4)2+(8)2=√16+64=√80=4√5N
Final Results
- Momentum at t=2s: 8^i+8^jkg m/s or 8√2kg m/s
- Force at t=2s: 4^i+8^jN or 4√5N
- Mass of the body, m=2kg
- Velocity as a function of time, υ(t)=2t^i+t2^j
Substituting t=2 into the velocity function:
υ(2)=2(2)^i+(2)2^j=4^i+4^j
Momentum p is given by the formula:
p=m⋅υ
Substituting the values:
p=2kg⋅(4^i+4^j)=8^i+8^jkg m/s
The magnitude of the momentum can be calculated using the formula:
|p|=√(8)2+(8)2=√64+64=√128=8√2kg m/s
Force F can be calculated using the formula:
F=dpdt=m⋅dυdt
First, we need to find the derivative of the velocity function:
dυdt=ddt(2t^i+t2^j)=2^i+2t^j
Now substituting t=2:
dυdt=2^i+2(2)^j=2^i+4^j
F=2kg⋅(2^i+4^j)=4^i+8^jN
The magnitude of the force can be calculated as:
|F|=√(4)2+(8)2=√16+64=√80=4√5N
- Momentum at t=2s: 8^i+8^jkg m/s or 8√2kg m/s
- Force at t=2s: 4^i+8^jN or 4√5N
Topper's Solved these Questions
- DYNAMICS OF A PARTICLEBOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 0 (LONG ANSWER TYPE)
4 Videos
- DYNAMICS OF A PARTICLEBOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 1
75 Videos
- DYNAMICS OF A PARTICLEBOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 0 (SHORT ANSWER TYPE - I)
7 Videos
- DC CIRCUITBOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - JEE ADVANCED ARCHIVE
68 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G
10 Videos
Similar Questions
Knowledge Check
The position time graph of a body of mass 2kg is as given in What is the impulse on the body at t=0 s and t=4s ?
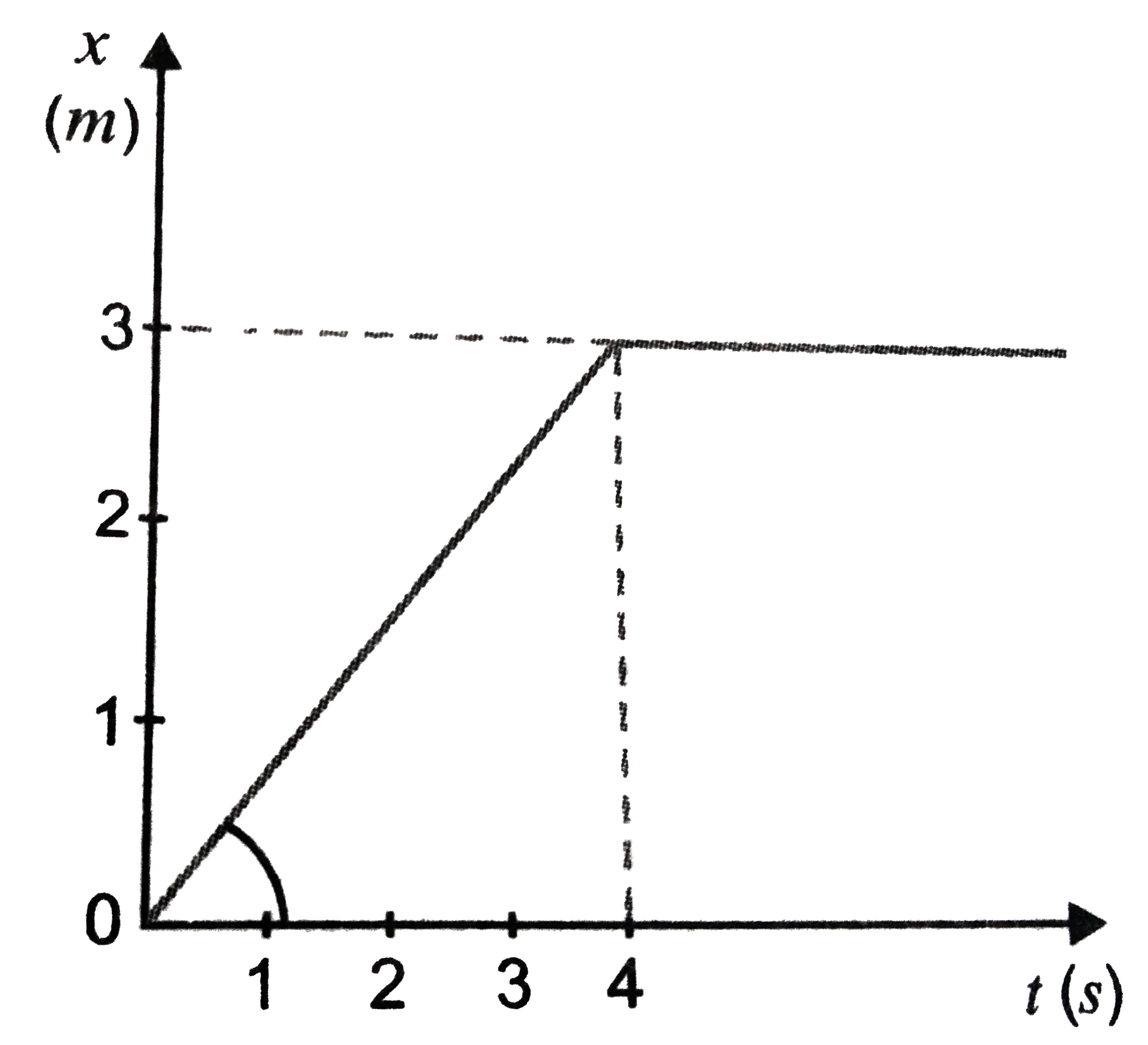 . A23kgms−1B−23kgms−1C32kgms−1D−32kgms−1
. A23kgms−1B−23kgms−1C32kgms−1D−32kgms−1
The position (x) of a particle of mass 1 kg moving along x-axis at time t is given by (x=12t2) metre. Find the work done by force acting on it in time interval from t=0 to t=3 s.
View SolutionThe position (x) of a particle of mass 1 kg moving along x-axis at time t is given by (x=12t2) meter. Find the work done by force acting on it in time interval from t=0 to t=3 s.
View SolutionThe position (x) of a particle of mass 2 kg moving along x-axis at time t is given by x=(2t3) metre. Find the work done by force acting on it in time interval t=0 to t=2 is :-
View SolutionThe time dependence of the position of a particle of mass m = 2 is given by →r(t)=2tˆi−3t2ˆj Its angular momentum, with respect to the origin, at time t = 2 is :
View SolutionThe time dependence of the position of a particle of mass m = 2 is given by →r(t)=2tˆi−3t2ˆj Its angular momentum, with respect to the origin, at time t = 2 is :
View SolutionThe velocity vector of the motion described by the position vector of a particle, r=2tˆi+t2ˆj is given by
View SolutionThe momentum of a body in two perpendicular direction at any time 't' are given by Px=2t2+6 and Py=3t22+3. The force acting on the body at t= 2 sec is
View Solution
