A particle moves in a circular path such that its speed v varies with distance s as v=α√s where α is a positive constant. If the acceleration of the particle after traversing a distance s is [α2√x+s2R2] find x.
The correct Answer is:32
To solve the problem step by step, we need to analyze the motion of the particle moving in a circular path with a varying speed. The speed v is given by the equation v=α√s, where α is a positive constant. We also know the expression for the acceleration of the particle after traversing a distance s is given as a=α2√x+s2R2. Our goal is to find the value of x.
Step 1: Understanding the Components of Acceleration
The total acceleration a of a particle moving in a circular path can be expressed as the vector sum of tangential acceleration at and radial (centripetal) acceleration ar:
a=√a2t+a2r
Step 2: Finding Tangential Acceleration
The tangential acceleration at can be defined as the rate of change of velocity with respect to time:
at=dvdt
Given v=α√s, we differentiate v with respect to t:
dvdt=ddt(α√s)=α⋅12√s⋅dsdt
Since dsdt=v=α√s, we substitute this into the equation:
dvdt=α⋅12√s⋅(α√s)=α22
Step 3: Finding Radial Acceleration
The radial (centripetal) acceleration ar is given by:
ar=v2R
Substituting v=α√s:
ar=(α√s)2R=α2sR
Step 4: Total Acceleration
Now, we can express the total acceleration a as:
a=√a2t+a2r=√(α22)2+(α2sR)2
This simplifies to:
a=√α44+α4s2R2=α2√14+s2R2
Step 5: Equating to Given Acceleration
We know from the problem statement that:
a=α2√x+s2R2
Setting the two expressions for acceleration equal to each other:
α2√14+s2R2=α2√x+s2R2
Dividing both sides by α2 (since α is positive):
√14+s2R2=√x+s2R2
Step 6: Squaring Both Sides
Squaring both sides gives:
14+s2R2=x+s2R2
Subtracting s2R2 from both sides results in:
14=x
Conclusion
Thus, the value of x is:
14
The total acceleration a of a particle moving in a circular path can be expressed as the vector sum of tangential acceleration at and radial (centripetal) acceleration ar:
a=√a2t+a2r
The tangential acceleration at can be defined as the rate of change of velocity with respect to time:
at=dvdt
Given v=α√s, we differentiate v with respect to t:
dvdt=ddt(α√s)=α⋅12√s⋅dsdt
Since dsdt=v=α√s, we substitute this into the equation:
dvdt=α⋅12√s⋅(α√s)=α22
The radial (centripetal) acceleration ar is given by:
ar=v2R
Substituting v=α√s:
ar=(α√s)2R=α2sR
Now, we can express the total acceleration a as:
a=√a2t+a2r=√(α22)2+(α2sR)2
This simplifies to:
a=√α44+α4s2R2=α2√14+s2R2
We know from the problem statement that:
a=α2√x+s2R2
Setting the two expressions for acceleration equal to each other:
α2√14+s2R2=α2√x+s2R2
Dividing both sides by α2 (since α is positive):
√14+s2R2=√x+s2R2
Squaring both sides gives:
14+s2R2=x+s2R2
Subtracting s2R2 from both sides results in:
14=x
Thus, the value of x is:
14
Topper's Solved these Questions
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Main (Archive)
19 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Advanced (Archive)
19 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - Long Answer Type
5 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
Similar Questions
A particle moves in a circular path such that its speed v varies with distance as V=α√s where α is a positive constant. Find the acceleration of the particle after traversing a distance s.
View SolutionA particle moves in a circular path such that its speed v varies with distance as v=α√s where α is a positive constant. Find the acceleration of particle after traversing a distance S?
View SolutionA particle moves in a circular path such that its speed 1v varies with distance s as v=√s, where ∝ is a positive constant. Find the acceleration of the particle after traversing a distance s.
View SolutionA particle is moving on a circular path with speed given by v= αt, where α is a constant. Find the acceleration of the particle at the instant when it has covered the nth fraction of the circle.
View SolutionThe v−s and v2−s graph are given for two particles. Find the accelerations of the particles at s=0.
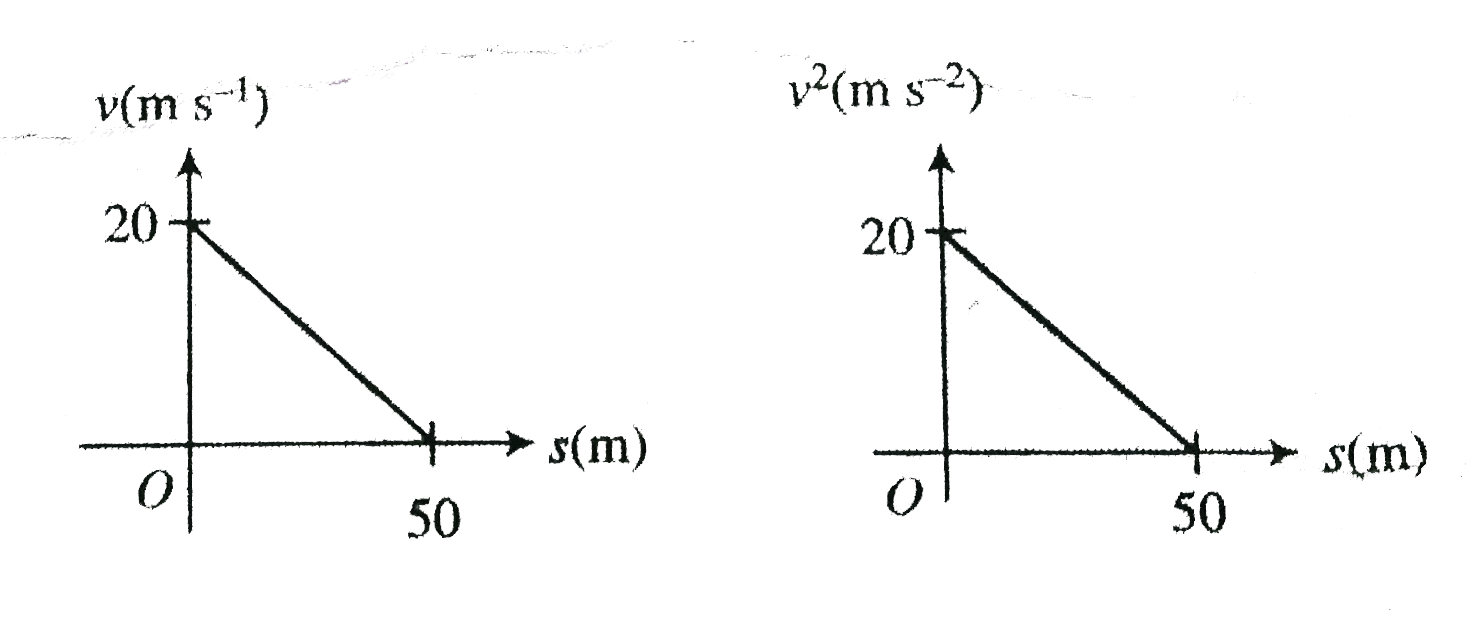 .
.
View SolutionThe kinetic energy of a particle moving along a circle of radius R depends on the distance covered S as K =α.S2, where α is a constant. Find the force acting on the particle as a function of S.
View SolutionA particle of mass 1 kg moves in a circular path of radius 1 m such that its speed varies with time as per equation v=3t2m/s where time t is in seconds. The power delivered by the force acting on the paritcle at t=1s, is :−
View SolutionA particle of unit mass is moving along x-axis. The velocity of particle varies with position x as v(x). =αx−β (where α and β are positive constants and x>0 ). The acceleration of the particle as a function of x is given as
View SolutionA particle is moving on a circular path of radius 1 m with 2 m/s. If speed starts increasing at a rate of 2m/s2, then acceleration of particle is
View SolutionA particle moves in a circle of radius 1 cm at a speed given v=2t, where v is cm/s and t is in seconds. Total acceleration of the particle at t=1 second is 2√ncm/s2. Find value of n.
View Solution
