An aeroplane takes off from Mumbai to Delhi with velocity 50 kph at an angle 30∘ north of east. Wind is blowing at 25 kph from north to south. What is the resultant displacement of aeroplane in 2 hrs. (in km)
The correct Answer is:86−5
To solve the problem of the aeroplane's resultant displacement, we can follow these steps:
Step 1: Identify the Components of the Aeroplane's Velocity
The aeroplane is flying at a velocity of 50 kph at an angle of 30 degrees north of east. We can break this velocity into its x (east) and y (north) components using trigonometric functions.
- East Component (Vx):
Vx=V⋅cos(θ)=50⋅cos(30∘)=50⋅√32=25√3 kph
- North Component (Vy):
Vy=V⋅sin(θ)=50⋅sin(30∘)=50⋅12=25 kph
Step 2: Account for the Wind's Effect
The wind is blowing from north to south at 25 kph. This wind will affect the northward component of the aeroplane's velocity.
- The wind's velocity is in the negative y-direction (south), so we subtract it from the north component of the aeroplane's velocity:
Vy,resultant=Vy−wind speed=25−25=0 kph
Step 3: Calculate the Resultant Velocity
Since the northward component of the velocity cancels out due to the wind, the resultant velocity of the aeroplane is only in the east direction.
- Thus, the resultant velocity (Vr) is:
Vr=Vx=25√3 kph
Step 4: Calculate the Displacement Over Time
To find the displacement over a time period of 2 hours, we use the formula:
Displacement=Velocity×Time
Substituting the values:
Displacement=Vr×t=25√3×2=50√3 km
Step 5: Convert to Decimal Form
To express the displacement in decimal form, we calculate 50√3:
√3≈1.732⟹50√3≈50×1.732≈86.6 km
Final Answer
The resultant displacement of the aeroplane in 2 hours is approximately 86.6 km.
---
The aeroplane is flying at a velocity of 50 kph at an angle of 30 degrees north of east. We can break this velocity into its x (east) and y (north) components using trigonometric functions.
Vx=V⋅cos(θ)=50⋅cos(30∘)=50⋅√32=25√3 kph
Vy=V⋅sin(θ)=50⋅sin(30∘)=50⋅12=25 kph
The wind is blowing from north to south at 25 kph. This wind will affect the northward component of the aeroplane's velocity.
Vy,resultant=Vy−wind speed=25−25=0 kph
Since the northward component of the velocity cancels out due to the wind, the resultant velocity of the aeroplane is only in the east direction.
Vr=Vx=25√3 kph
To find the displacement over a time period of 2 hours, we use the formula:
Displacement=Velocity×Time
Substituting the values:
Displacement=Vr×t=25√3×2=50√3 km
To express the displacement in decimal form, we calculate 50√3:
√3≈1.732⟹50√3≈50×1.732≈86.6 km
The resultant displacement of the aeroplane in 2 hours is approximately 86.6 km.
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Main ( ARCHIVE ) ( LEVEL-1)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 1
75 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)
5 Videos
Similar Questions
An aeroplane has to go from a point A to another point B, 500km away due 30∘ east of north. Wind is blowing due north at a speed of 20m/s. The air-speed of the plane is 150m/s. (a) Find the direction in which the pilot should head the plane to reach the point B. (b) Find the time taken by the plane to go from A to B.
View SolutionAn aeroplane is flying with the velocity of V1 =800 kmph relative to the air towards south. A wind with velocity of V2=15ms−1 is blowing from West to East. What is the velocity of the aeroplane with respect to the earth.
View Solution
Knowledge Check
A Body moves 6m north, 8m east and 10m vertically upwards, what is its resultant displacement from initial position
A10√2 mB10 mC10√2 mD20 m
A body goes 30km south and then 40km east. What will be the displacement from initial point ?
View SolutionAn aeroplane has to go from a point O to another point A, at distance d due 37∘ East of North. A wind is blowing due North at a speed of 10ms−1. The air speed of the plane is v. (i) Find the direction in which the pilot should head the plane to reach the point A. (ii) Find the time taken by the plane to go from O to A.
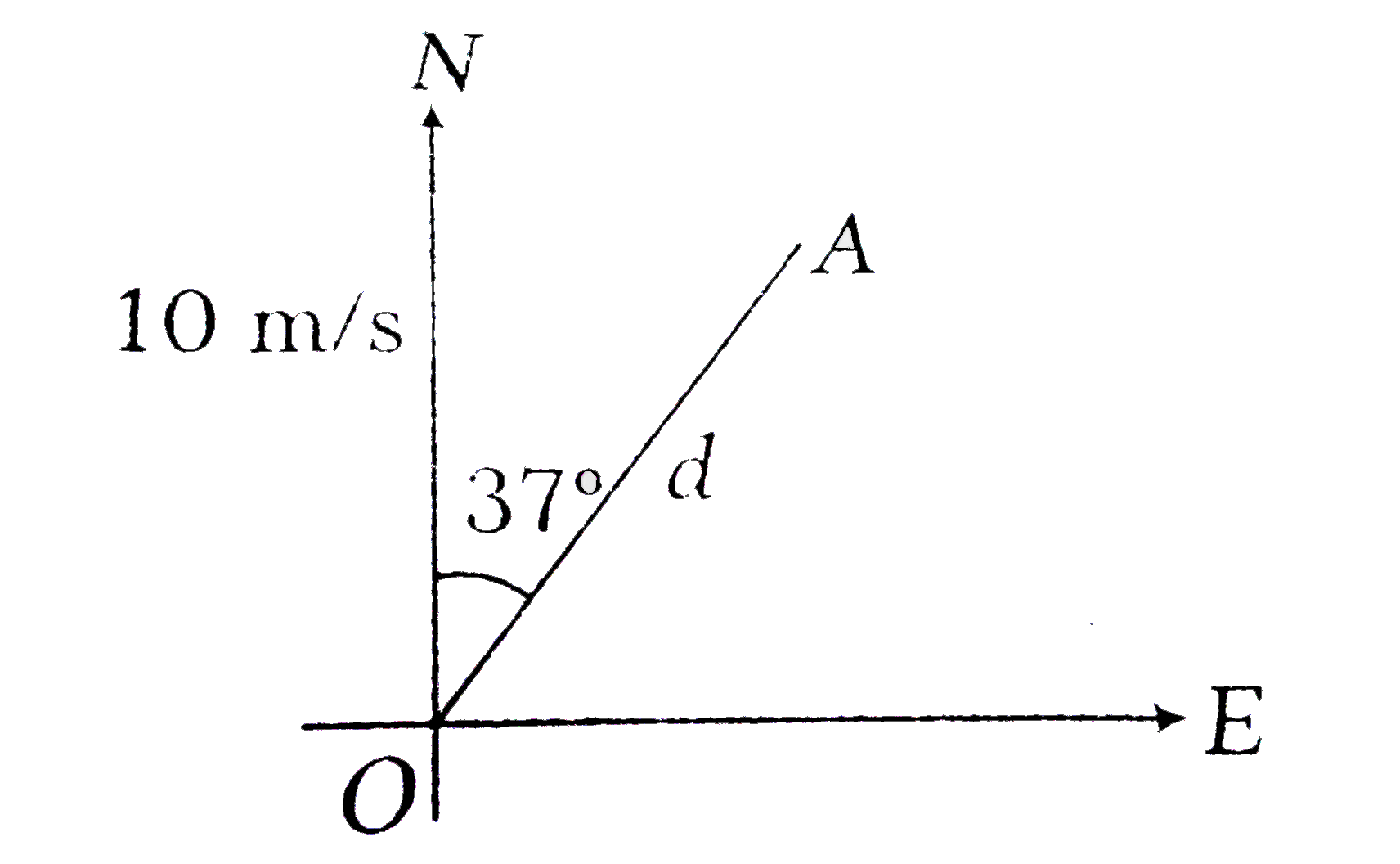
View SolutionA man walks 2 km dur north .After that he walks another 2 km along
due West . What is the final displacement from the starting point in each case ?
View SolutionA hydrogen balloon is flying eastward with speed 12ms−1 , when wind starts blowing from north to south with speed 5ms−1 what is the resultant velocity of balloon ?
View SolutionAn aeroplane is flying in vertical plane at an angle of 30∘ with the horizontal (north) and wind is is blowing from west.A package is dropped from an aeroplane. The velocity of the wind if package hits a kite flying in the space with a position vector →R=(400√3ˆi+80ˆj+200ˆk)m with respect to the point of dropping. (Here ˆi and ˆj are the unit vectors along north and vertically up respectively and ˆk be the unit vector due east. Assume that the bag is light enough to get carried away by the wind)
View SolutionA projectile takes off with an initial velocity of 50m//s at an angle of 37∘ with horizontal, It is just able to clear two hurdles of height 25m each, separated from each other by a distance d Calculate d.
View SolutionA plane moves in windy weather due east while the pilot the plane somewhat south of east. The wind is blowing at 50 km/h directed 30∘ east of north, while the palne moves at 200 km/h relative to the wind . What is the velocity of the plane relative to the ground and what is the direction in which the pilot points the palne ?
View Solution
