State parallelogram law of vector addition. Find analytically the magnitude and direction of resultant vector. Apply it to find the resultant when,
(i) Two vectors are parallel to each other
(ii) Two vectors are perpendicular to each other
Step-by-Step Solution
Step 1: State the Parallelogram Law of Vector Addition
The Parallelogram Law of Vector Addition states that if two vectors →A and →B are represented in magnitude and direction by the two sides of a parallelogram that meet at a point, then the resultant vector →R can be represented in magnitude and direction by the diagonal of the parallelogram that starts from the same point.
Step 2: Find the Magnitude and Direction of the Resultant Vector
To find the resultant vector →R when two vectors →A and →B are given:
1. Magnitude: The magnitude of the resultant vector can be calculated using the formula:
R=√A2+B2+2ABcos(θ)
where θ is the angle between the two vectors.
2. Direction: The direction of the resultant vector can be found using the formula:
tan(ϕ)=Bsin(θ)A+Bcos(θ)
where ϕ is the angle of the resultant vector with respect to vector →A.
Step 3: Case (i) - Two Vectors are Parallel to Each Other
When two vectors →A and →B are parallel (i.e., θ=0∘):
- The magnitude of the resultant vector is:
R=A+B
- The direction of the resultant vector is the same as that of the individual vectors, as they are in the same direction.
Step 4: Case (ii) - Two Vectors are Perpendicular to Each Other
When two vectors →A and →B are perpendicular (i.e., θ=90∘):
- The magnitude of the resultant vector is:
R=√A2+B2
- The direction of the resultant vector can be found using:
tan(ϕ)=BA
This means that the angle ϕ can be calculated as:
ϕ=tan−1(BA)
Summary of Results
1. For Parallel Vectors:
- Magnitude: R=A+B
- Direction: Same as →A and →B
2. For Perpendicular Vectors:
- Magnitude: R=√A2+B2
- Direction: ϕ=tan−1(BA)
The Parallelogram Law of Vector Addition states that if two vectors →A and →B are represented in magnitude and direction by the two sides of a parallelogram that meet at a point, then the resultant vector →R can be represented in magnitude and direction by the diagonal of the parallelogram that starts from the same point.
To find the resultant vector →R when two vectors →A and →B are given:
R=√A2+B2+2ABcos(θ)
where θ is the angle between the two vectors.
tan(ϕ)=Bsin(θ)A+Bcos(θ)
where ϕ is the angle of the resultant vector with respect to vector →A.
When two vectors →A and →B are parallel (i.e., θ=0∘):
- The magnitude of the resultant vector is:
R=A+B
- The direction of the resultant vector is the same as that of the individual vectors, as they are in the same direction.
When two vectors →A and →B are perpendicular (i.e., θ=90∘):
- The magnitude of the resultant vector is:
R=√A2+B2
- The direction of the resultant vector can be found using:
tan(ϕ)=BA
This means that the angle ϕ can be calculated as:
ϕ=tan−1(BA)
1. For Parallel Vectors:
- Magnitude: R=A+B
- Direction: Same as →A and →B
- Magnitude: R=√A2+B2
- Direction: ϕ=tan−1(BA)
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 1
75 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 2
65 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Video
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)
5 Videos
Similar Questions
State parallelogram of law of vector addition .
View SolutionFind the magnitude and direction of the resultant of given vectors
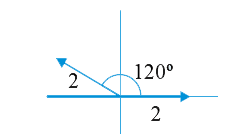
View SolutionTwo vectors →P and →Q that are perpendicular to each other if :
View SolutionFind the magnitude and direction of the resultant of two vectors A and B in the terms of their magnitudes and angle θ between them.

View SolutionTwo vector A and B have equal magnitudes. Then the vector A+B is perpendicular to
View SolutionIn the above problem, this horizontal distance between the two fragments when their position vectors are perpendicular to each other is
View Solution(a) Write law of parallelogram of vector and obtain the expression for the resultant of two vectors acting in two different directions.
View SolutionIs it possible that dot product of two vectors is zero even if they are not perpendicular to each other ?
View SolutionIn Q-2, if vectors are perpendicular to each other then find the value of λ.
View SolutionTwo vectors 3 units and 5 units are acting at 60∘ to each other .What is the magnitude and direction of the resultant ?
View Solution
