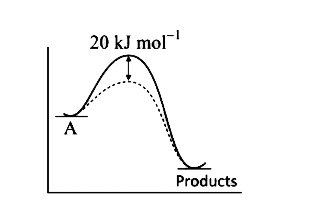
For following reactions
A400K−−→ Product
A200K−−−−−→catalyst Product
it was found that the Ea is decreased by 20 kJ/ mol in the presence of catalyst. If the rate remains unchanged, the activation energy for catalysed reaction is ( Assume per exponential factor is same ) :
40 kJ/ mol
20kJ /mol
80kJ/mol
35kJ/mol
To solve the problem, we need to analyze the activation energy (Ea) for both the uncatalyzed and catalyzed reactions, and how they relate to the rate of the reaction.
1. Define the Variables:
- Let Ea1 be the activation energy for the uncatalyzed reaction at 400 K.
- Let Ea2 be the activation energy for the catalyzed reaction at 200 K.
- It is given that Ea1−Ea2=20kJ/mol.
2. Use the Arrhenius Equation:
The Arrhenius equation is given by:
k=Ae−EaRT
where k is the rate constant, A is the pre-exponential factor, R is the gas constant, and T is the temperature in Kelvin.
3. Set Up the Equations:
For the uncatalyzed reaction at 400 K:
k1=Ae−Ea1R⋅400
For the catalyzed reaction at 200 K:
k2=Ae−Ea2R⋅200
4. Equate the Rate Constants:
Since the rate remains unchanged, we have k1=k2:
Ae−Ea1R⋅400=Ae−Ea2R⋅200
We can cancel A from both sides (assuming A is the same for both reactions):
e−Ea1R⋅400=e−Ea2R⋅200
5. Take the Natural Logarithm:
Taking the natural logarithm of both sides gives:
−Ea1R⋅400=−Ea2R⋅200
This simplifies to:
Ea1400=Ea2200
6. Express Ea1 in terms of Ea2:
Rearranging gives:
Ea1=2Ea2
7. Substitute the Relationship:
Now we can substitute Ea1 in terms of Ea2 into the equation Ea1−Ea2=20:
2Ea2−Ea2=20
This simplifies to:
Ea2=20kJ/mol
8. Find Ea1:
Using Ea1=2Ea2:
Ea1=2×20=40kJ/mol
Final Answer:
The activation energy for the catalyzed reaction is:
Ea2=20kJ/mol
- Let Ea1 be the activation energy for the uncatalyzed reaction at 400 K.
- Let Ea2 be the activation energy for the catalyzed reaction at 200 K.
- It is given that Ea1−Ea2=20kJ/mol.
The Arrhenius equation is given by:
k=Ae−EaRT
where k is the rate constant, A is the pre-exponential factor, R is the gas constant, and T is the temperature in Kelvin.
For the uncatalyzed reaction at 400 K:
k1=Ae−Ea1R⋅400
For the catalyzed reaction at 200 K:
k2=Ae−Ea2R⋅200
Since the rate remains unchanged, we have k1=k2:
Ae−Ea1R⋅400=Ae−Ea2R⋅200
We can cancel A from both sides (assuming A is the same for both reactions):
e−Ea1R⋅400=e−Ea2R⋅200
Taking the natural logarithm of both sides gives:
−Ea1R⋅400=−Ea2R⋅200
This simplifies to:
Ea1400=Ea2200
Rearranging gives:
Ea1=2Ea2
Now we can substitute Ea1 in terms of Ea2 into the equation Ea1−Ea2=20:
2Ea2−Ea2=20
This simplifies to:
Ea2=20kJ/mol
Using Ea1=2Ea2:
Ea1=2×20=40kJ/mol
The activation energy for the catalyzed reaction is:
Ea2=20kJ/mol
Topper's Solved these Questions
Similar Questions
For the following reaction,
A500K−−→ Products
A400K/catalyst−−−−−−−−→ Product
If (−d[A]dt)500K=−(d[A]dt)400K
no catalyst in presence of catalyst Then Ea is approximately :
View SolutionAt 227∘C , the presence of catalyst causes the activation energy of a reaction to decrease by 4.606Kcal.The rate of the reaction will be increased by :−
View SolutionA hydrogenation reaction is carried out at 500K. If the same reaction is carried out in the presence of a catalyst at the same rate, the temperature required is 400K. Calculate the activation energy of the reaction if the catalyst lowers the activation barrier by 20kJmol−1.
View SolutionRate of reaction in absence of catalyst at 700 K is same as in presence of catalyst at 500 K. If catalyst decreases activation energy barrier by 30 kJ/mole, determine activation energy in presence of catalyst. (Assume 'A' factor to be same in both cases)
View SolutionRate of reaction in absence of catalyst at 700 K is same as in presence of catalyst at 500 K. If catalyst decreases activation energy barrier by 30 kJ/mole, determine activation energy in presence of catalyst. (Assume 'A' factor to be same in both cases)
View SolutionA reaction is carried out at 600K. If the same reaction is carried out in the presence of catalyst at the same rate and same frequency factor, the temperature required is 500K. What is the activation energy of the reaction, if the catalyst lowers the activation energy barrier by 20KJ/mol ?
View SolutionThe rate constant of a reaction at temperature 200 K is 10 times less than the rate constant at 400 K. What is the activation energy (Ea) of the reaction ? (R = gas constant)
View SolutionA catalyst decreases Ea form 100KJmol−1 to 80KJmol−1. At what temperature the rate of reaction in the absence of catalyst at 500K will be equal to rate reaction in the presence of catalyst ?
View SolutionThe activation energy for a first order reaction is 60 kJ mol−1 in the absence of a catalyst and 50 kJ mol−1 in the presence of a catalyst. How many times will the rate of reaction grow in the presence of the catalyst if the reaction proceeds at 27∘C ?
View SolutionThe activation energy for a first order reaction is 60 kJ mol−1 in the absence of a catalyst and 50 kJ mol−1 in the presence of a catalyst. How many times will the rate of reaction grow in the presence of the catalyst if the reaction proceeds at 27∘C ?
View Solution
