A given LCR series circuit satisfes the condition for resonance with a given AC source. If the angular frequency of the AC source is increased by 100% then in order to establish resonance, and without changing the value of inductance, capacitance must be
(a)Increased by 100%
(b)Reduced by 50%
(c)Increased by 75%
(d)Reduced by 75%
The correct Answer is:D
To solve the problem step by step, we need to understand the concept of resonance in an LCR circuit and how the change in angular frequency affects the capacitance required for resonance.
1. Understanding Resonance Condition:
In an LCR series circuit, resonance occurs when the inductive reactance (XL) equals the capacitive reactance (XC). Mathematically, this is expressed as:
XL=XC
where XL=ωL and XC=1ωC.
2. Initial Condition:
At resonance, we have:
ωL=1ωC
Rearranging gives:
ω2LC=1(1)
3. Change in Angular Frequency:
The problem states that the angular frequency of the AC source is increased by 100%. Therefore, the new angular frequency (ω′) is:
ω′=2ω
4. New Resonance Condition:
For the new frequency, the resonance condition becomes:
ω′L=1ω′C′
Substituting ω′=2ω gives:
2ωL=12ωC′
5. Rearranging the New Condition:
Rearranging the above equation yields:
4ω2LC′=1(2)
6. Relating the Two Conditions:
From equation (1), we know ω2LC=1. Substituting this into equation (2):
4⋅1=1⇒C′=C4
7. Conclusion:
To establish resonance with the new frequency, the capacitance must be reduced to:
C′=C4
This means the capacitance must be decreased to one-fourth of its original value.
In an LCR series circuit, resonance occurs when the inductive reactance (XL) equals the capacitive reactance (XC). Mathematically, this is expressed as:
XL=XC
where XL=ωL and XC=1ωC.
At resonance, we have:
ωL=1ωC
Rearranging gives:
ω2LC=1(1)
The problem states that the angular frequency of the AC source is increased by 100%. Therefore, the new angular frequency (ω′) is:
ω′=2ω
For the new frequency, the resonance condition becomes:
ω′L=1ω′C′
Substituting ω′=2ω gives:
2ωL=12ωC′
Rearranging the above equation yields:
4ω2LC′=1(2)
From equation (1), we know ω2LC=1. Substituting this into equation (2):
4⋅1=1⇒C′=C4
To establish resonance with the new frequency, the capacitance must be reduced to:
C′=C4
This means the capacitance must be decreased to one-fourth of its original value.
Topper's Solved these Questions
Similar Questions
For an LCR series circuit with an ac source of angular frequency ω.
View SolutionFor an LCR series circuit with an aac source of angular frequency ω.
View SolutionA bulb and a conender are connected in series with an A.C source. On increasing the frequency of the source its brightness will
View SolutionIn series LCR circuit voltage leads the current. When
(Given that ω0 = resonant angular frequency)
View SolutionConsider in L−C−R circuit as shown in figureure with an AC source of peak value V0 and angular frequency ω. Then the peak value of current through the AC
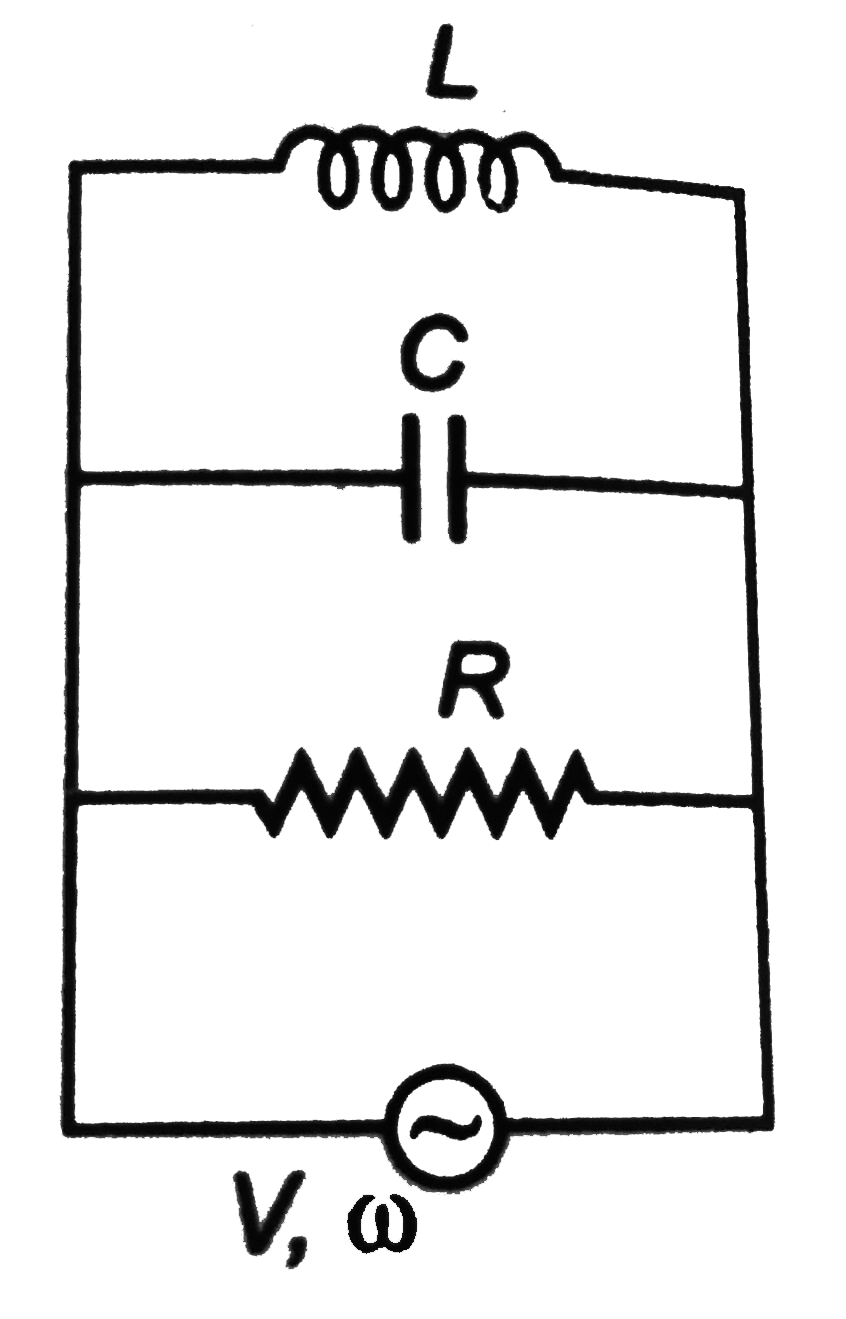 .
.
View SolutionAn LCR series circuit is in resonance with the frequency of applied ac generator. Select the incorrect statement:
View SolutionIn a series LCR circuit resonance of frequency is the half of the frequency of source.The nature of the circuit is
View SolutionIn a series LCR circuit, operated with an ac of angular frequency ω , the total impedance is
View SolutionA series LCR circuit containing a resistance of 120Ω has angular resonance frequency 4×105rads−1. At resonance the vlotage across resistance and inductance are 60V and 40 V, repectively,
the value of inductance L is
View SolutionA series LCR circuit with L=4.0H,C=100μF and R=60Ω is connected to a variable frequency 240 V source. Calculate
(i) angular frequency of the source which drives the circuit in resonance,
(ii) current at the resonating frequency,
(iii) rms potential drop across the inductance at resonance.
View Solution