A square loop is carrying a steady current I and the magnitude of its magnetic dipole moment is m. if this square loop is changed to a circular loop and it carries the current, the magnitude of the magnetic dipole moment of circular loop will be :
4mπ
2mπ
3mπ
mπ
The correct Answer is:A
To solve the problem, we need to find the magnetic dipole moment of a circular loop when we know the magnetic dipole moment of a square loop carrying the same current.
1. Magnetic Dipole Moment of Square Loop:
The magnetic dipole moment m of a square loop is given by the formula:
m=n⋅I⋅A
where:
- n is the number of turns (which is 1 in this case),
- I is the current,
- A is the area of the square loop.
For a square loop with side length a, the area A is:
A=a2
Therefore, the magnetic dipole moment for the square loop becomes:
m=I⋅a2
2. Relating the Side Length to the Radius of the Circular Loop:
When the square loop is transformed into a circular loop, we need to relate the side length a of the square to the radius r of the circular loop. The perimeter of the square is equal to the circumference of the circle:
4a=2πr
From this, we can solve for r:
r=4a2π=2aπ
3. Area of the Circular Loop:
The area A′ of the circular loop is given by:
A′=πr2
Substituting the expression for r:
A′=π(2aπ)2=π⋅4a2π2=4a2π
4. Magnetic Dipole Moment of Circular Loop:
The magnetic dipole moment m′ of the circular loop is given by:
m′=n⋅I⋅A′=1⋅I⋅4a2π=4Ia2π
5. Expressing m′ in terms of m:
Recall that m=I⋅a2. Therefore:
m′=4πm
Final Answer:
The magnitude of the magnetic dipole moment of the circular loop is:
m′=4mπ
The magnetic dipole moment m of a square loop is given by the formula:
m=n⋅I⋅A
where:
- n is the number of turns (which is 1 in this case),
- I is the current,
- A is the area of the square loop.
A=a2
Therefore, the magnetic dipole moment for the square loop becomes:
m=I⋅a2
When the square loop is transformed into a circular loop, we need to relate the side length a of the square to the radius r of the circular loop. The perimeter of the square is equal to the circumference of the circle:
4a=2πr
From this, we can solve for r:
r=4a2π=2aπ
The area A′ of the circular loop is given by:
A′=πr2
Substituting the expression for r:
A′=π(2aπ)2=π⋅4a2π2=4a2π
The magnetic dipole moment m′ of the circular loop is given by:
m′=n⋅I⋅A′=1⋅I⋅4a2π=4Ia2π
Recall that m=I⋅a2. Therefore:
m′=4πm
The magnitude of the magnetic dipole moment of the circular loop is:
m′=4mπ
Topper's Solved these Questions
- JEE MAIN REVISION TEST - 13Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 13Exercise:PHYSICS (SECTION 2)
Explore 5 Videos
- JEE MAIN REVISION TEST - 12 Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 12 Exercise:PHYSICS
Explore 25 Videos
- JEE MAIN REVISION TEST - 18Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 18Exercise:PHYSICS - SECTION 2
Explore 5 Videos
Similar Questions
A square loop is carrying a steady current I and the magnitude of its magnetic dipole moment is m. If this square loop is changed to a circular loop and it carries the same current, the magnitude of the magnetic dipole moment of circular loop will be:
View SolutionMagnetic dipole moment of rectangular loop is
View SolutionThe magnetic dipole moment of current loop is independent of
View SolutionIn the given option,the magnetic dipole moment of current loop is independent of
View SolutionCompute the magnetic dipole moment of the loop shown in Fig. 1.69
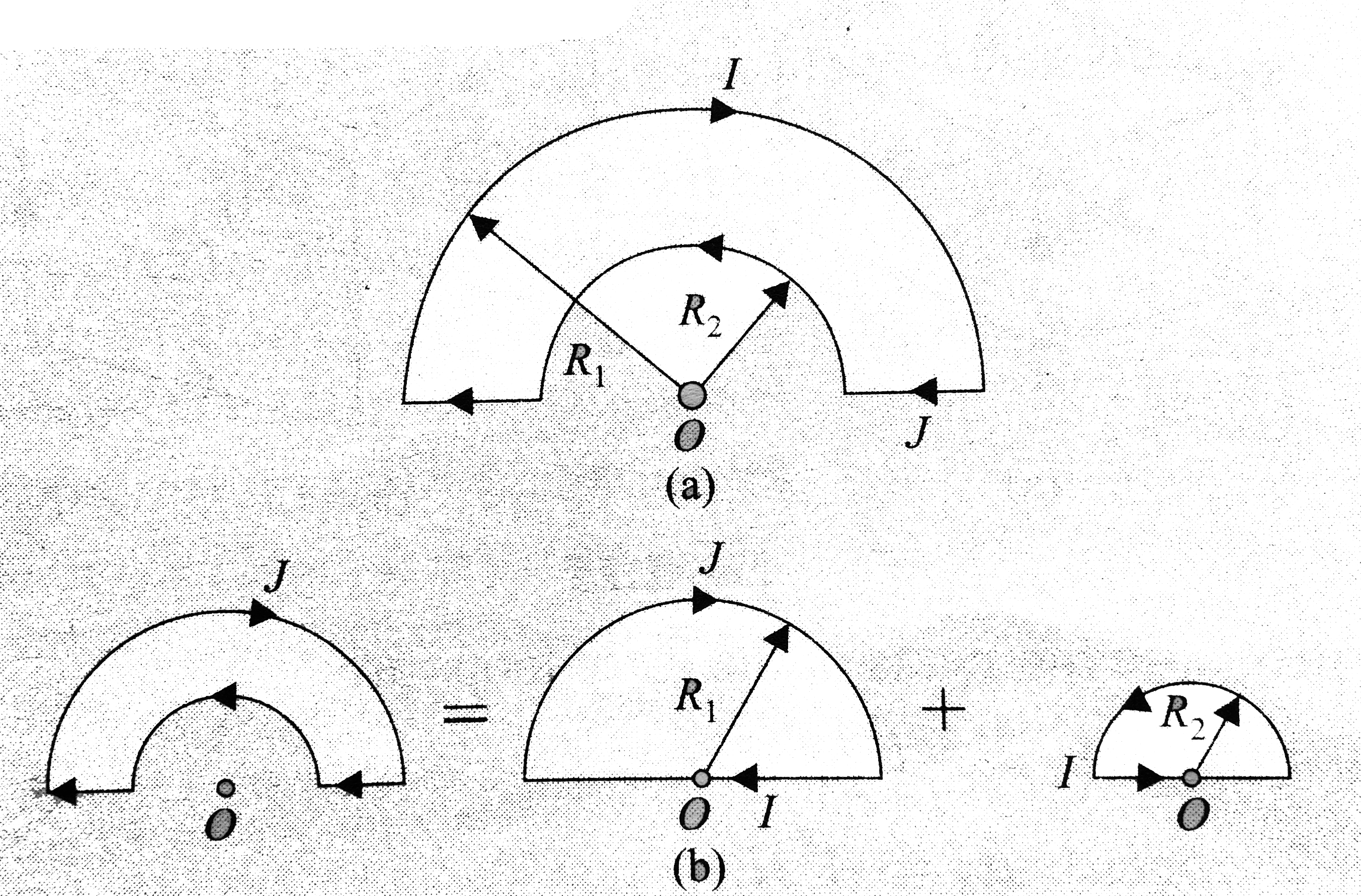
View SolutionA circular loop carrying a current is replaced by an equivalent magnetic dipole. A point on the axis of the loop is in
View SolutionA circular loop carrying a current is replaced by an equivalent magnetic dipole. A point on the axis of the loop is in
View SolutionA thin circular wire carrying a current I has a magnetic moment M. The shape of the wire is changed to a square and it carries the same current. It will have a magnetic moment
View SolutionA current - carrying loop is shown in the figure. The magnitude of the magnetic field produced at a point O is
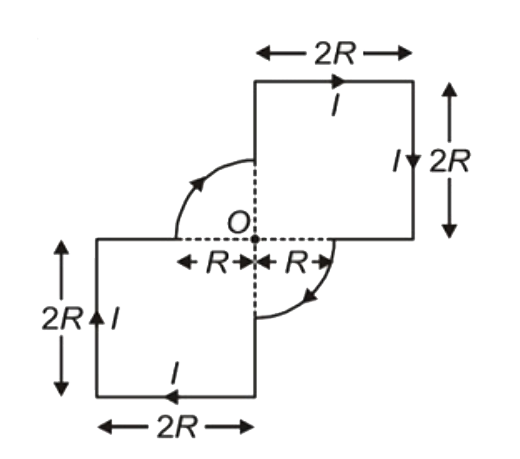
View SolutionA square loop of side a carris a current I. The magnetic field at the centre of the loop is
View Solution