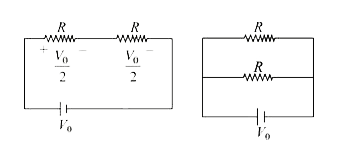
Two equal resistances when connected in series to a battery ,consume electric power of 60 W .If these resitances are now connected in parealled combination to the same battery ,the electric power cansumed will be :
The correct Answer is:B, D
P0=1R(V02)2×2=V202R
P=V20R×2
So, PP0=4
∵P0=60w ⇒ P = 60 x 4 = 240 w
Topper's Solved these Questions
Similar Questions
Four equal resistance dissipated 5 W of power together when connected in series to a battery of negligible internal resistance . The total power dissipated in these resistance when connected in parallel across the same battery would be .
View SolutionThree equal resistances connected is series across a source of e.m.f consume 20 watt. If the same resistor are connected in parallel across the same source of e.m.f., what would be the power dissipated ?
View SolutionTwo identical capacitors of 12pF each are connected in a series across a battery of 50V. How much electrostatic energy is stored in the combination ? If these were connected in parallel across the same battery , how much energy will be stored in combintion now ?
Also find the charge drawn from the battery in each case.
View SolutionWhen three identical bulbs are connected in series. The consumed power is 10W. If they are now connected in pa rallel then the consumed power will be:-
View SolutionThe Total resistance when connected in series in 9Ω and when connected in parallel is 2Ω The value of two resistance are
View SolutionA set of 'n' equal resistor, of value of 'R' each are connected in series to a battery of emf 'E' and internal resistance 'R'. The current drawn is I. Now, the 'n' resistors are connected in parallel to the same battery. Then the current drawn from battery becomes 10.1. The value of 'n' is
View SolutionWhen two resistances R1andR2 are connected in series , they consume 12 W powers . When they are connected in parallel , they consume 50 W powers . What is the ratio of the powers of R1andR2 ?
View SolutionTwo electric bulbs A and B are rated as 60 W and 100 W . They are connected in parallel to the same source. Then,
View SolutionAssertion : Two identical bulbs when connected across a battery, produce a total power P. When they are connected across the same battery in series total power consumed will be P4.
Reason : In parallel, P=P1+P2 and in series P=P1P2P1+P2
View SolutionTwo resistors of resistances 2Ωand4Ω when connected to a battery will have :
View Solution
