Find the graphical solutions for the system of linear inequations 2x+y≤2,x−y≤1

Video Solution
Doubtnut Promotions Banner
Text Solution
Generated By DoubtnutGPT

To find the graphical solutions for the system of linear inequalities 2x+y≤2 and x−y≤1, we will follow these steps:

Step 1: Convert Inequalities to Equations
We start by converting the inequalities into equations to find the lines that represent the boundaries of the inequalities.

1. For the first inequality 2x+y≤2, we convert it to the equation:
2x+y=2

2. For the second inequality x−y≤1, we convert it to the equation:
x−y=1

Updated on:18/9/2024
|
Class 12MATHSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The graphical solution of the system of linear inequalities 3x,4y≥12,y≥1,x≥0 is

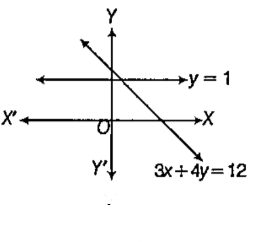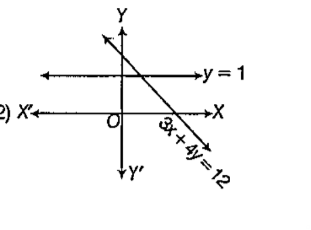    Aocr_image
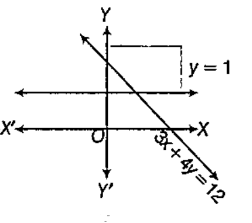    Bocr_image
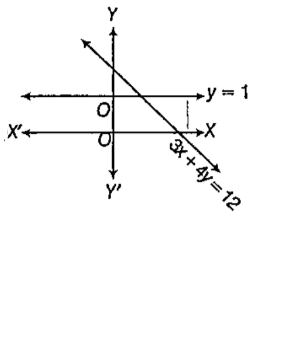
    Cocr_image
    Docr_image

  • The carner points of the feasible region formed by the system of linear inequations x−y≥−1,−x+y≥0,x+y≤2andx,y≥0, are

    A(0,0),(−1,0),(0,1)
    B(0,0),(2,0),(1,1)
    C(0,1),(1,1),(0,0)
    D(0,0),(1,1),(12,32),(0,1)

  • The feasible region of the system of linear inequations x+2y≤120,x+y≥60,x−2y≥0,x,y≥0:

    Ais bounded and lies in first quadrant .
    Bis unbounded and lies in first quadrant.
    Cdoes in first quadrant .
    Ddoes not exist.