∣∣bxy+byx∣∣≥_________
The correct Answer is:2|r|
To solve the question regarding the relationship between the regression coefficients bxy and byx, we will follow these steps:
1. Understanding Regression Coefficients:
- Let bxy be the regression coefficient of x on y.
- Let byx be the regression coefficient of y on x.
- We denote the correlation coefficient as r.
2. Using the Relationship between Correlation and Regression Coefficients:
- There is a known relationship which states that:
r2=bxy⋅byx
- From this, we can express r as:
r=±√bxy⋅byx
3. Applying the Absolute Value:
- Taking the absolute value, we have:
|r|=√|bxy⋅byx|
4. Using the Arithmetic Mean-Geometric Mean Inequality:
- By the Arithmetic Mean-Geometric Mean (AM-GM) inequality:
bxy+byx2≥√bxy⋅byx
- This implies:
|bxy+byx|≥2√|bxy⋅byx|
5. Substituting the Expression for |r|:
- Since |r|=√|bxy⋅byx|, we can substitute this into our inequality:
|bxy+byx|≥2|r|
Final Answer:
Thus, we conclude that:
|bxy+byx|≥2|r|
- Let bxy be the regression coefficient of x on y.
- Let byx be the regression coefficient of y on x.
- We denote the correlation coefficient as r.
- There is a known relationship which states that:
r2=bxy⋅byx
- From this, we can express r as:
r=±√bxy⋅byx
- Taking the absolute value, we have:
|r|=√|bxy⋅byx|
- By the Arithmetic Mean-Geometric Mean (AM-GM) inequality:
bxy+byx2≥√bxy⋅byx
- This implies:
|bxy+byx|≥2√|bxy⋅byx|
- Since |r|=√|bxy⋅byx|, we can substitute this into our inequality:
|bxy+byx|≥2|r|
Thus, we conclude that:
|bxy+byx|≥2|r|
Topper's Solved these Questions
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 3. LINEAR REGRESSION (IV.Answer the following:)
15 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 3. LINEAR REGRESSION (V. Activity questions:)
8 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 3. LINEAR REGRESSION (II State whether the following statements are true or false:)
17 Videos
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
Similar Questions
Knowledge Check
bxy and byx are __________
AIndependent of change of origin and scaleBIndependent of change of origin but not of scaleCIndependent of change of scale but not of originDAffected by change of origin and scalebxy×byx is equal to
Aρ(X,Y)BCov (X,Y)C[p(X,Y)]2DNone of theseIf byx<0 and bxy<0 then r is _____
Aa<0Bb>0Cc=0Dd>1
Find the regression coefficient byx and bxy and the two lines of regression for the following data. Also compute the correlation coefficient
View SolutionFind the regression coefficients byxandbxy between x and y for the data n=8,∑x=36,∑y=48,∑xy=225,∑x2=146,∑y2=324.
View SolutionIf bxy=−32,byx=−16, then value of r is ……………..
View Solution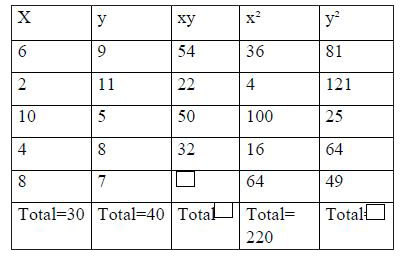
bxy=□□
byx=□□
∴ Regression equation of x on y is □
∴ Regression equation of y on x is □
View SolutionIf for a bivariate data, byx=−1.2 and bxy=−0.3 then r =
View Solution
