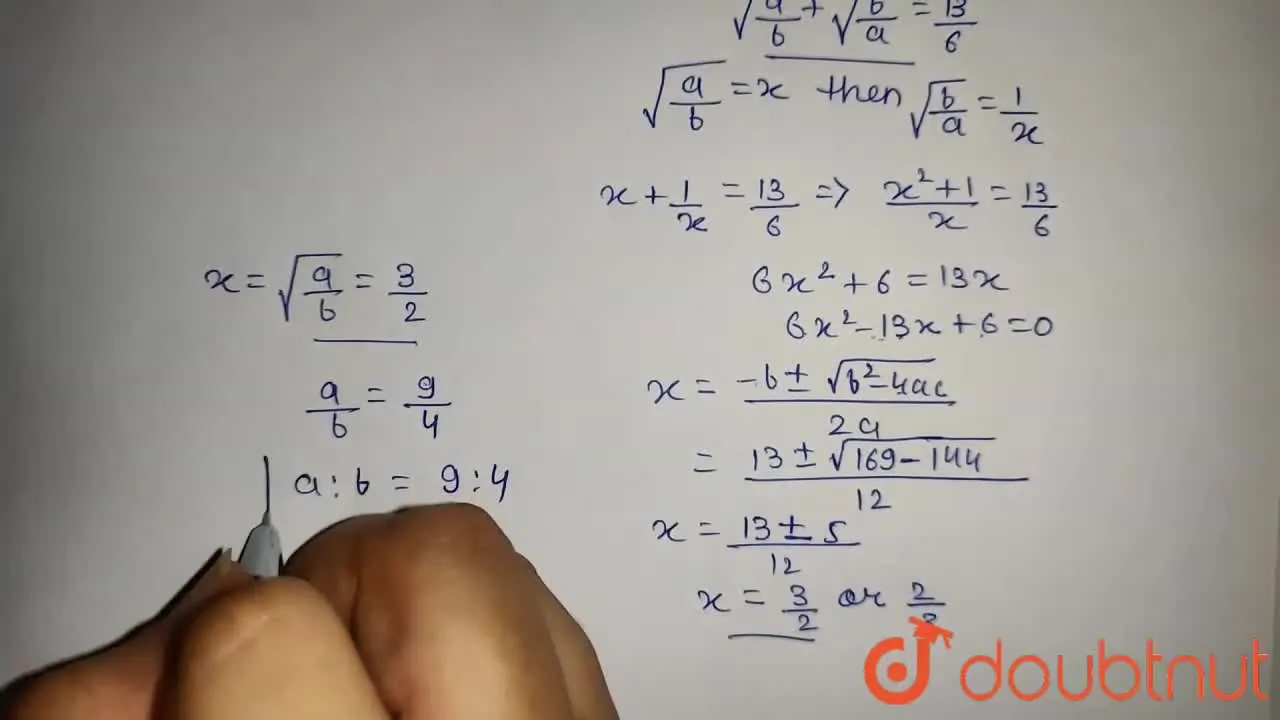
If the ratio of H.M. between two positive numbers 'a' and 'b' (a>b) is to their G.M. as 12 to 13, prove that a:bis9:4.
Step by step video & image solution for If the ratio of H.M. between two positive numbers 'a' and 'b' (a gt b) is to their G.M. as 12 to 13, prove that a:b is 9:4. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - SEQUENCE & SERIES EXERCISE - EXERCISE -1 PART -I RMO43videos
SEQUENCE & SERIES
- BOOK - RESONANCE ENGLISHCHAPTER - SEQUENCE & SERIES EXERCISE - EXERCISE -1 PART -II RMO1videos
SEQUENCE & SERIES
- BOOK - RESONANCE ENGLISHCHAPTER - SEQUENCE & SERIES EXERCISE - EXERCISE -2 (PART-II : PREVIOUSLY ASKED QUESTION OF RMO)3videos
SEQUENCE & SERIES
- BOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - SSP55videos
RELATION, FUNCTION & ITF
- BOOK - RESONANCE ENGLISHCHAPTER - TEST PAPEREXERCISE - MATHEMATICS48videos
TEST PAPER
Similar Questions
If the ratio of AM to GM of two positive numbers a and b is 5:3, then a:b is
View SolutionIf the arithmetic means of two positive number a and b (a>b) is twice their geometric mean, then find the ratio a: b
View SolutionIf the ratio of H.M. and G.M. between two numbers a and b is 4:5, then find the ratio of the two number ?
View SolutionThe A.M. and H.M. between two numbers are 27 and 12, respectively, then find their G.M.
View SolutionIf G1 is the first of n G.M. s between positive numbers a and b , then show that Gn+11=anb .
View SolutionThe A.M. and H.M. between two numbers are 27 and 122, respectively, then find their G.M.
View SolutionIf the A.M. between two positive numbers exceeds their G.M. by 2 and the ratio of the numbers
be 1:4 find the numbers .View SolutionIf H1 and H2 are two harmonic means between two positive numbers a and b (a≠b) , A and G are the arithmetic and geometric menas between a and b , then H2+H1H2H1 is
View SolutionProve that the A.M. of two positive real numbers is greater than their G.M.
View SolutionIf A1, A2, A3 , G1, G2, G3 , and H1, H2, H3 are the three arithmetic, geometric and harmonic means between two positive numbers a and b(a>b), then which of the following is/are true ?
View Solution