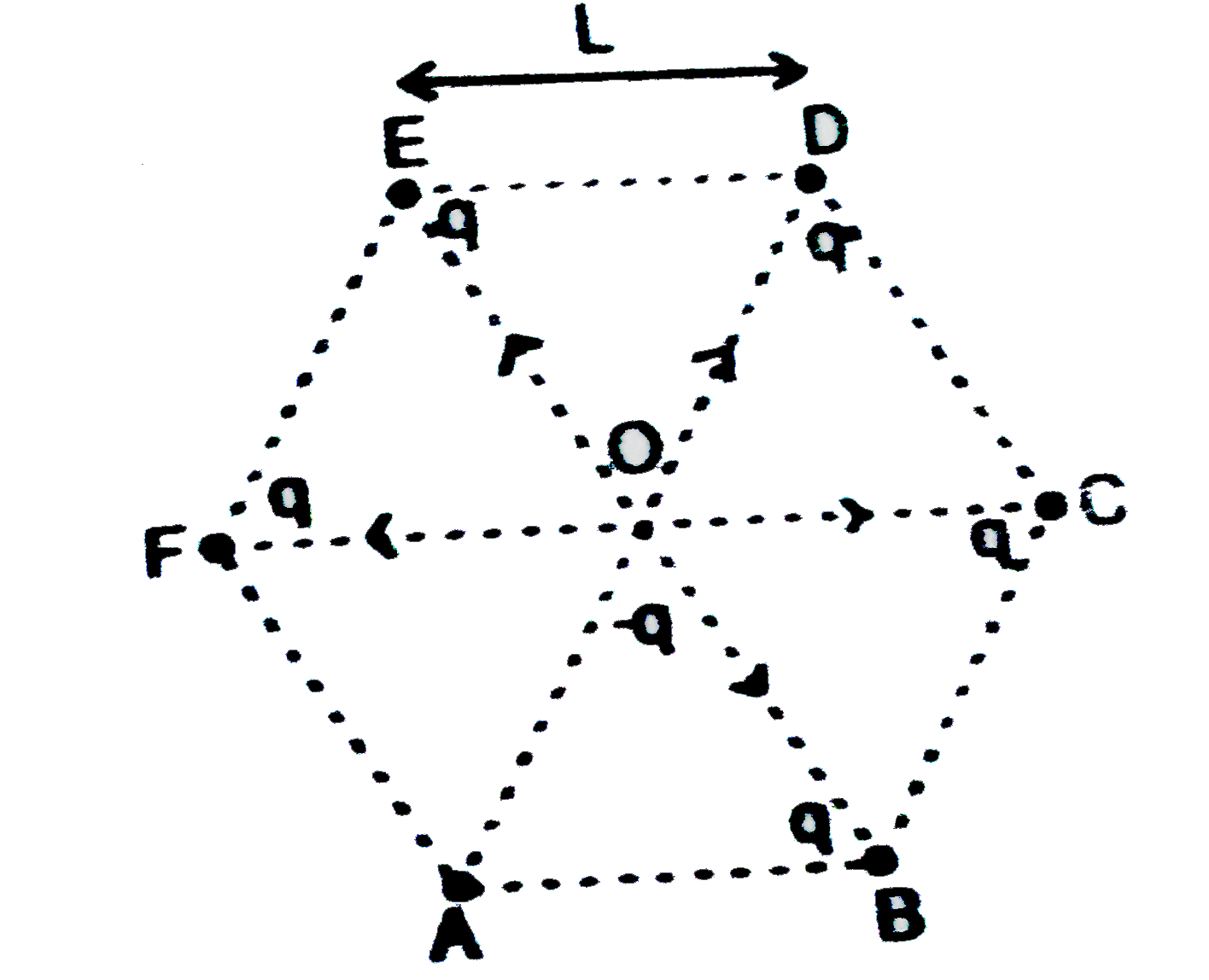
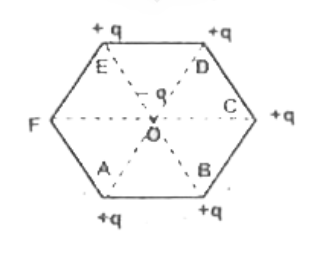
Five point charge each of value +q are placed on five vertices of a regular hexagon of side a metre. What is the magnitude of the force on a point charge of value $- q$ coulomb placed at the centre of the hexagon?

Video Solution

Text Solution

Verified by Experts

Method-I : If there had been a sixth charge +q at the remaining vertex of hexagon, force due to all the six charges on -q at O would have been zero (as the forces due to individual charges will balance each other), i.e., $- \to F_{R} = 0$
Now if $\to f$ is the force due to sixth charge and $\to F$ due to remaining five charges.
From $\to F + \to f = 0$ i.e. $\to F = - \to f$
or, $| F | = | f | = \frac{1}{4 π ε_{0}} \frac{q \times q}{L^{2}} = \frac{1}{4 π ε_{0}} \frac{q^{2}}{L^{2}}$
${\to F}_{Net} = {\to F}_{O D} = \frac{1}{4 π ε_{0}} \frac{q^{2}}{L^{2}}$ along $O D$

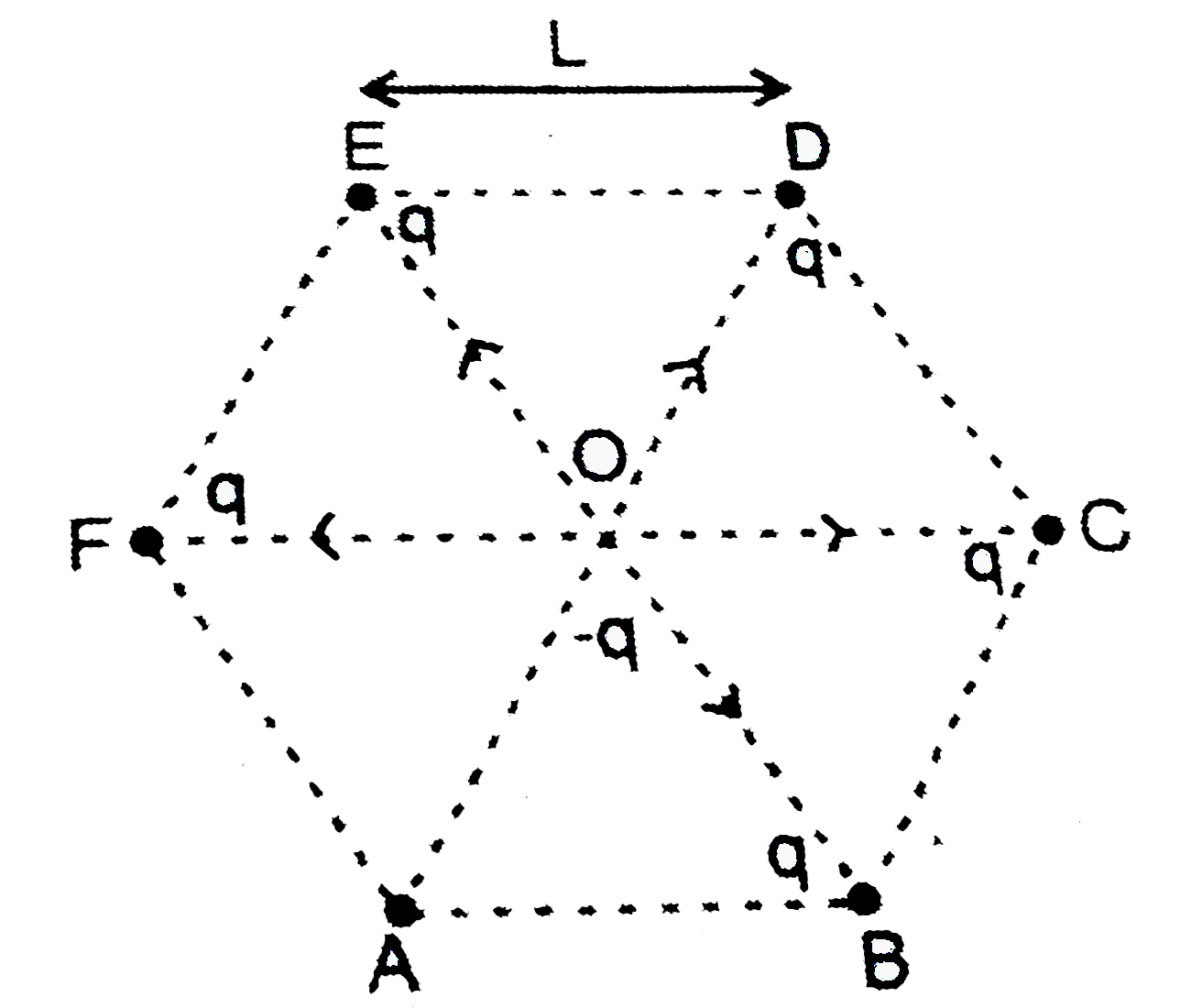
Method-II : In the diagram, we can see that force due to charge A and D are opposite to each other
${\to F}_{O F} + {\to F}_{O C} = \to 0$ ....(i)
Similarly ${\to F}_{O B} + {\to F}_{O E} = \to 0$ ...(ii)
So ${\to F}_{O F} + {\to F}_{O B} + {\to F}_{O C} + {\to F}_{O D} + {\to F}_{O E} = {\to F}_{Net}$
Using (i) and (ii) ${\to F}_{Net} = {\to F}_{O D} = \frac{1}{4 π ε_{0}} \frac{q^{2}}{L^{2}}$ along OD.

Updated on:21/07/2023