Show that f(x) =(x3−6x2+12x−8) does not have any point of local maxima or minima . Hence draw graph
f(x)=x3=6x2+12x−8
f(x)=3(x2−4x+4)
f(x)=3(x−2)2
f(x)=0 ⇒ x=2
But clearly f(x) does not change sigh about x=2. f(2)<0 and f(2)<0.So f(x) has no point of maxima or minima . In fact f(x) is a monotonically increasing function for x∈R

Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Self Practice Problems
36 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise -1A
5 Videos
- COMBINATORICSBOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)
5 Videos
Similar Questions
Find points of local maxima /minima of
f(x)=x2e−x.
View SolutionDraw graph of f(x) =x|x−2| and hence find points of local maxima/minima.
View SolutionFind the points of local maxima or minima for f(x)= sin 2x−x,x∈(0,π)
View SolutionFind the points of local maxima and minima of the function f(x)=x2−4x.
View SolutionDraw the graph of f(x)=12x43−6x13
View SolutionFind all the points of local maxima and minima of the function f(x)=x3−6x2+9x−8 .
View SolutionLet f(x) =x3−x2−x−4
(i) find the possible points of maxima /minima of f(x) x∈R
(ii) Find the number of critical points of f(x) x∈[1,3]
(iii) Discuss absolute (global) maxima / minima value of f(x) for x∈[−2,2]
(iv) Prove that for x∈(1,3) the function does not has a Global maximum.
View SolutionFind the points of maxima or minima of f(x) =x2(x−2)2.
View SolutionLet f(x)={x3+x2+10x,x<0.Investigate x=0 for local
−3sinx,x≥0
maxima/minima.
View SolutionIn each of following graphs identify if x= a is point of local maxima minima or neither
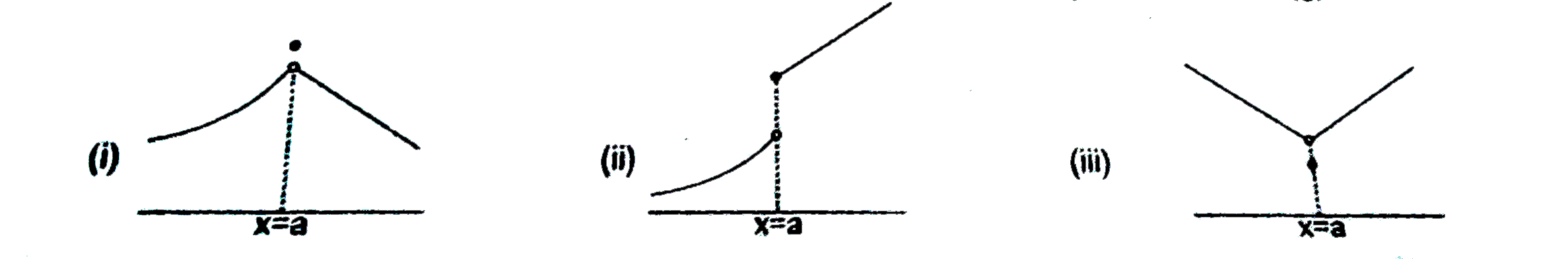
View Solution
