A long straight wire along the Z-axis carries a current I in the negative z-direction. The magnetic vector field B at a point having coodinates (x, y) in the z = 0 plane is
μ0i2π(yˆi−xˆj)(x2+y2)
μ0i2π(xˆi+yˆj)(x2+y2)
μ0i2π(xˆj−yˆi)(x2+y2)
μ0i2π(xˆj−yˆi)(x2+y2)
The correct Answer is:A
Magnetic field at P is ¯¯¯B, perpendicular to OP in the direction shown in figure.
So, ¯¯¯B=Bsinθˆi−Bcosθˆj
Here B=μ02πIr
sinθ=yr and cosθ=xr
∴¯¯¯B=μ0I2π1r2(yˆi−xˆj)=μ0I(yˆi−xˆj)2π(x2+y2)

Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-2 PART-2
26 Videos
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-2 PART-3
18 Videos
- ELECTRODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-1 PART-2
54 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
Similar Questions
A long straight wire along the z- axis carries a current I in the negative z−direction . The magnetic vector field →B at a point having coordinates (x,y) in the Z=0 plane is
View SolutionA long straight wire along the z- axis carries a current I in the negative z−direction . The magnetic vector field →B at a point having coordinates (x,y) in the Z=0 plane is
View Solution
Knowledge Check
A long straight wire in the horizontal plane carries as curret of 75 A in north to south direction, magnitude and direction of field B at a point 3 m east of the wire is
A4×10−6T, vertical upB5×10−6T, vertical downC5×10−6T, vertical upD4×10−6T, vertical downIf a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
A5.34×10−5TB8.34×10−5TC9.6×10−5TD10.2×10−5T
A long straight wire is kept along x-axis. It carries a current i in the positive x-direction. A proton and an electron are placed at (0, a,0) and (0, -a, 0) respecitively. The proton is imparted an initial velocity v along +z axis and the electron is imparted an initial velocity v along +x-axis. The magnetic forces experienced by the two particles at the instant are
View SolutionConsider a system of two long coaxial cylinders: solid (of radius a carrying i current in positive z direction) and hollow (or radius b carrying current i in negative z direction). Find the magnetic field at a point distant r from of the cylinders for
(i) r≤a
(ii) a<r<b
(iii) r>b

View SolutionAn infinitely long, noc-conducting cylidner of radius R lies along the z-axis. Five long, conducting wires are parallel to the cylinder and spaced equally on the upperhalf of its surface. Each wire carries a current I in the opposite z-direction. Find the magnetic field on the z-axis. opposite z-direction. Find the magnetic field on the z-axis.
View SolutionA long, straight wire carries a current along the z-axis. One can find two points in the x-y plane such that
View SolutionTwo long straight wires, each carrying a current I in opposite directions are separated by a distance R. The magnetic induction at a point mid way between the wire is
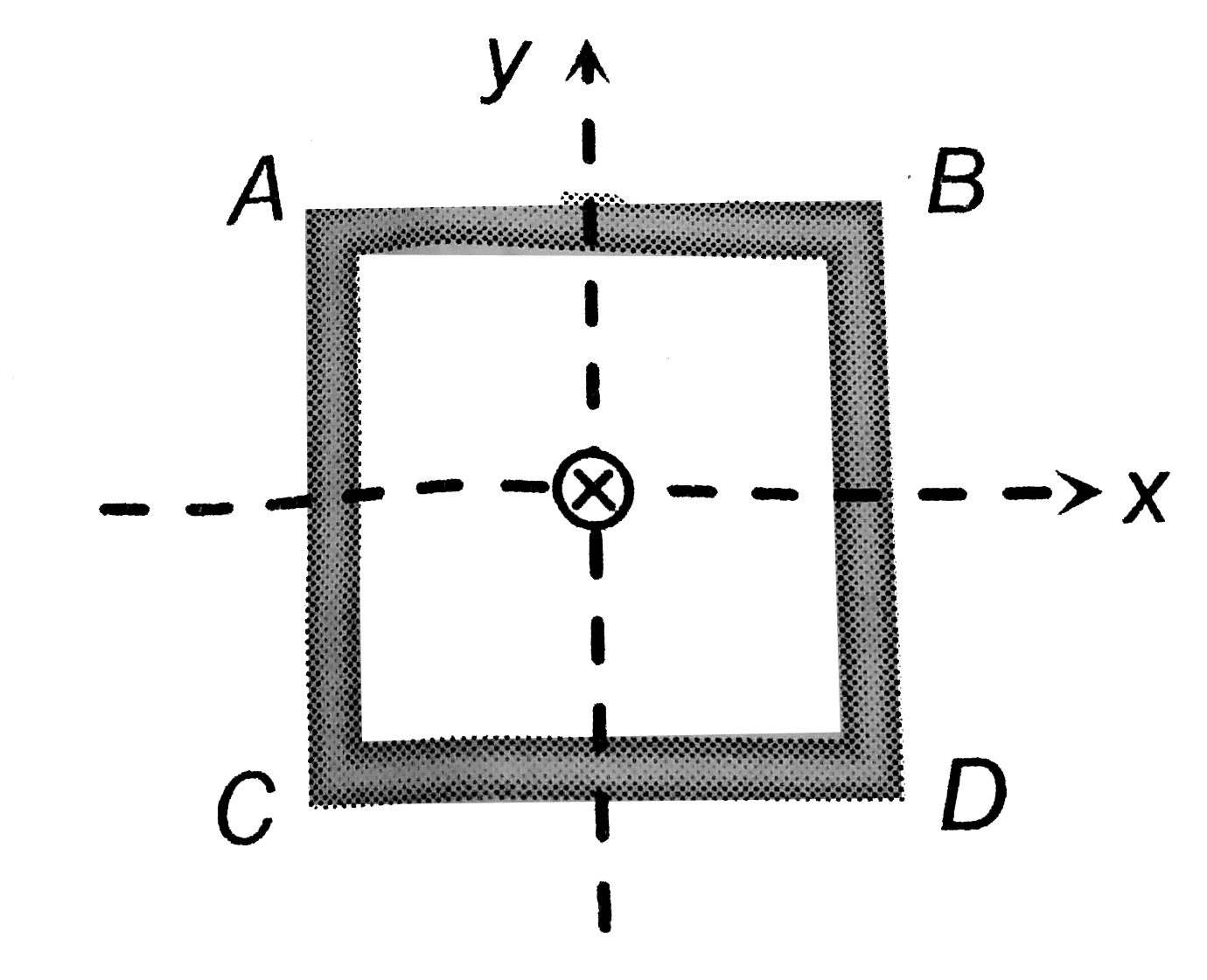
View SolutionA square coil ABCD lying in x−y plane with its centre at origin. A long straight wire passing through origin carries a current i=2t in negative z-direction. The induced current in the coil is
View Solution
