A travelling wave on a string is given by y=Asin[αx+βt+π6]. If α=0.56/cm,β=12/sec,A=7.5cm, then find the position and velocity of particle at x=1cm and t=1s is
4.6cm,46.5cms−1
3.75cm,77.94cms−1
1.76cm,7.5cms−1
7.5cm,75cms−1
The correct Answer is:B
To solve the problem, we need to find the position and velocity of a particle on a string described by the wave equation:
y=Asin(αx+βt+π6)
where:
- α=0.56cm−1
- β=12s−1
- A=7.5cm
We will evaluate this at x=1cm and t=1s.
Step 1: Calculate the position y
1. Substitute the values into the wave equation:
y=7.5sin(0.56⋅1+12⋅1+π6)
2. Calculate the argument of the sine function:
0.56⋅1+12⋅1+π6=0.56+12+π6
- Convert π6 to a decimal (approximately 0.5236):
0.56+12+0.5236≈12.56
3. Now, calculate y:
y=7.5sin(12.56)
4. Recognize that 12.56 can be simplified:
12.56=4π+π6
- Using the periodic property of sine:
sin(4π+π6)=sin(π6)=12
5. Substitute back to find y:
y=7.5⋅12=3.75cm
Step 2: Calculate the velocity v
1. The velocity of the particle is given by the derivative of the displacement with respect to time:
v=dydt=Addt(sin(αx+βt+π6))
2. Using the chain rule:
v=Acos(αx+βt+π6)⋅β
3. Substitute the values:
v=7.5cos(0.56⋅1+12⋅1+π6)⋅12
4. We already calculated the argument:
0.56+12+π6≈12.56
5. Calculate v:
v=7.5cos(12.56)⋅12
6. Recognize that:
cos(12.56)=cos(4π+π6)=cos(π6)=√32
7. Substitute back to find v:
v=7.5⋅√32⋅12
v=7.5⋅6⋅√3=45√3
- Using √3≈1.732:
v≈45⋅1.732≈77.86cm/s
Final Results
- The position of the particle at x=1cm and t=1s is y=3.75cm.
- The velocity of the particle at the same point is v≈77.86cm/s.
- α=0.56cm−1
- β=12s−1
- A=7.5cm
y=7.5sin(0.56⋅1+12⋅1+π6)
0.56⋅1+12⋅1+π6=0.56+12+π6
- Convert π6 to a decimal (approximately 0.5236):
0.56+12+0.5236≈12.56
y=7.5sin(12.56)
12.56=4π+π6
- Using the periodic property of sine:
sin(4π+π6)=sin(π6)=12
y=7.5⋅12=3.75cm
v=dydt=Addt(sin(αx+βt+π6))
v=Acos(αx+βt+π6)⋅β
v=7.5cos(0.56⋅1+12⋅1+π6)⋅12
0.56+12+π6≈12.56
v=7.5cos(12.56)⋅12
cos(12.56)=cos(4π+π6)=cos(π6)=√32
v=7.5⋅√32⋅12
v=7.5⋅6⋅√3=45√3
- Using √3≈1.732:
v≈45⋅1.732≈77.86cm/s
- The velocity of the particle at the same point is v≈77.86cm/s.
Topper's Solved these Questions
- WAVE ON STRING BOOK - RESONANCE ENGLISHCHAPTER - WAVE ON STRING EXERCISE - Exercise- 2 PART I
21 Videos
- WAVE ON STRING BOOK - RESONANCE ENGLISHCHAPTER - WAVE ON STRING EXERCISE - Exercise- 2 PART II
19 Videos
- WAVE ON STRING BOOK - RESONANCE ENGLISHCHAPTER - WAVE ON STRING EXERCISE - Exercise- 1 PART I
23 Videos
- TRAVELLING WAVESBOOK - RESONANCE ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Exercise- 3 PART I
19 Videos
- WAVE OPTICSBOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Advanced Level Problems
8 Videos
Similar Questions
A transverse wave along a string is given by
y=2sin(2π(3t−x)+π4)
where x and y are in cm and t in second. Find acceleration of a particle located at x = 4 cm at t = 1s.
View SolutionA standing wave on a string is given by y=(4cm)cos[xπ]sin[50πt], where x is in metres and t is in seconds. The velocity of the string section at x=1/3matt=1/5s, is
View SolutionThe equation of a progressive wave travelling along a string is given by
y=10sinπ(0.01x−2.00t)
where x and y are in centimetres and t in seconds. Find the (a) velocity of a particle at x=2 m and t=5/6 s. (b) acceleration of a particle at x=1 m and t=1/4 s. also find the velocity amplitude and acceleration amplitude for the wave.
View SolutionA stationary wave is given by y=5sin(πx)3cos40πt where x and y are in cm and t is in seconds
What is the velocity of a particle of the string at the position x = 1.5 cm when t=98s
View SolutionThe equation for a wave travelling in x-direction 0n a string is
y=(3.0cm)sin[(3.14cm−1x−(314s−1t]
(a) Find the maximum velocity of a particle of the string.
(b) Find the acceleration of a particle at x=6.0cm at time t=0.11s
View SolutionThe equation for a wave travelling in x-direction on a string is y =(3.0cm)sin[(3.14cm−1x−(314s−1)t] (a) Find the maximum velocity of a particle of the string. (b) Find the acceleration of a particle at x =6.0 cm at time t = 0.11 s.
View SolutionA travelling wave in a string is represented by y=3sin(π2t−π4x). The phase difference between two particles separated by a distance 4 cm is ( Take x and y in cm and t in seconds )
View SolutionThe wave funcation for a travelling wave on a string in given as
y(x,t)=(0.350m)sin(10πt−3πx+π4)
(a) What are the speed and direction of travel of the wave ?
(b) What is the vertical displacement of the string at t=0,x=0.1m ?
View SolutionThe equation of a wave travelling on a string is given by Y(mn) = 8 sin[(5m−1x−(4s−1t]. Then
View SolutionConsider a sinusoidal travelling wave shown in figure. The wave velocity is +40cm/s.
Find
(a) the frequency
(b) the phase difference between points 2.5cm apart
(c) the velocity of a particle at P at the instant shown.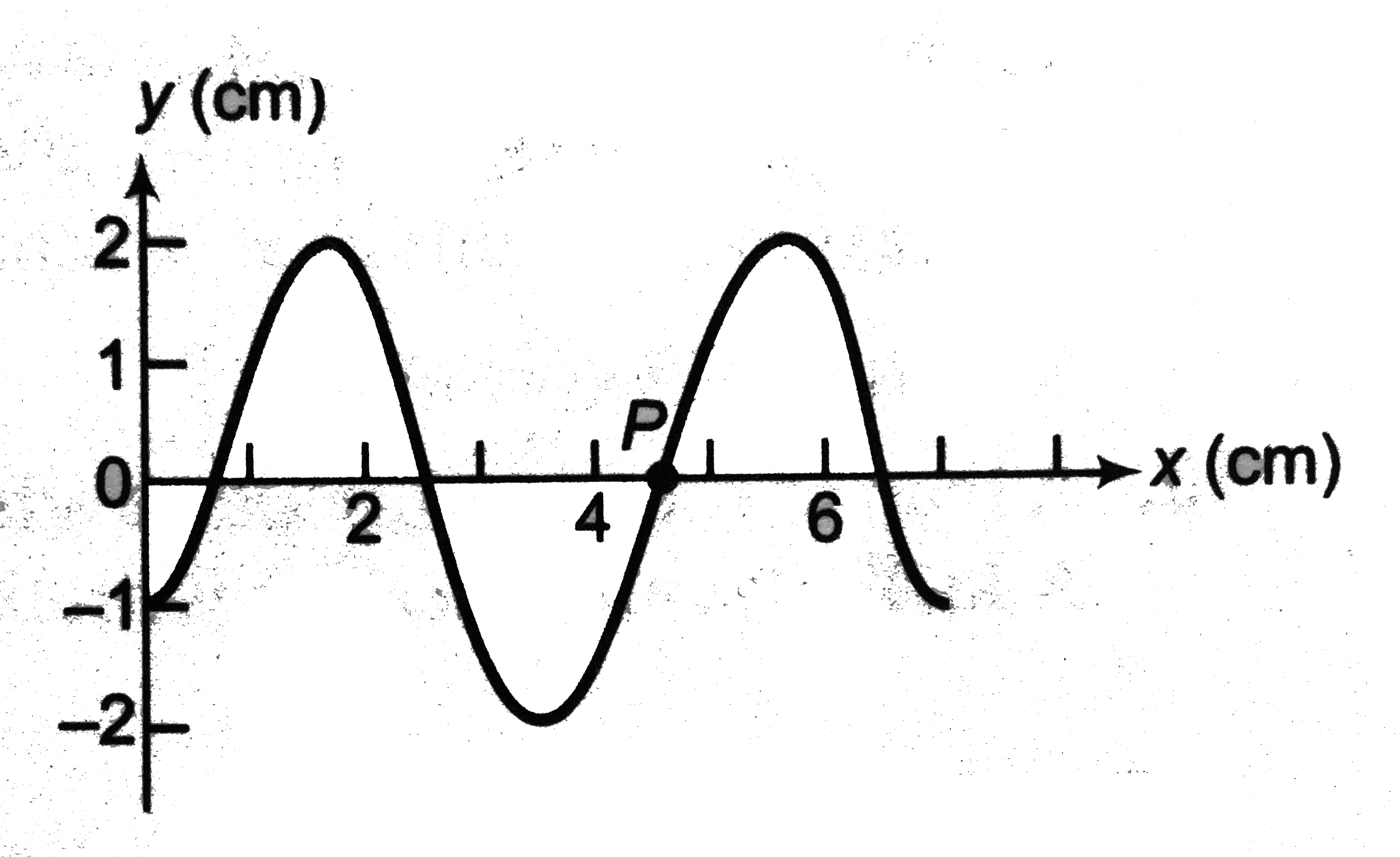
View Solution
