A particle of mass 'm' moves on a horizont smooth line AB of length 'a' such that when particle is at any general point P on the line two force act on it. A force mg(AP)a towards A and another force 2mg(BP)a towards B.
If the force acting towards A stops acting when the particle is nearest to B then find the velocity with which it crosses point B.
√2ga3
√2ga6
√2ga5
√ga3
The correct Answer is:B
To solve the problem, we need to analyze the forces acting on the particle and how they contribute to its motion. Let's break it down step by step.
Step 1: Understand the Forces Acting on the Particle
When the particle is at a point P on the line AB:
- The force acting towards point A is given by FA=mg(AP)a.
- The force acting towards point B is given by FB=2mg(BP)a.
Here, AP is the distance from point A to point P, and BP is the distance from point B to point P.
Step 2: Express the Forces in Terms of Position
Let x be the distance from point A to point P. Therefore:
- AP=x
- BP=a−x
Now, we can rewrite the forces:
- FA=mgxa
- FB=2mg(a−x)a
Step 3: Find the Net Force Acting on the Particle
The net force F acting on the particle at point P is:
F=FB−FA=2mg(a−x)a−mgxa
F=2mg(a−x)−mgxa=2mga−3mgxa
F=mg(2a−3x)a
Step 4: Relate Force to Acceleration
Using Newton's second law, F=ma, we can relate the net force to acceleration:
ma=mg(2a−3x)a
Dividing both sides by m:
a=g(2a−3x)a
Step 5: Identify the Motion Type
The equation we derived resembles the equation of simple harmonic motion (SHM):
a=−ω2x
Comparing, we can identify:
ω2=3ga
Thus, ω=√3ga.
Step 6: Find the Time Period of the Motion
The time period T of the SHM can be calculated as:
T=2π√a3g
Step 7: Calculate the Maximum Velocity
The maximum velocity vmax in SHM is given by:
vmax=ωA
where A is the amplitude of the motion. The amplitude can be determined from the initial conditions.
Assuming the particle starts from rest at point A and moves towards B, the maximum displacement from the equilibrium position (which is at 2a3) can be taken as A=a3.
Thus,
vmax=√3ga⋅a3=√3ga3=√ga
Step 8: Find the Velocity at Point B
When the force towards A stops acting as the particle approaches point B, the velocity at point B can be calculated using energy conservation principles or directly from the maximum velocity derived above.
Thus, the velocity with which the particle crosses point B is:
vB=√2ga6=√ga3
Final Answer
The velocity with which the particle crosses point B is:
vB=√2ga6=√ga3
When the particle is at a point P on the line AB:
- The force acting towards point A is given by FA=mg(AP)a.
- The force acting towards point B is given by FB=2mg(BP)a.
Let x be the distance from point A to point P. Therefore:
- AP=x
- BP=a−x
- FA=mgxa
- FB=2mg(a−x)a
The net force F acting on the particle at point P is:
F=FB−FA=2mg(a−x)a−mgxa
F=2mg(a−x)−mgxa=2mga−3mgxa
F=mg(2a−3x)a
Using Newton's second law, F=ma, we can relate the net force to acceleration:
ma=mg(2a−3x)a
Dividing both sides by m:
a=g(2a−3x)a
The equation we derived resembles the equation of simple harmonic motion (SHM):
a=−ω2x
Comparing, we can identify:
ω2=3ga
Thus, ω=√3ga.
The time period T of the SHM can be calculated as:
T=2π√a3g
The maximum velocity vmax in SHM is given by:
vmax=ωA
where A is the amplitude of the motion. The amplitude can be determined from the initial conditions.
vmax=√3ga⋅a3=√3ga3=√ga
When the force towards A stops acting as the particle approaches point B, the velocity at point B can be calculated using energy conservation principles or directly from the maximum velocity derived above.
vB=√2ga6=√ga3
The velocity with which the particle crosses point B is:
vB=√2ga6=√ga3
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 3, PART - I
20 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 3, PART - II
17 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - III
12 Videos
- SEMICONDUCTORSBOOK - RESONANCE ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS
784 Videos
Similar Questions
A particle of mass 'm' moves on a horizont smooth line AB of length 'a' such that when particle is at any general point P on the line two force act on it. A force mg(AP)a towards A and another force 2mg(BP)a towards B.
Find its time period when released from rest from mid-point of line AB.
View SolutionA particle of mass 'm' moves on a horizont smooth line AB of length 'a' such that when particle is at any general point P on the line two force act on it. A force mg(AP)a towards A and another force 2mg(BP)a towards B. particle released from rest from mid point of AB.
Find the minimum distance of the particle from B during the motion.
View SolutionA particle of mass m is acted upon by a force F=t2−kx . Initially , the particle is at rest at the origin. Then
View SolutionA particle of mass 1 g moves on a straight line. The variation of its velocity with time is shown in fig. Find the force acting on the particle att = 1 s,4s and 7 s.
View SolutionA particle of mass m moves on positive x-axis under the influence of force acting towards the origin given by −kx2ˆi. If the particle starts from rest at x=a, the speed it will attain when it crosses the origin is
View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionA particle of mass m moves from rest under the action of a constant force F which acts for two seconds. The maximum power attained is
View SolutionA particle is placed at the origin of the coordinate system. Two forces of magnitude 20N&10N act on it as shown in figure. It is found that it starts moving towards the point (1,1) . The net unknown force acting on the particle at this position can be :
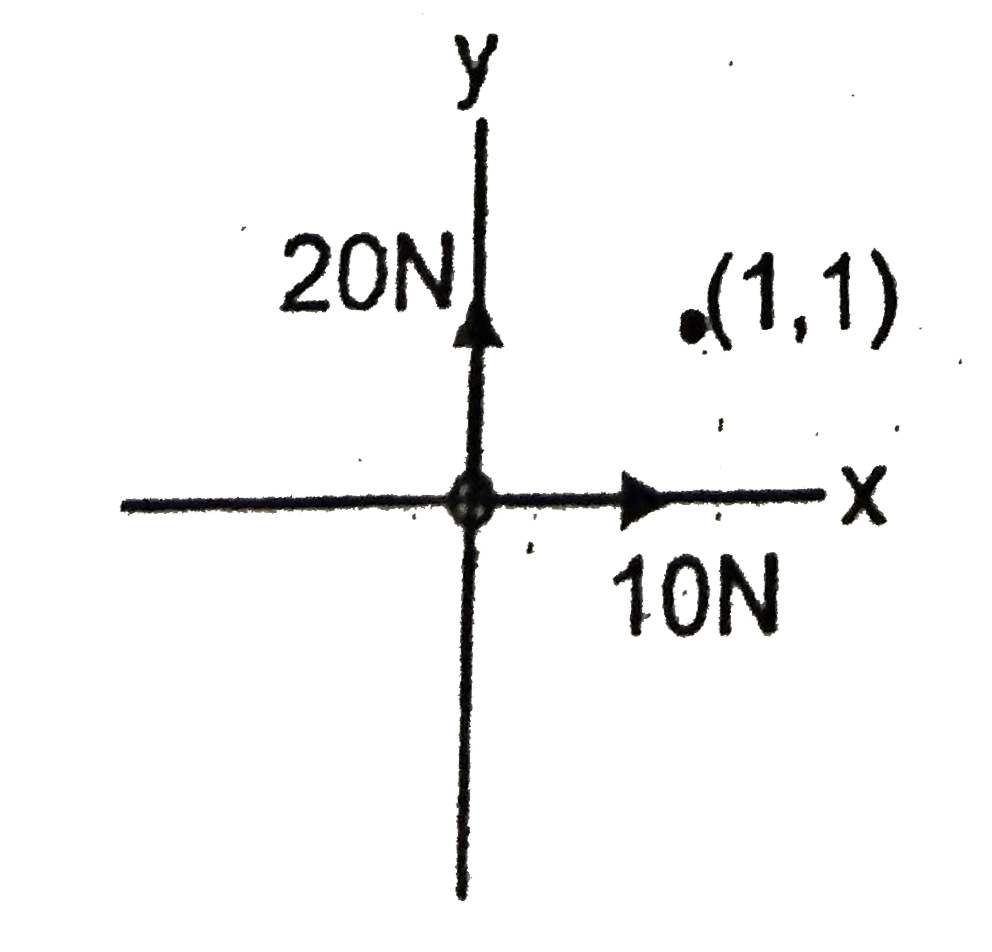
View SolutionA particle of mass 1 kg is moving in SHM with path length 0.01 m and a frequency of 50 Hz. The maximum force in newton, acting on the particle is
View SolutionA force P acts on a particle so as to accelerate it from rest to certain velocity. If P is replaced then by Q which decelerates it to rest.
View Solution
