Only those atoms which four covalent bonds produce a repetitive three dimesional structure using only covalent bonds., e.g. , diamond structure.The latter is based on a FCC lattice where lattice points are occupied by carbon atoms.Every atom in this structure is surrounded tetrahedrally by four others.Germanium, silicon and grey tin also crystallize in the same way as diamond. (Given :NA=6×1023sin54∘44'=0.8164)
If edge length of the cube is 3.60Å, then radius of carbon atom is
0.78Å
0.92Å
0.64Å
0.35Å
The correct Answer is:A
To solve the problem of finding the radius of a carbon atom in a diamond structure with a given edge length of the cube, we can follow these steps:
Step 1: Understand the Structure
The diamond structure is based on a face-centered cubic (FCC) lattice. In this structure, each carbon atom is tetrahedrally coordinated, meaning each carbon atom is surrounded by four other carbon atoms.
Step 2: Identify the Relationship Between Edge Length and Atomic Radius
In an FCC lattice, the relationship between the edge length (a) of the cube and the radius (R) of the atoms can be derived from the body diagonal of the cube. The body diagonal of a cube can be expressed as:
Body Diagonal=√3×a
In the FCC structure, the body diagonal is equal to 4 times the radius of the atoms at the corners:
Body Diagonal=4R
Step 3: Set Up the Equation
From the above relationships, we can equate the two expressions for the body diagonal:
√3×a=4R
Rearranging this gives us:
R=√3×a4
Step 4: Substitute the Given Edge Length
We are given that the edge length (a) of the cube is 3.60 Å. Now, we can substitute this value into the equation:
R=√3×3.604
Step 5: Calculate the Radius
First, calculate √3:
√3≈1.732
Now substituting this value:
R=1.732×3.604
Calculating the numerator:
1.732×3.60≈6.2272
Now divide by 4:
R≈6.22724≈1.5568 Å
Step 6: Round the Answer
Rounding to two decimal places, we find:
R≈1.56 Å
Final Answer
The radius of the carbon atom in the diamond structure is approximately 1.56 Å.
---
The diamond structure is based on a face-centered cubic (FCC) lattice. In this structure, each carbon atom is tetrahedrally coordinated, meaning each carbon atom is surrounded by four other carbon atoms.
In an FCC lattice, the relationship between the edge length (a) of the cube and the radius (R) of the atoms can be derived from the body diagonal of the cube. The body diagonal of a cube can be expressed as:
Body Diagonal=√3×a
In the FCC structure, the body diagonal is equal to 4 times the radius of the atoms at the corners:
Body Diagonal=4R
From the above relationships, we can equate the two expressions for the body diagonal:
√3×a=4R
Rearranging this gives us:
R=√3×a4
We are given that the edge length (a) of the cube is 3.60 Å. Now, we can substitute this value into the equation:
R=√3×3.604
First, calculate √3:
√3≈1.732
Now substituting this value:
R=1.732×3.604
Calculating the numerator:
1.732×3.60≈6.2272
Now divide by 4:
R≈6.22724≈1.5568 Å
Rounding to two decimal places, we find:
R≈1.56 Å
The radius of the carbon atom in the diamond structure is approximately 1.56 Å.
Topper's Solved these Questions
Similar Questions
Only those atoms which four covalent bonds produce a repetitive three dimesional structure using only covalent bonds., e.g. , diamond structure.The latter is based on a FCC lattice where lattice points are occupied by carbon atoms.Every atom in this structure is surrounded tetrahedrally by four others.Germanium, silicon and grey tin also crystallize in the same way as diamond. (Given :NA=6×1023sin54∘44'=0.8164)
If the edge length is 3.60Å, density of diamond crystal is
View SolutionIn a covalent solid the lattice points are occupied by
View SolutionIn diamond structure ,carbon atoms form fcc lattic and 50% tetrahedral voids occupied by acrbon atoms . Evergy carbon atoms is surrounded tetrachedral by four carbon atom with bond length 154 pm . Germanium , silicon and grey tin also crystallise in same way as diamond (NA=6×1023)
The mass of diamond unit cell is:
View SolutionIn diamond structure ,carbon atoms form fcc lattic and 50% tetrahedral voids occupied by acrbon atoms . Evergy carbon atoms is surrounded tetrachedral by four carbon atom with bond length 154 pm . Germanium , silicon and tin also crystallise in same way as diamond (NA=6×1023)
The side length of diamond unit cell is (in pm):
View SolutionIn diamond structure ,carbon atoms form fcc lattic and 50% tetrahedral voids occupied by acrbon atoms . Evergy carbon atoms is surrounded tetrachedral by four carbon atom with bond length 154 pm . Germanium , silicon and grey tin also crystallise in same way as diamond (NA=6×1023)
The side length of diamond unit cell (in pm):
a. 154
b. 1422.63
c. 711.32
d. 355.66
View SolutionPercentage of tetrahedral voids occupied by carbon atoms per unit cell of diamond is
View SolutionTetrahedral voids is surrounded by four atoms. Which atoms in the given figure are around the tetrahedral voids ?
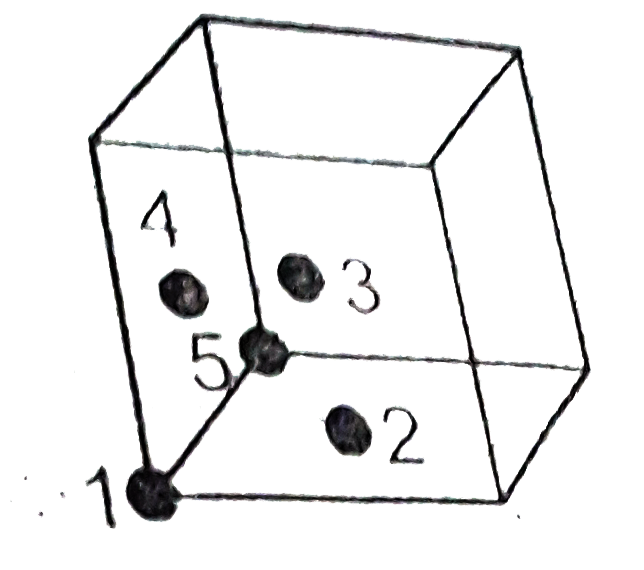
View SolutionNumber of octahedral void(s) occupied by carbon atoms in the unit cell of diamond is
View SolutionIn a diamond, carbon atom occupy fcc lattice points as well as alternate tetrahedral voids. If edge length of the unit cell is 356pm, then diameter of carbon atom is:
View SolutionIn a diamond, each carbon atom is bonded to four other carbon atoms tetrahedrally. Alternate tetrahedral voids are occupied by carbon atoms. The number of carbon atoms per unit cell is:
View Solution