For the third resonance, which option shows correct mode shaoe for displacement variation and pressure variation.




To solve the question regarding the correct mode shape for displacement variation and pressure variation for the third resonance, we can follow these steps:
1. Understanding Resonance in Open and Closed Ends:
- In a tube that is open at one end and closed at the other, the open end will have a displacement antinode (maximum displacement) and a pressure node (minimum pressure).
- Conversely, at the closed end, there will be a displacement node (minimum displacement) and a pressure antinode (maximum pressure).
2. Identifying the Third Resonance:
- For the third resonance in a tube, we need to visualize the distribution of nodes and antinodes.
- The pattern for the third harmonic (or third resonance) will consist of 3 nodes and 2 antinodes.
3. Drawing the Mode Shapes:
- At the open end, we will have:
- Displacement: Antinode (maximum)
- Pressure: Node (minimum)
- At the closed end, we will have:
- Displacement: Node (minimum)
- Pressure: Antinode (maximum)
4. Counting Nodes and Antinodes:
- For the third resonance, we should have:
- 3 nodes and 2 antinodes in total.
- The arrangement will look like this:
- Open end: Antinode (displacement) and Node (pressure)
- Closed end: Node (displacement) and Antinode (pressure)
5. Evaluating the Options:
- We need to check the given options to see which ones correctly depict this arrangement of nodes and antinodes.
- We will look for options that show:
- 3 nodes and 2 antinodes with the correct placement of displacement and pressure variations.
6. Conclusion:
- After evaluating the options, we find that options 2 and 4 show the correct mode shapes for displacement and pressure variations for the third resonance.
Final Answer:
- The correct options for the third resonance are Option 2 and Option 4.
- In a tube that is open at one end and closed at the other, the open end will have a displacement antinode (maximum displacement) and a pressure node (minimum pressure).
- Conversely, at the closed end, there will be a displacement node (minimum displacement) and a pressure antinode (maximum pressure).
- For the third resonance in a tube, we need to visualize the distribution of nodes and antinodes.
- The pattern for the third harmonic (or third resonance) will consist of 3 nodes and 2 antinodes.
- At the open end, we will have:
- Displacement: Antinode (maximum)
- Pressure: Node (minimum)
- At the closed end, we will have:
- Displacement: Node (minimum)
- Pressure: Antinode (maximum)
- For the third resonance, we should have:
- 3 nodes and 2 antinodes in total.
- The arrangement will look like this:
- Open end: Antinode (displacement) and Node (pressure)
- Closed end: Node (displacement) and Antinode (pressure)
- We need to check the given options to see which ones correctly depict this arrangement of nodes and antinodes.
- We will look for options that show:
- 3 nodes and 2 antinodes with the correct placement of displacement and pressure variations.
- After evaluating the options, we find that options 2 and 4 show the correct mode shapes for displacement and pressure variations for the third resonance.
- The correct options for the third resonance are Option 2 and Option 4.
Topper's Solved these Questions
- EXPERIMENTAL PHYSICSBook:RESONANCE ENGLISHChapter:EXPERIMENTAL PHYSICSExercise:Exercise
Explore 12 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCE ENGLISHChapter:EXPERIMENTAL PHYSICSExercise:Exercise -1 PART
Explore 1 Video
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:HLP
Explore 40 Videos
- GEOMATRICAL OPTICS Book:RESONANCE ENGLISHChapter:GEOMATRICAL OPTICS Exercise:Advance level Problems
Explore 35 Videos
Similar Questions
For the second overtone which option shows correct mode for displacement y and pressure variation ΔP
View SolutionWhich of the following is incorrect about Darwinian variations?
View SolutionAn open organ pipe of length L vibrates in its second harmonic mode. The correct statement about the Pressure variation is
View SolutionThe given curve represents the variation of temperature as a function of volume for one mole of an ideal gas. Which of the following curves best represents the variation of pressure as a function of volume?
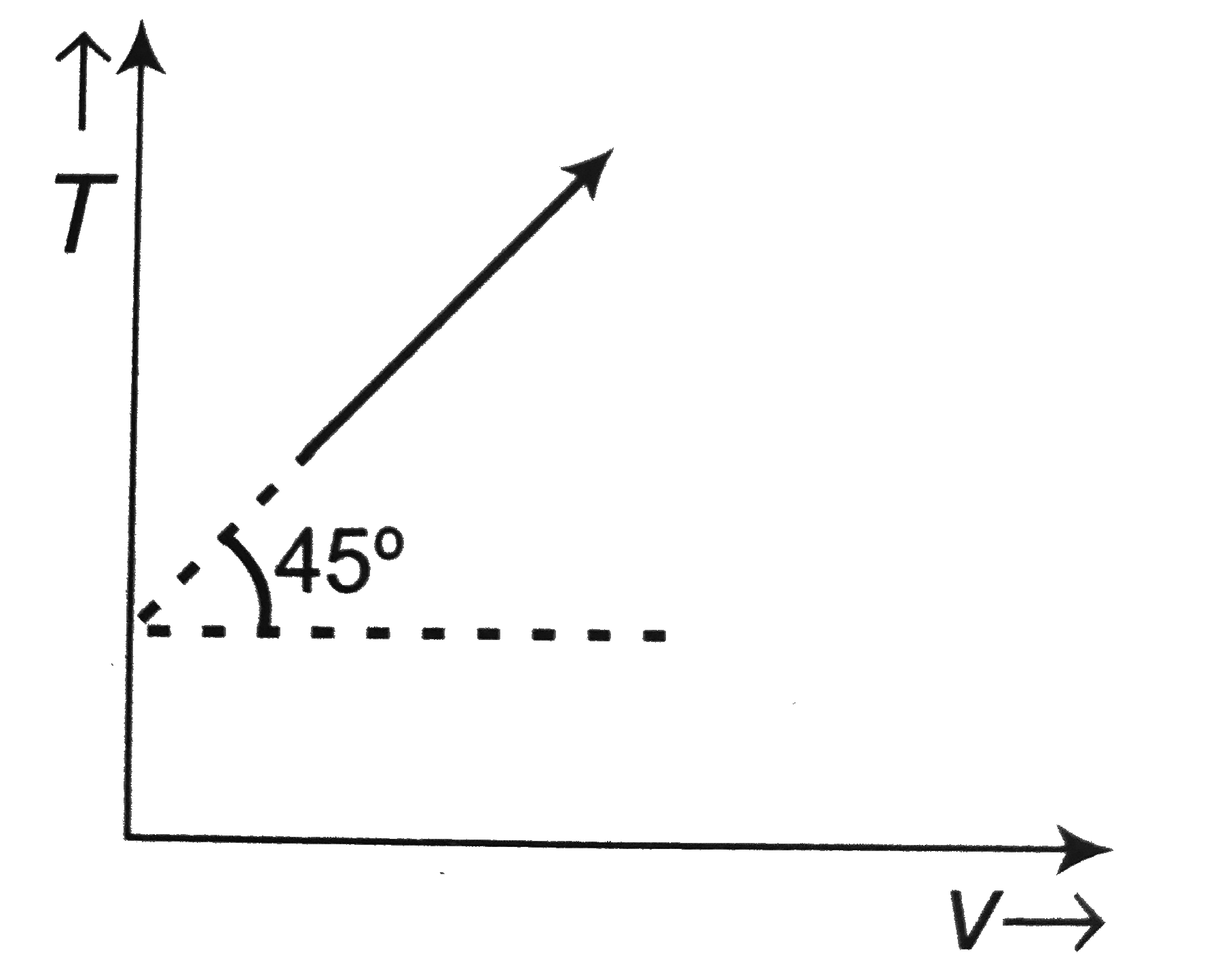
View SolutionThe given curve represents the variation of temperatue as a function of volume for one mole of an ideal gas. Which of the following curves best represents the variation of pressure as a function of volume ?
 .
.
View SolutionThe given curve represents the variation of temperatue as a function of volume for one mole of an ideal gas. Which of the following curves best represents the variation of pressure as a function of volume ?
 .
.
View SolutionThe given curve represents the variation of temperature as a function of volume for one mole of an ideal gas. Which of the following curves best represents the variation of pressure as a function of volume?

View SolutionWhich mutation/ variation is not heredity
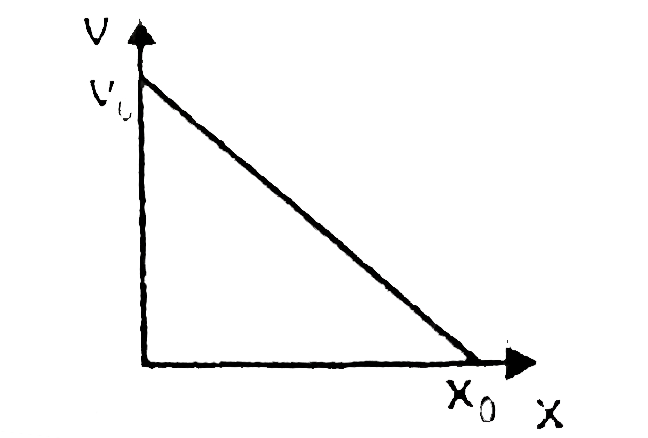
View SolutionThe given graph shows the variation of velocity with displacement. Which one of the graph given below correctly represents the variation of acceleration with displacement :-
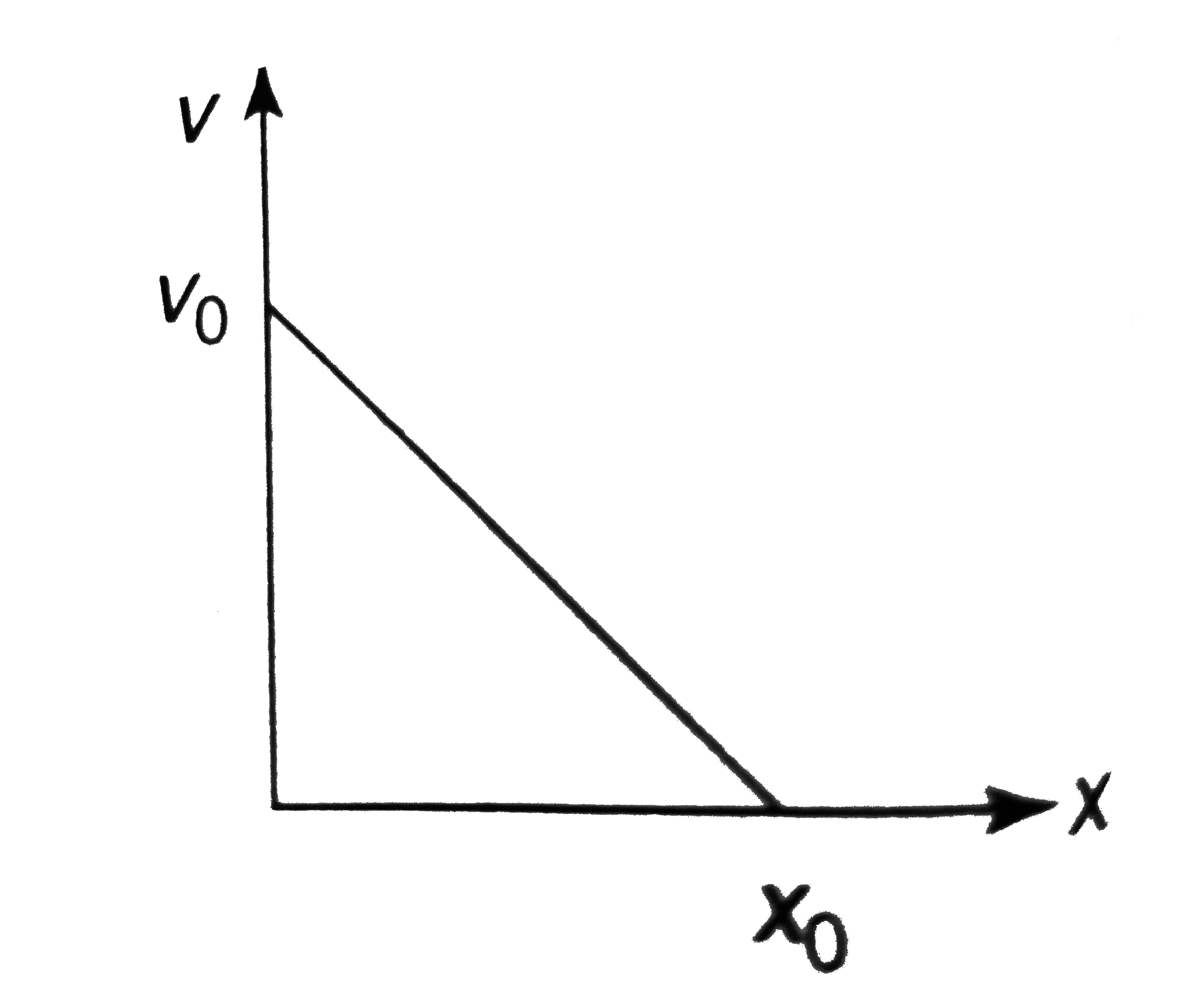
View SolutionThe given graph shows the variation of velocity with displacement. Which one of the graphs given below correctly represents the variation of acceleration with displacement ?
View Solution
