Source and observer start moving simulatneously along x and y-axis respectively. The speed of source is twice the speed of observer V0. If the ratio of observer frequency to the frequency of the source is 0.75, find the velocity of sound.


The correct Answer is:B
To solve the problem, we need to determine the velocity of sound given the conditions of the source and observer's motion and the ratio of their frequencies. Here’s a step-by-step breakdown of the solution:
Step 1: Define the Variables
Let:
- V0 = speed of the observer
- Vs = speed of the source = 2V0
- v = velocity of sound
- n = actual frequency of the source
- na = apparent frequency observed
Step 2: Calculate the Distances
In time t:
- Distance moved by the observer along the y-axis:
y=V0⋅t
- Distance moved by the source along the x-axis:
x=Vs⋅t=2V0⋅t
Step 3: Determine the Angles
Using the distances moved, we can find the hypotenuse of the triangle formed by the movements of the source and observer:
Hypotenuse=√(2V0t)2+(V0t)2=√4V20t2+V20t2=√5V20t2=V0t√5
Step 4: Calculate the Cosines of the Angles
- For the angle α (source's angle):
cosα=2V0t√5V0t=2√5
- For the angle β (observer's angle):
cosβ=V0t√5V0t=1√5
Step 5: Apply the Doppler Effect Formula
The ratio of the apparent frequency to the actual frequency is given by:
nan=v−V0cosβv+Vscosα
Substituting Vs=2V0:
nan=v−V0⋅1√5v+2V0⋅2√5
Step 6: Substitute Known Values
Given that nan=0.75:
0.75=v−V0√5v+4V0√5
This can be rewritten as:
34=v−V0√5v+4V0√5
Step 7: Cross Multiply and Solve for v
Cross multiplying gives:
3(v+4V0√5)=4(v−V0√5)
Expanding both sides:
3v+12V0√5=4v−4V0√5
Rearranging gives:
v=16V0√5
Final Answer
The velocity of sound v is:
v=16V0√5
Let:
- V0 = speed of the observer
- Vs = speed of the source = 2V0
- v = velocity of sound
- n = actual frequency of the source
- na = apparent frequency observed
In time t:
- Distance moved by the observer along the y-axis:
y=V0⋅t
- Distance moved by the source along the x-axis:
x=Vs⋅t=2V0⋅t
Using the distances moved, we can find the hypotenuse of the triangle formed by the movements of the source and observer:
Hypotenuse=√(2V0t)2+(V0t)2=√4V20t2+V20t2=√5V20t2=V0t√5
- For the angle α (source's angle):
cosα=2V0t√5V0t=2√5
- For the angle β (observer's angle):
cosβ=V0t√5V0t=1√5
The ratio of the apparent frequency to the actual frequency is given by:
nan=v−V0cosβv+Vscosα
Substituting Vs=2V0:
nan=v−V0⋅1√5v+2V0⋅2√5
Given that nan=0.75:
0.75=v−V0√5v+4V0√5
This can be rewritten as:
34=v−V0√5v+4V0√5
Cross multiplying gives:
3(v+4V0√5)=4(v−V0√5)
Expanding both sides:
3v+12V0√5=4v−4V0√5
Rearranging gives:
v=16V0√5
The velocity of sound v is:
v=16V0√5
Topper's Solved these Questions
- SOUND WAVESBook:RESONANCE ENGLISHChapter:SOUND WAVESExercise:Exercise- 2 PART - I
Explore 24 Videos
- SOUND WAVESBook:RESONANCE ENGLISHChapter:SOUND WAVESExercise:Exercise- 2 PART - II
Explore 20 Videos
- SOUND WAVESBook:RESONANCE ENGLISHChapter:SOUND WAVESExercise:Exercise- 1 PART - I
Explore 34 Videos
- SIMPLE HARMONIC MOTIONBook:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Exercise
Explore 28 Videos
- STRING WAVESBook:RESONANCE ENGLISHChapter:STRING WAVESExercise:Exercise
Explore 32 Videos
Similar Questions
source and observer both start moving simultaneously from origion one along y - axis with speed of source = 2 (speed of observer ). The graph between the apparent frequency observed by observer (f) and time (t) would be
View Solutionif the speed of a sound source is equal to the speed fo sound and the source is moving away from the observer, then the ratio of apparent frequency to the original frequency is
View Solution
Knowledge Check
At what speed should a source of sound move so that observer finds the apparent frequency equal to half of the original frequency ?
Av2B2vCv/4Dv
If source and observer both are relatively at rest and if speed of sound is increased then frequency heard by observer will
View SolutionA listener and a source of sound are moving with the same speed in the same direction. The ratio of the frequency of the source and the frequency which is heard by the listerner is
View Solution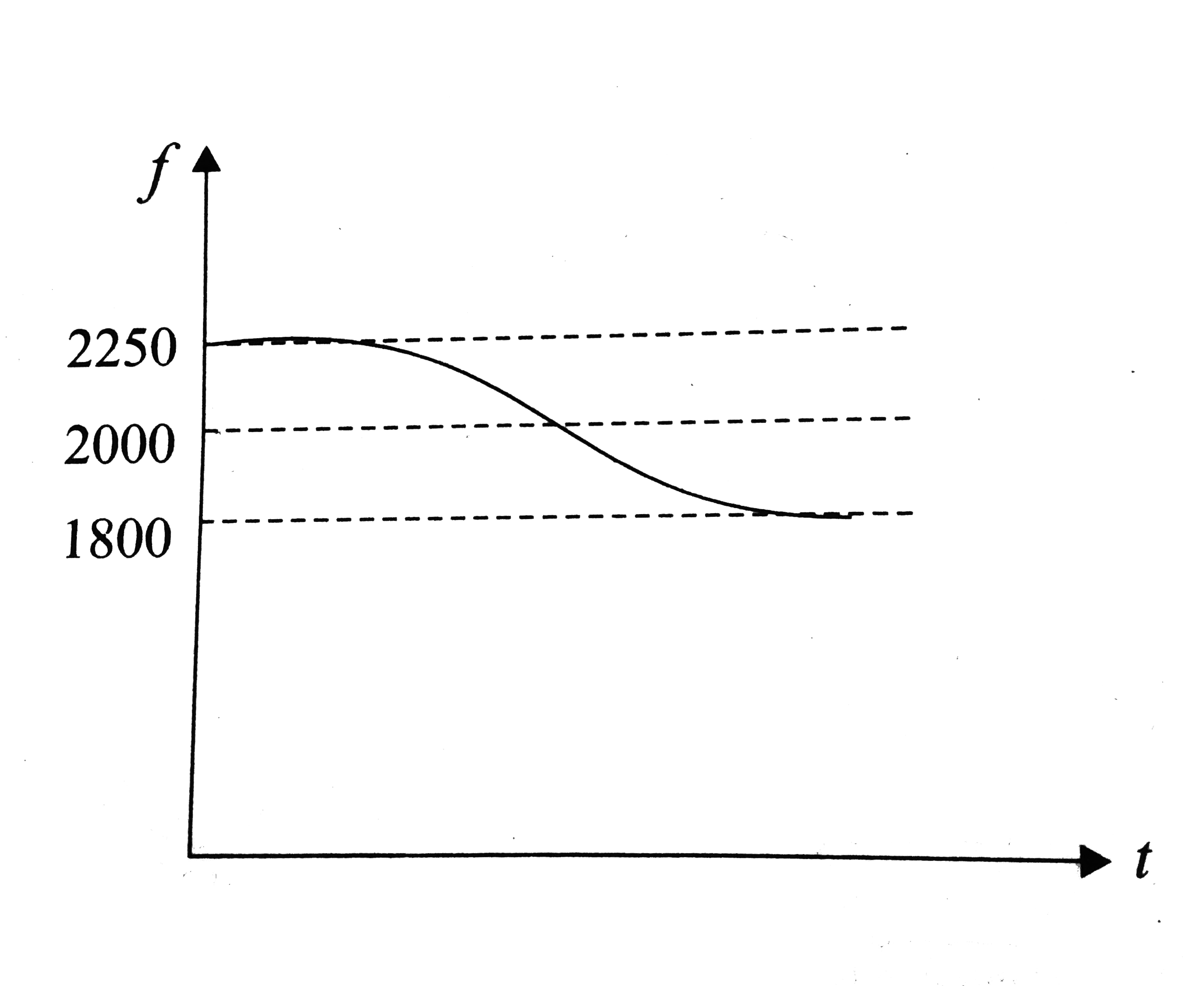
A statinary observer receives a sound of frequency 2000 Hz. The variation of apparent frequency and time is shown. Find the speed of source, if velocity of sound is 300ms
View SolutionOn the same path, the source and observer are moving such a way that the distance between these two increases with the time. The speeds of source and observer are same and equal to 10ms−1 with respect to the ground while no wind in blowing. The apparent frequency received by observer is 1950Hz then the original frequency must be (the speed of sound in present medium is 340m/s
View SolutionAn observer is moving towards a source with speed 15 m/s. The source is moving with speed 5 m/s in the same direction. Air is blowing with speed 10 m/s from observer to source. If frequency of sound emitted is 325 Hz then find frequency of sound heard by observer (velocity of sound in air = 330 m/s) in Hz .
View SolutionAn observer starts moving with unifrom acceleration a towards a stationary sound soure of frequency fo. As the observer approaches the source ,the apparent frequency f heard by the observer varies with time t as
View SolutionA source of sound is travelling towards a stationary observer. The frequency of sound heard by the observer is 25% more that the actual frequency. If the speed of sound is v, that of the source is
View Solution