Two radioactive materials A & B have decay constant 3λ and 2λ respectively. At t=0 the numbers of nuclei of A and B are 4N0 and 2N0 respectively then,
Their number of radioactive nuclei will be equal at t=ln2λ
Their decay rate will be equals at t=ln4λ
Their decay rate will be equal at t=ln3λ
At t=ln4λ the decay rate of A will be greater than that of B.
The correct Answer is:A, C
To solve the problem, we need to analyze the decay of two radioactive materials A and B with given decay constants and initial quantities.
Given:
- Decay constant of A, λA=3λ
- Decay constant of B, λB=2λ
- Initial number of nuclei of A, N0A=4N0
- Initial number of nuclei of B, N0B=2N0
Step 1: Write the decay equations for both materials
The number of undecayed nuclei at time t can be expressed using the formula:
N(t)=N0e−λt
For material A:
NA(t)=4N0e−3λt
For material B:
NB(t)=2N0e−2λt
Step 2: Set the equations equal to find when the number of nuclei is the same
To find the time t when the number of undecayed nuclei of A equals that of B, we set NA(t)=NB(t):
4N0e−3λt=2N0e−2λt
Step 3: Simplify the equation
Cancel N0 from both sides (assuming N0≠0):
4e−3λt=2e−2λt
Step 4: Rearrange the equation
Divide both sides by 2:
2e−3λt=e−2λt
Step 5: Isolate the exponential terms
Rearranging gives:
2=eλt
Step 6: Take the natural logarithm of both sides
Taking the natural logarithm:
ln(2)=λt
Step 7: Solve for t
Thus, we find:
t=ln(2)λ
Conclusion
At t=ln(2)λ, the number of radioactive nuclei of A and B will be equal.
- Decay constant of A, λA=3λ
- Decay constant of B, λB=2λ
- Initial number of nuclei of A, N0A=4N0
- Initial number of nuclei of B, N0B=2N0
The number of undecayed nuclei at time t can be expressed using the formula:
N(t)=N0e−λt
NA(t)=4N0e−3λt
NB(t)=2N0e−2λt
To find the time t when the number of undecayed nuclei of A equals that of B, we set NA(t)=NB(t):
4N0e−3λt=2N0e−2λt
Cancel N0 from both sides (assuming N0≠0):
4e−3λt=2e−2λt
Divide both sides by 2:
2e−3λt=e−2λt
Rearranging gives:
2=eλt
Taking the natural logarithm:
ln(2)=λt
Thus, we find:
t=ln(2)λ
At t=ln(2)λ, the number of radioactive nuclei of A and B will be equal.
Similar Questions
Two radioactive materials A and B have decay constants 10λandλ, respectively. If initially they have the same number of nuclei, then the ratio of the number of nuclei of A of that of B will be 1/e after a time :
View SolutionNucleus A decays into B with a decay constant λ1 and B further decays into C with a decay constant λ2 . Initially, at t = 0, the number of nuclei of A and B were 3N0 and N0 respectively. If at t = t0 number of nuclei of B becomes constant and equal to 2N0, then
View Solution
Knowledge Check
Two radioactive substance A and B have decay constants 5λ and λ respectively. At t=0 they have the same number of nuclei. The ratio of number of nuclei of nuclei of A to those of B will be (1e)2 after a time interval
A14λB4λC2λD12λTwo radioactive materials X1 and X2 have decay constant 11λ and λ respectively. If initially they have same number of nuclei, then ratio of number of nuclei of X1 to X2 will be 1e after a time
A110λB111λC1110λD19λ
Two radioactive materials X1 and X2 have decay constants 10λ and λ respectively. If initially they have the same number of nuclei, if the ratio of the number of nuclei of X1 to that of X2 will be 1/e after a time n9λ. Find the value of n?
View SolutionTwo radioactive material A and B have decay constants 10λ and λ, respectively. If initially they have a the same number of nuclei, then the ratio of the number of nuclei of A to that of B will be 1/e after a time 1nλ, where n is ___________
View SolutionA stable nuclei C is formed from two radioactive nuclei A and B with decay constant of λ1 and λ2 respectively. Initially, the number of nuclei of A is N0 and that of B is zero. Nuclei B are produced at a constant rate of P. Find the number of the nuclei of C after time t.
View SolutionTwo radioactive substances A and B have decay constants 5λ and λ respectively. At t=0 , they have the same number of nuclei. The ratio of number of nuclei of A of those of B will be (1/e)2 after a time interval
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
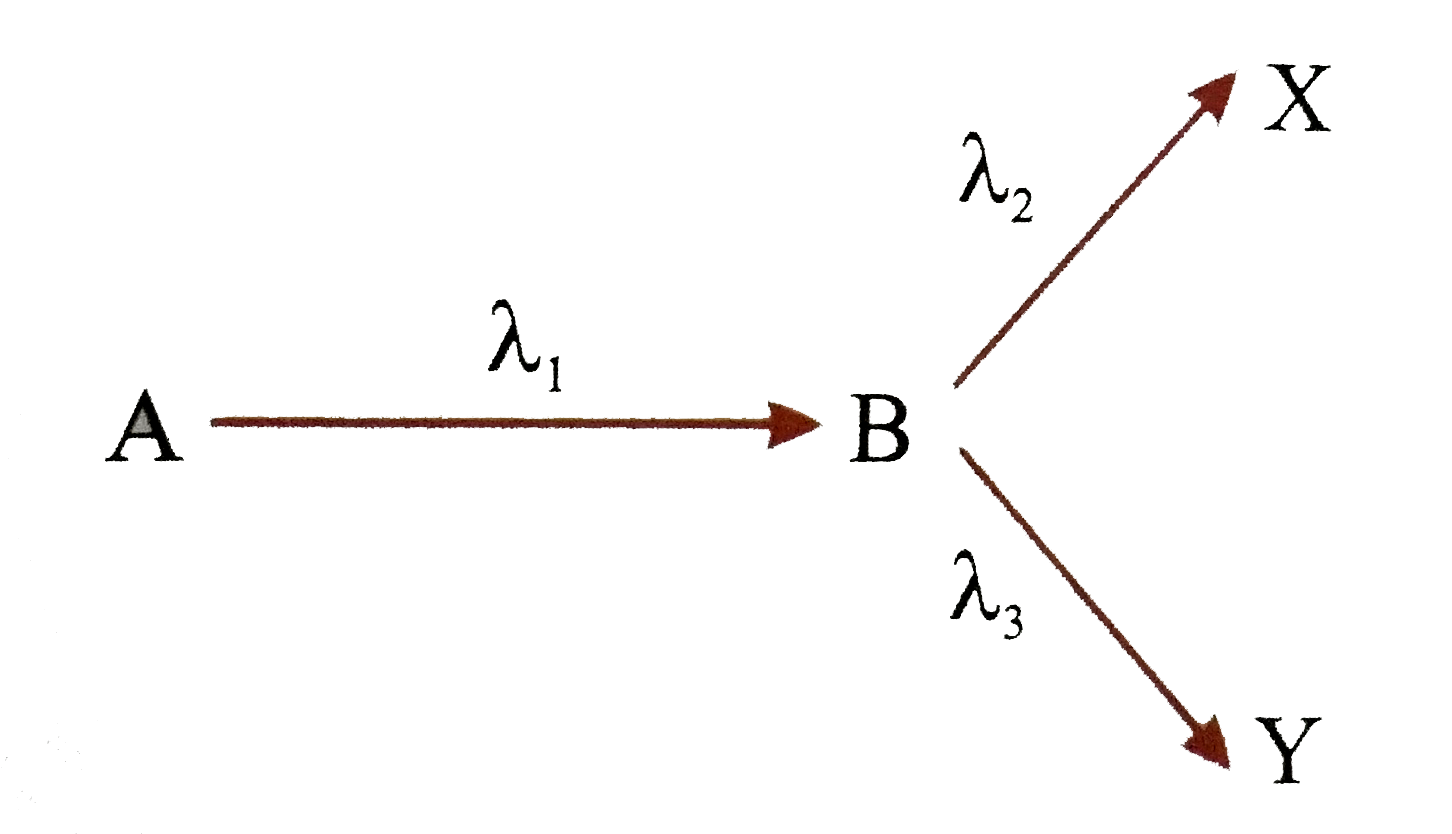
The net rate of accumulation of B at any instant is
View SolutionNucleus A decays to B with decay constant λ1 and B decays to C with decay constant λ2. Initially at t=0 number of nuclei of A and B are 2N0 and N0 respectively. At t=to, no. of nuclei of B is 3N02 and nuclei of B stop changing. Find t0?
View Solution
