
Consider two point charges q1=q2=q(q>0) placed in x-y plane at (0,−R) and (R,0) as shown in the figure. Now charges q2 starts revolving in anticlockwise sense with uniform angular velocity ω in a circle about origin in xy-plane. E is magnitude of net field at origin, V is potential at origin and Ex is x-component of field at origin then select correct variation of V,Ex and E with time t.
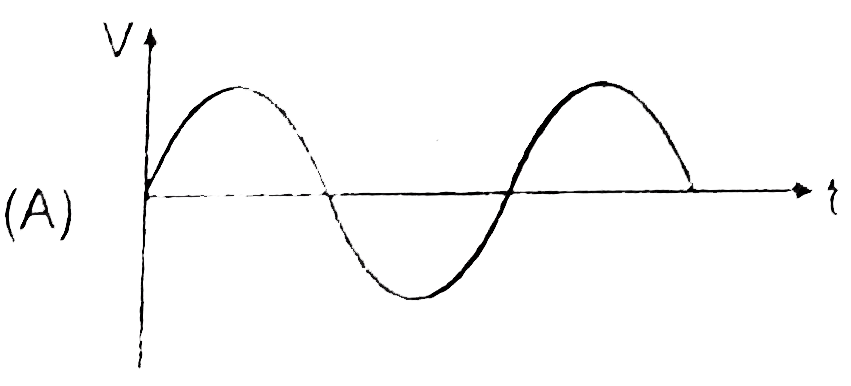
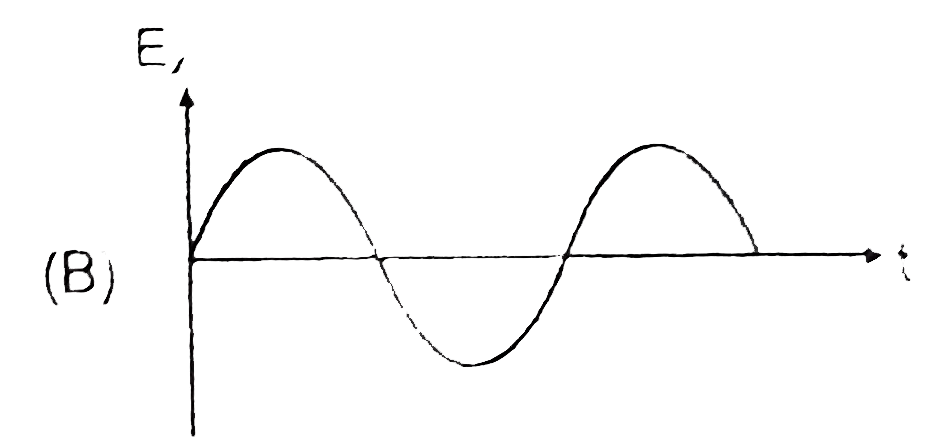
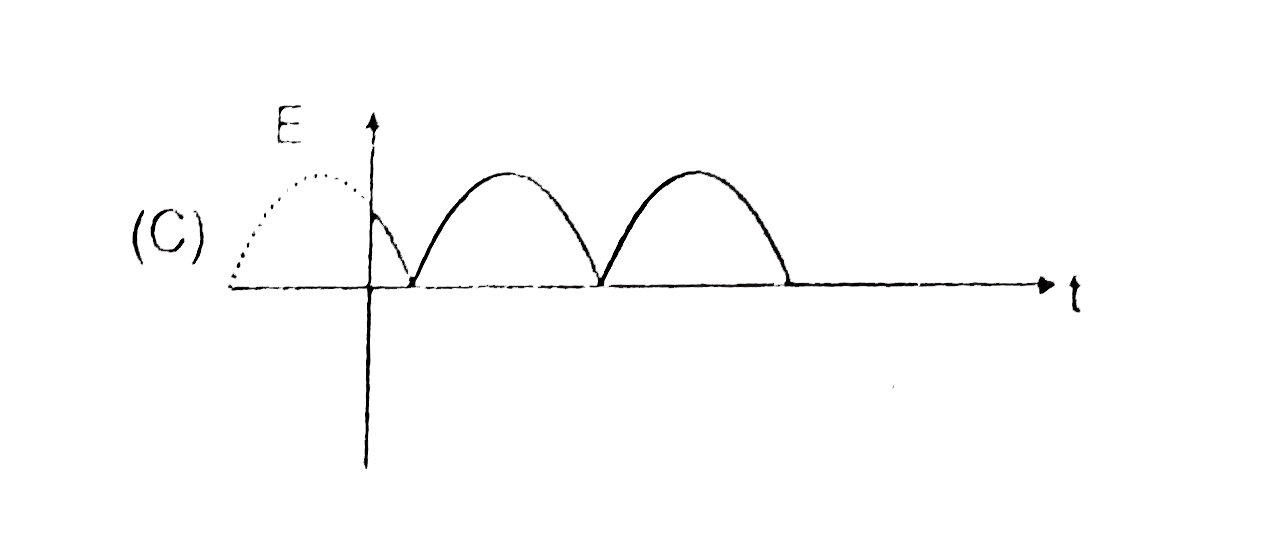

The correct Answer is:C, D
V=2KqR= constant
E≠t=2Kqr2cos(θ2)
E≠t=2Kqr2cos(θ2)
Where θ is angle between electric field due to two charges.
Ex=−KqR2cosωt
Similar Questions
Charge 2qand−q are placed at (a,0)and(−a,0) as shown in the figure. The coordinates of the point at which electric field intensity is zero will be (x,0) where

View SolutionA positively charged ring is in y - z plane with its centre at origin . A positive test charge qe , held at origin is released along x axis, then its speed
View SolutionFour equal point charges Q each are placed in the xy plane at (0,2), (4,2), (4,-2) and (0, -2). The work required to put a fifth charge Q at the origin of the coordinate system will be
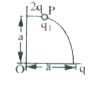
View SolutionTwo point charges q AND 2q are placed at (a,0) and (0,a) A point charge q1 is placed at a P on the quarter circle of radius a as shown in the diagram so that the electric field at the origin becomes zero
View Solution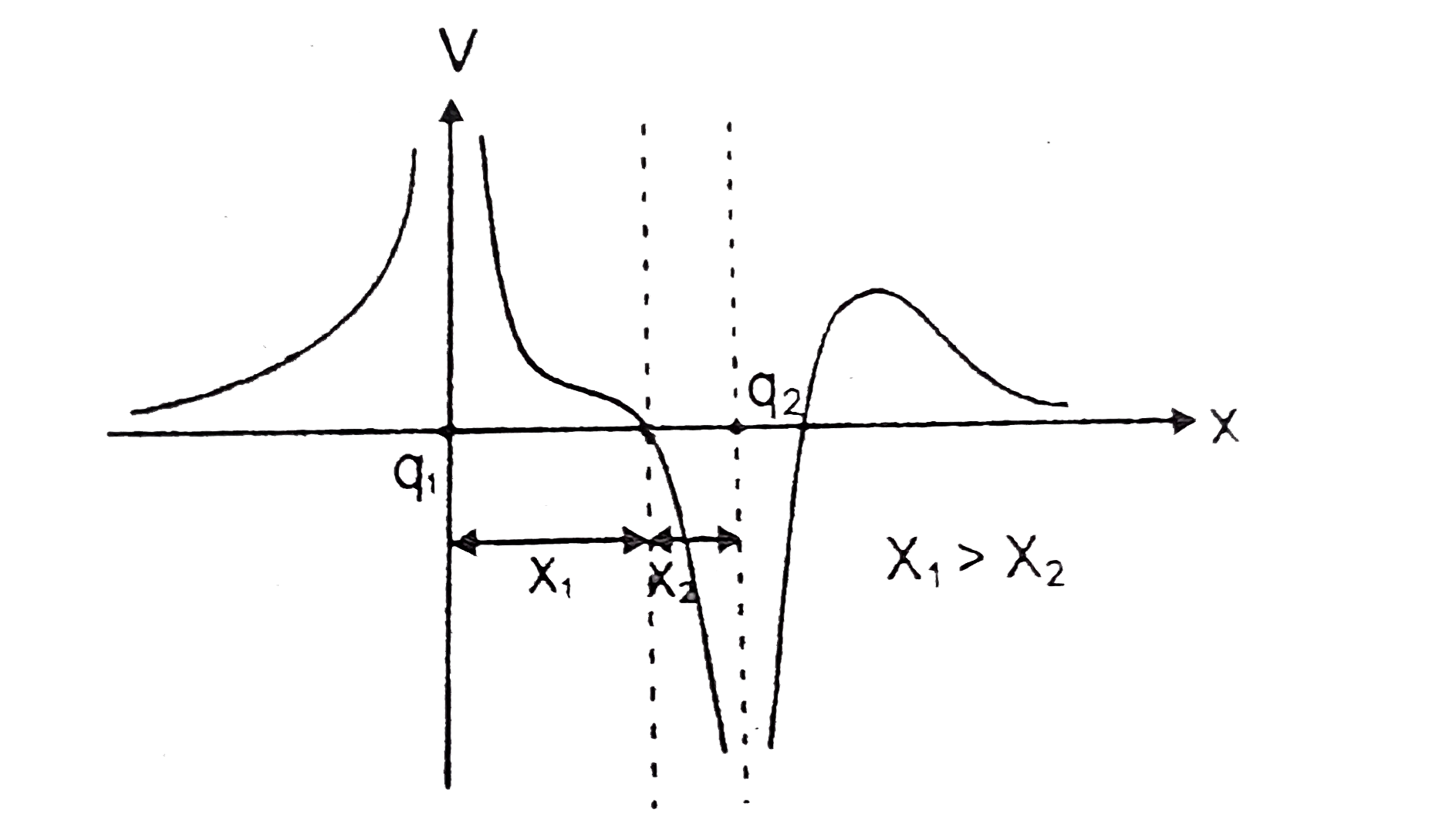
Two point charges (q1 and q2) are placed on x-axis, figure shows graph potential (V) on x-axis. With x-co-ordinate:
View SolutionIn the figure, two point charges +qand−q are placed on the x-axis at (+a,0)and(−a,0) respectively. A tiny dipole of dipolem moment (p) is kept at the origin along the y-axis. The torque on the dipole equals

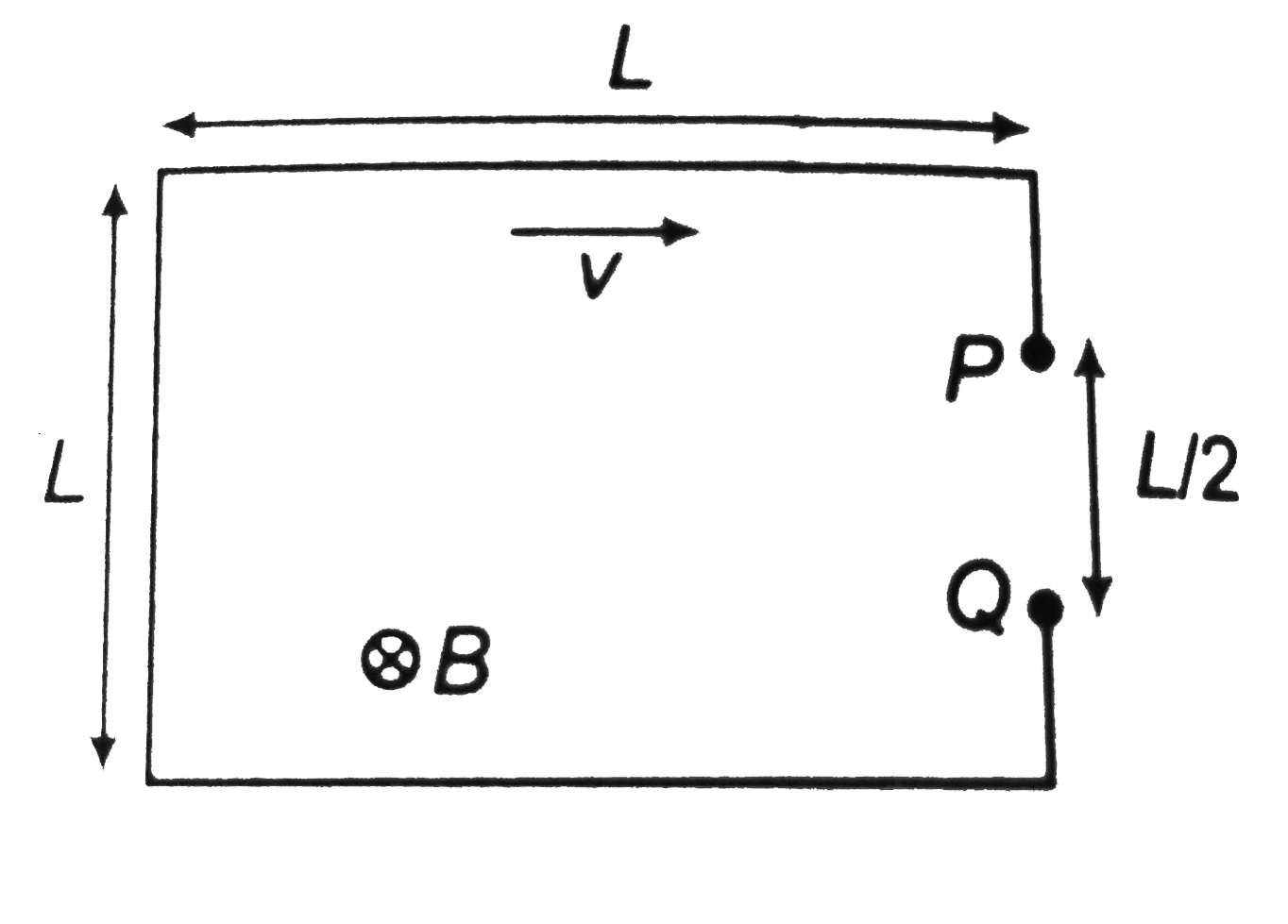
View SolutionThe loop shown moves with a velocity v in a uniform magnetic field of magnitude B, directed into the paper. The potential differene between point P and Q is e. Then
View Solution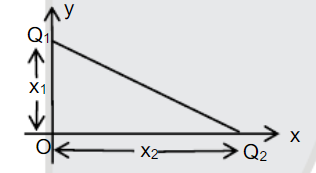
Two charge Q1 and Q2 are placed in xy plane at a distance x1 and x2 from origin a shown in figure. if electric field at O is same for both charge then find ratio Q1 and Q2.
View SolutionA point charge q is placed at origin. Find the flux of electric,produced due to this charge, through a circle of radius R lying in y-z plane. The centre of the circle lies at (x,0).
View SolutionTwo point charge q1=2μC and q2=1μC are placed at distance b=1 and a=2cm from the origin on the y and x axes as shown in figure .The electric field vector at point (a,b) will subtend on angle θ with the "x-axis" given by

View Solution
