n equal cell having emf E and internal resistance r, are connected in circuit of a resistance R. Same current flows in circuit either they connected in series or parallel, if:
R=nr
R=r
The correct Answer is:D
To solve the problem, we will analyze the cases when the cells are connected in series and in parallel, and then find the conditions under which the same current flows in both configurations.
Step 1: Analyze the Series Configuration
- When n cells, each with an EMF E and internal resistance r, are connected in series, the total EMF Eseries is:
Eseries=nE
- The total internal resistance Rinternal,series is:
Rinternal,series=nr
- The total resistance in the circuit Rtotal,series is:
Rtotal,series=R+nr
- The current Iseries flowing through the circuit can be calculated using Ohm's law:
Iseries=EseriesRtotal,series=nER+nr
Step 2: Analyze the Parallel Configuration
- When the same n cells are connected in parallel, the total EMF Eparallel remains the same:
Eparallel=E
- The equivalent internal resistance Rinternal,parallel is given by:
Rinternal,parallel=rn
- The total resistance in the circuit Rtotal,parallel is:
Rtotal,parallel=R+rn
- The current Iparallel flowing through the circuit can be calculated as:
Iparallel=EparallelRtotal,parallel=ER+rn
Step 3: Set the Currents Equal
- According to the problem, the same current flows in both configurations:
Iseries=Iparallel
- Substituting the expressions for current:
nER+nr=ER+rn
Step 4: Simplify the Equation
- Cancel E from both sides (assuming E≠0):
nR+nr=1R+rn
- Cross-multiplying gives:
n(R+rn)=R+nr
- Expanding both sides:
nR+r=R+nr
- Rearranging terms:
nR−R=nr−r
- Factoring out common terms:
(n−1)R=(n−1)r
Step 5: Solve for Resistance
- Assuming n−1≠0 (which is valid for n>1):
R=r
Conclusion
The condition for the same current to flow in both series and parallel configurations is that the resistance R connected in the circuit must equal the internal resistance r of the cells.
- When n cells, each with an EMF E and internal resistance r, are connected in series, the total EMF Eseries is:
Eseries=nE
- The total internal resistance Rinternal,series is:
Rinternal,series=nr
- The total resistance in the circuit Rtotal,series is:
Rtotal,series=R+nr
- The current Iseries flowing through the circuit can be calculated using Ohm's law:
Iseries=EseriesRtotal,series=nER+nr
- When the same n cells are connected in parallel, the total EMF Eparallel remains the same:
Eparallel=E
- The equivalent internal resistance Rinternal,parallel is given by:
Rinternal,parallel=rn
- The total resistance in the circuit Rtotal,parallel is:
Rtotal,parallel=R+rn
- The current Iparallel flowing through the circuit can be calculated as:
Iparallel=EparallelRtotal,parallel=ER+rn
- According to the problem, the same current flows in both configurations:
Iseries=Iparallel
- Substituting the expressions for current:
nER+nr=ER+rn
- Cancel E from both sides (assuming E≠0):
nR+nr=1R+rn
- Cross-multiplying gives:
n(R+rn)=R+nr
- Expanding both sides:
nR+r=R+nr
- Rearranging terms:
nR−R=nr−r
- Factoring out common terms:
(n−1)R=(n−1)r
- Assuming n−1≠0 (which is valid for n>1):
R=r
The condition for the same current to flow in both series and parallel configurations is that the resistance R connected in the circuit must equal the internal resistance r of the cells.
Topper's Solved these Questions
Similar Questions
Four cells, each of emf E and internal resistance r, are connected in series across an external resistance R. By mistake one of the cells is connected in reverse. Then, the current in the external circuit is
View SolutionThere are n cells, each of emf E and internal resistance r, connected in series with an external resistance R. One of the cells is wrongly connected, so that it sends current in the opposite direction. The current flowing in the circuit is
View Solution
Knowledge Check
A battery having 12V emf and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 1A, then the resistance of resistor and lost voltage of the battery when circuit is closed will be
A7Ω,7VB8Ω,8VC9Ω,9VD9Ω,10VIf n cells each of emf E and internal resistance rare connected in parallel, then the total emf and internal resistances will be
Aε,rnBε,nrCnε,rnDnε,nr
n identical cells each of e.m.f. E and internal resistance r are connected in series. An external resistance R is connected in series to this combination. The current through R is
View Solutionn identical cells, each of emf E and internal resistance r, are joined in series to form a closed circuit. Find the potential difference across any one cell.
View SolutionA circuit consists of a resistance R connected to n similar cells. If the current in the circuit is the same whether the cells are connected in series or in parallel, then the internal resistancer of each cell is given by
View SolutionA cell of emf E and internal resistance r is connected in series with an external resistance nr. Than what will be the ratio of the terminal potential difference to emf, if n=9.
View SolutionTwo cells , each of emfE and internal resistance r, are connected in parallel across a resistor R. The power delivered to the resistor is maximum if R is equal to
View SolutionThree identical cells , each having an emf1.5V and a constant internal resistance 2.0ohm, are connected in series with a 4.0ohm resistor R, first as in circuit (i) , and second as in circuit (ii) . Then
( power in R in circuit (i))/( Power in R in circuit (ii)) =
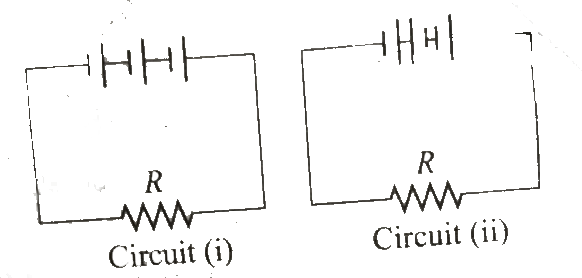
View Solution
