Two particles of mass 1 kg and 0.5 kg are moving in the same direction with speed of 2m/s and 6 m/s respectively on a smooth horizontal surface. The speed of centre of mass of the system is
103m/s
107m/s
112m/s
123m/s
The correct Answer is:A
To find the speed of the center of mass of a system consisting of two particles, we can use the formula for the velocity of the center of mass (V_cm):
Vcm=m1v1+m2v2m1+m2
Where:
- m1 and m2 are the masses of the two particles,
- v1 and v2 are the velocities of the two particles.
Step 1: Identify the given values
- Mass of the first particle, m1=1kg
- Velocity of the first particle, v1=2m/s
- Mass of the second particle, m2=0.5kg
- Velocity of the second particle, v2=6m/s
Step 2: Substitute the values into the formula
Now, we substitute the values into the center of mass velocity formula:
Vcm=(1kg×2m/s)+(0.5kg×6m/s)1kg+0.5kg
Step 3: Calculate the numerator
Calculate the contributions from each mass:
1kg×2m/s=2kg m/s
0.5kg×6m/s=3kg m/s
Adding these together gives:
2kg m/s+3kg m/s=5kg m/s
Step 4: Calculate the denominator
Calculate the total mass:
1kg+0.5kg=1.5kg
Step 5: Compute the speed of the center of mass
Now, substitute the values back into the formula:
Vcm=5kg m/s1.5kg=51.5m/s
To simplify this fraction:
51.5=103m/s
Final Answer
The speed of the center of mass of the system is:
103m/s
- m1 and m2 are the masses of the two particles,
- v1 and v2 are the velocities of the two particles.
- Mass of the first particle, m1=1kg
- Velocity of the first particle, v1=2m/s
- Mass of the second particle, m2=0.5kg
- Velocity of the second particle, v2=6m/s
Now, we substitute the values into the center of mass velocity formula:
Calculate the contributions from each mass:
0.5kg×6m/s=3kg m/s
Calculate the total mass:
Now, substitute the values back into the formula:
The speed of the center of mass of the system is:
Topper's Solved these Questions
Similar Questions
Two particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo particles of masses 2 kg and 4 kg are approaching each other with acceleration 1ms−2 and 2ms−2, respectively, on a smooth horizontal surface. Find the acceleration of center of mass of the system.
View SolutionTwo particles of masses 2kg and 4kg are approaching towards each other with ccelerations 1m/s2 and 2m/s2, respectively, on a smooth horizontal suface. Then find the acceleration of centre of mass of the system and direction of acceleration of CM.
View SolutionTwo blocks of masses m1=2kg and m2=4kg are moving in the same direction with speeds v1=6m/s and v2=3m/s, respectively on a frictionless surface as shown in the figure. An ideal spring with spring constant k=30000N/m is attached to the back side of m2. Then the maximum compression of the spring after collision will be
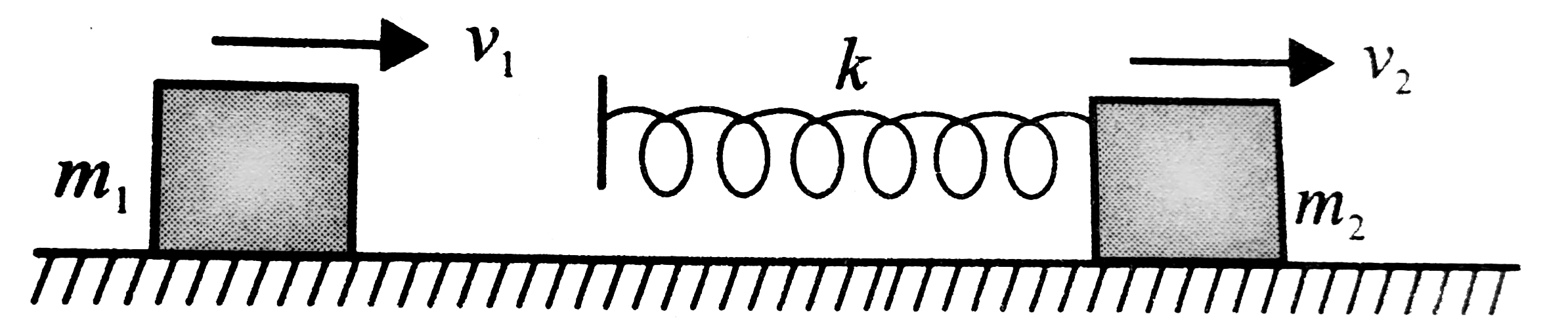
View SolutionTwo particles A and B of masses 2kg and 3kg moving on a smooth horizontal surface with speed 1m/s and 2m/s collides perfectly inelastically, then
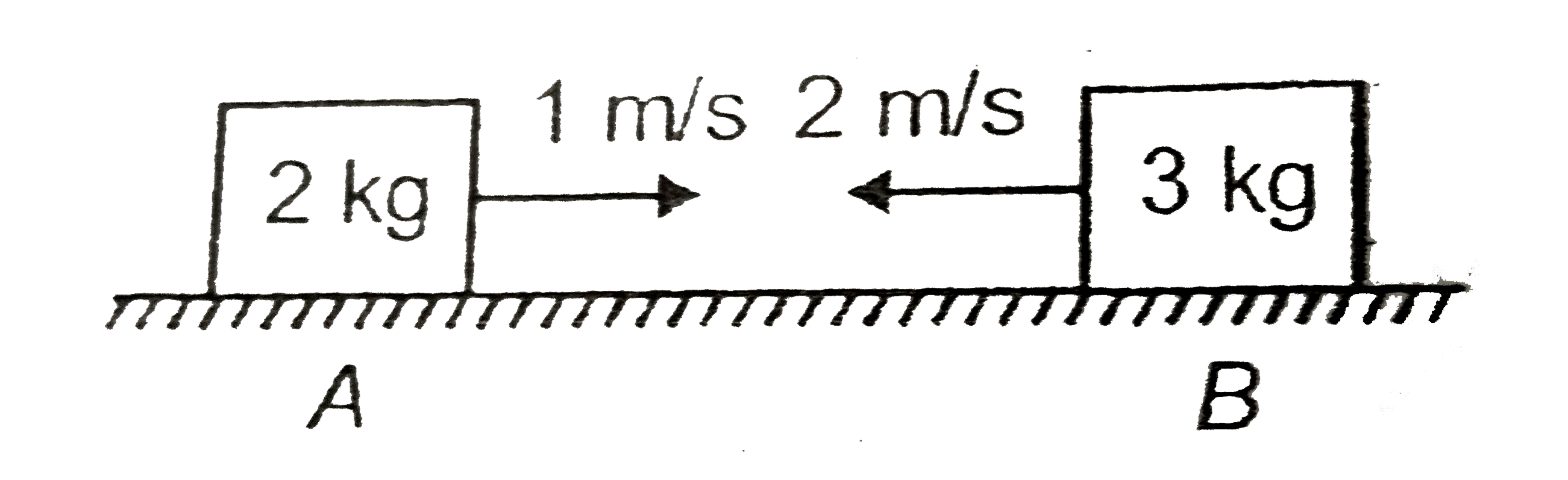
View SolutionThree bodies having masses 5 kg, 4 kg and 2 kg is moving at the speed of 5 m/sand2m//s and 5 m/s respectively along X-axis.The magnitude of velocity of centre of mass is
View SolutionTwo particles of equal mass are moving along the same line with the same speed in the same direction. What is the speed of the centre of mass of the system ?
View SolutionTwo particles of equal mass are moving along the same line with the same speed in the same direction. What is the speed of the centre of mass of the system ?
View SolutionTwo particles of equal mass are moving along the same straight line with the same speed in opposite direction. What is the speed of the centre of mass of the system ?
View Solution
