An object is moving along a straight line path from P to Q under the action of a force →F=(4ˆi−3ˆj+ˆ2k)N. If the co-ordinate of P and Q in meters are (3,2,-1) and (2,-1,4) respectively. Then the work done by the force is
−15J
+15J
1015J
(4ˆi−3ˆj+2ˆk)
The correct Answer is:B
To solve the problem of calculating the work done by the force as the object moves from point P to point Q, we will follow these steps:
Step 1: Identify the coordinates of points P and Q
The coordinates of point P are given as (3, 2, -1) and the coordinates of point Q are (2, -1, 4).
Step 2: Write the force vector
The force vector is given as:
→F=4^i−3^j+2^k N
Step 3: Calculate the displacement vector
The displacement vector →s can be calculated by subtracting the coordinates of point P from point Q:
→s=→Q−→P=(2^i−1^j+4^k)−(3^i+2^j−1^k)
Calculating this gives:
→s=(2−3)^i+(−1−2)^j+(4+1)^k=−1^i−3^j+5^k
Step 4: Calculate the work done
The work done W by the force is given by the dot product of the force vector and the displacement vector:
W=→F⋅→s=(4^i−3^j+2^k)⋅(−1^i−3^j+5^k)
Calculating the dot product:
W=(4)(−1)+(−3)(−3)+(2)(5)=−4+9+10
Thus,
W=15 Joules
Final Answer
The work done by the force is 15 Joules.
---
The coordinates of point P are given as (3, 2, -1) and the coordinates of point Q are (2, -1, 4).
The force vector is given as:
→F=4^i−3^j+2^k N
The displacement vector →s can be calculated by subtracting the coordinates of point P from point Q:
→s=→Q−→P=(2^i−1^j+4^k)−(3^i+2^j−1^k)
Calculating this gives:
→s=(2−3)^i+(−1−2)^j+(4+1)^k=−1^i−3^j+5^k
The work done W by the force is given by the dot product of the force vector and the displacement vector:
W=→F⋅→s=(4^i−3^j+2^k)⋅(−1^i−3^j+5^k)
Calculating the dot product:
W=(4)(−1)+(−3)(−3)+(2)(5)=−4+9+10
Thus,
W=15 Joules
The work done by the force is 15 Joules.
Topper's Solved these Questions
Similar Questions
An object of mass 2 kg at rest at origin starts moving under the action of a force
→F=(3t2ˆi+4tˆj)N The velocity of the object at t = 2 s will be -
View SolutionA particle moves from point P(1,2,3) to (2,1,4) under the action of a constant force F=(2ˆi+ˆj+ˆk)N. Work done by the force is
View SolutionA particle moves from point P(1,2,3) to (2,1,4) under the action of a constant force F=(2ˆi+ˆj+ˆk)N. Work done by the force is
View SolutionA particle moves from point (ˆi+ˆj)m to a point (6ˆi−2ˆj)m under the action of force (10ˆi−2ˆj)N. The work done by the force is
View SolutionA particle moves under the action of a force F=20ˆi+15ˆj along a straight line 3y+αx=5, where, α is a constant. If the work done by the force F is zero the value of α is .
View SolutionIf →PQ=3ˆi+2ˆj−ˆk and the coordinates of P are (1,−1,2), find the coordinates of Q.
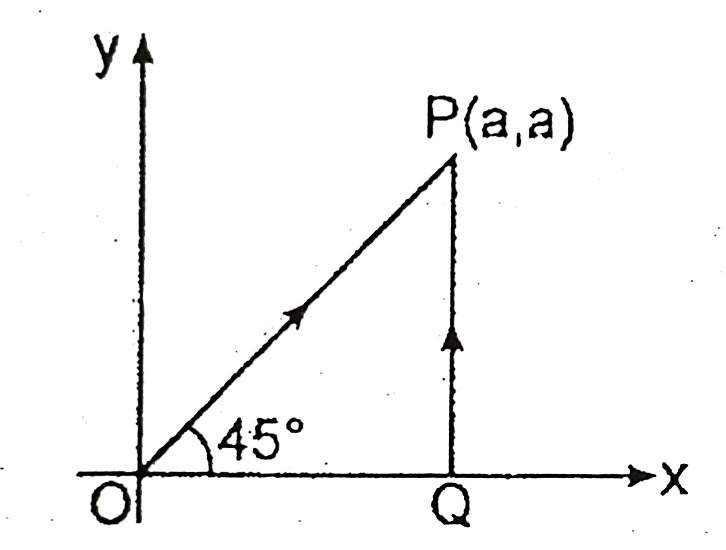
View SolutionA particle is moved from (0, 0) to (a, a) under a force a F=(3ˆi+4ˆj) from two paths. Path 1 is OP and path 2 is OPQ. Let W1 and W2 be the work done by this force in these two paths. Then,
View SolutionA particle is moved from (0,0)→(a,a) under a force F=(x2ˆi+y3ˆj) from two paths. Path 1 si OP and Path 2 is OQP. Let W1 and W2 be the work done by this force in these two paths. Then
View SolutionA force (3ˆi+4ˆj) newton acts on a boby and displaces it by (2ˆi+3ˆj) metre. The work done by the force is
View SolutionAn object is displaced from point A(2m,3m,4m) to a point B(1m,2m,3m) under a constant force F=(2ˆi+3ˆj+4ˆk)N. Find the work done by this force in this process.
View Solution