A bob is attached to one end of a string other end of which is fixed at ped A. The bob is taken to a position where string makes an angle of 30∘ with the horizontal. On the circular path of the bob in vertical plane there is a peg'B' at a symmetrical position with respect to the position of release as shown in the figure. If Vc and Va be the minimum speeds in clockwise and anticlokwise direction respectively, given to the bob in order to hit the peg 'B' then ratio Vc:Va is equal to :

1:1
1:√2
1:2
1:4
The correct Answer is:C
For anti-clockwise motion, speed at the highest point should be √gR Conserving energy at (1) & (2) :

12mv2a=mgR2+12(gR)
⇒V2a=gR+gR=2gR
⇒Va=√2gR
For clock-wise motion, the bob must have atleast that much speed initially, so that the string must not become loose any where until it reaches the peg B
At the initial position
T=mgcos60∘=mv20R
Vcbeing the initial speed in clockwise direction

For Vmin Put T =0 :
⇒V=√gR2
⇒VC/CA=√gR2√2gR=12
⇒V:V1=1:2
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.4320videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.4410videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.419videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)19videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
A particle of mass 200 g , is whirled into a vertical circle of radius 80 cm uisig a massless string The speed of the particle when the string makes an angle of 60∘ with the vertical line is 1.5ms−1. The tension in the string at this position is
View SolutionA stone is attached to one end of a string and rotated in verticle cricle .If string breaks at the position of maximum tension, will break at
 View Solution
View SolutionThe bob A of a simple pendulum is released when the string makes an angle of 45∘ with the vertical. It hits another bob B of the same material and same mass kept at rest on the table. If the collision is elastic, then
 View Solution
View SolutionA bob of mass m=50g is suspended form the ceiling of a trolley by a light inextensible string. If the trolley accelerates horizontally, the string makes an angle 37∘ with the vertical. Find the acceleration of the trolley.
 View Solution
View SolutionOne end of ideal string tied up with fixed suppot & other end is tied with a bob. The string is released from horizontal position. When string makes an angle of θ with vertically downward direction then the acceleration vector of bob is horizontal then θ is
View SolutionA bob hangs from a rigid support by an inextensible string of length l. It is released from rest when string makes an agngle 60∘ with vertical . The speed of the bob at the lowest position is
View SolutionA bob of mass 1 kg is suspended from an inextensible string of length 1 m. When the string makes an angle 60∘ with vertical, speed of the bob is 4 m/s
Upto what maximum height will the bob rise with respect to the bottommost point ?View SolutionA simple pendulum consists of a 50 cm long string connected to a 100 g ball. The ball is pulled aside so that the string makes an angel of 370 with the vertical and is then released. Find the tension in the string when the bob is at its lowest position.
 View Solution
View SolutionThe bob hanging from a light string is projected horizontally with a speed u0. In the subsequent upward motion of the bob in vertically plane
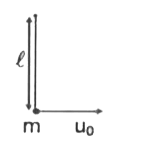 View Solution
View SolutionA pendulum bob has a speed of 3ms−1 at its lowest position. The pendulum is 0.5 m long. The speed of the bob, when string makes an angle of 60∘ to the vertical is (take, g=10ms−1)
View Solution
