A block of mass 20 kg is acted upon by a force F=30N at an angle 53∘ with horizontal in downward direction as shown. The coefficient of friction between the block and the forizontal surface is 0.2. The friction force acting on the block by the ground is (g=10ms2)
40.0N
30.0N
18.0N
44.8N
The correct Answer is:C
To solve the problem, we need to analyze the forces acting on the block and calculate the friction force. Here’s a step-by-step solution:
Step 1: Identify the Forces Acting on the Block
1. Weight of the Block (W): The weight can be calculated using the formula:
W=m⋅g
where m=20kg and g=10m/s2.
W=20kg⋅10m/s2=200N
2. Applied Force (F): The applied force is given as F=30N at an angle of 53∘ downwards.
3. Components of the Applied Force:
- Horizontal Component (Fx):
Fx=F⋅cos(53∘)=30⋅cos(53∘)=30⋅35=18N
- Vertical Component (Fy):
Fy=F⋅sin(53∘)=30⋅sin(53∘)=30⋅45=24N
Step 2: Calculate the Normal Force (N)
The normal force is affected by both the weight of the block and the vertical component of the applied force. The normal force can be calculated as:
N=W+Fy=200N+24N=224N
Step 3: Calculate the Maximum Frictional Force (Fmax)
The maximum frictional force can be calculated using the formula:
Fmax=μ⋅N
where μ=0.2.
Fmax=0.2⋅224N=44.8N
Step 4: Compare Horizontal Force and Maximum Frictional Force
The horizontal force acting on the block is Fx=18N. Since the maximum frictional force is greater than the horizontal force, the frictional force will equal the horizontal force:
Ffriction=Fx=18N
Conclusion
The friction force acting on the block by the ground is 18N.
---
1. Weight of the Block (W): The weight can be calculated using the formula:
W=m⋅g
where m=20kg and g=10m/s2.
W=20kg⋅10m/s2=200N
- Horizontal Component (Fx):
Fx=F⋅cos(53∘)=30⋅cos(53∘)=30⋅35=18N
- Vertical Component (Fy):
Fy=F⋅sin(53∘)=30⋅sin(53∘)=30⋅45=24N
The normal force is affected by both the weight of the block and the vertical component of the applied force. The normal force can be calculated as:
N=W+Fy=200N+24N=224N
The maximum frictional force can be calculated using the formula:
Fmax=μ⋅N
where μ=0.2.
Fmax=0.2⋅224N=44.8N
The horizontal force acting on the block is Fx=18N. Since the maximum frictional force is greater than the horizontal force, the frictional force will equal the horizontal force:
Ffriction=Fx=18N
The friction force acting on the block by the ground is 18N.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.24
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.25
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.22
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
A force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
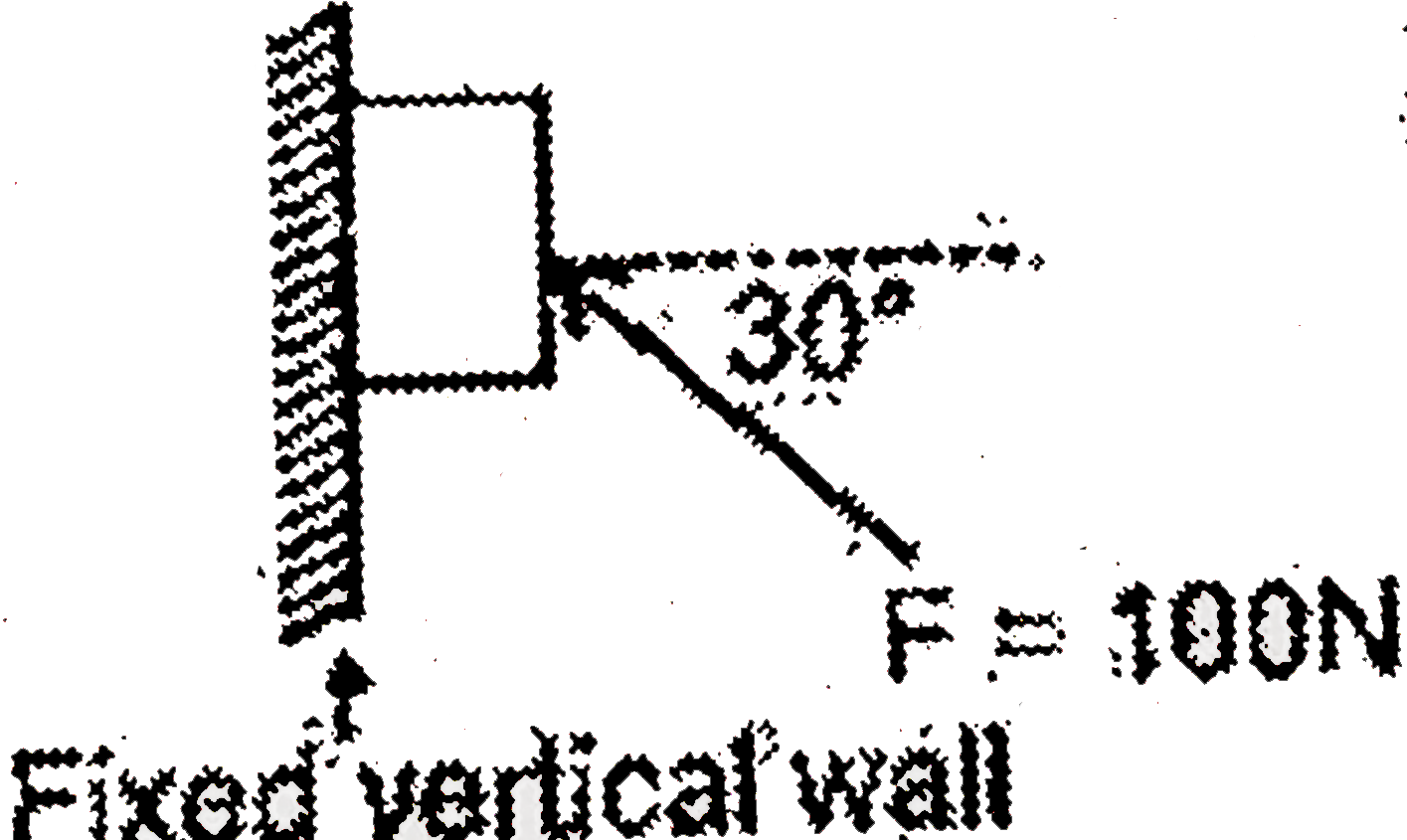
View SolutionA block of mass 8 kg is sliding on a surface inclined at an angle of 45∘ with the horizontal. Calculate the acceleration of the block. The coefficient of friction between the block and surface is 0.6. (Take g=10m/s2)
View Solution
Knowledge Check
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A10.3NB23.8NC11.9ND6.3NA block of mass 3 kg rests on a rough inclined plane making an angle of 30∘. With the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A9.8 NB0.7×9.8×√3 NC9.8×√3 ND0.7×9.8 N
A block of mass 8 kg is sliding on a surface inclined at an angle of 45∘ with the horizontal . Calculate the acceleration of the block . The coefficient of friction between the block and surface is 0⋅6 ( Take g = 10m/s2)
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block of mass 1 kg is placed on a truck which accelerates with acceleration 5ms−2. The coefficient of static friction between the block and truck is 0.6. The frictional force acting on the block is
View SolutionA force of 200N is applied as shown in the figure on block of 3 kg. The coefficient of friction between the block and the wall is 0.3. Find the friction acting on the block. (in N) {Take g = 10 m/s2

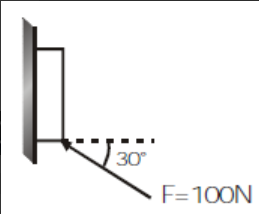
View SolutionA force of 100 N is applied on a block of mass 3 kg as shown in figure. The coefficient of friction between the surface and the block is 14 .Then the friction force acting on the block is
View SolutionA horizontal force of 10 N is necessary to just hold a block stationary against a wall. The coefficient of friction between the block and the wall is 0.2. the weight of the block is (g=10m/s2)

View Solution
