A force →F is applied to block (m=6kg) at rest on an inclined plane of inclination 30∘. The force →F is horizontal and parallel to surface of inclined plane. Maximum value of F so that block remains at rest is 40N. The coefficient of friction of the surface is.
2√3
√3
53√3
5√32
The correct Answer is:C
To find the coefficient of friction (μ) for the block on the inclined plane, we can follow these steps:
Step 1: Identify the Forces Acting on the Block
The forces acting on the block include:
1. The gravitational force (weight) →W=m⋅g
2. The normal force →N
3. The applied horizontal force →F
4. The frictional force →Ff
Step 2: Calculate the Weight of the Block
Given:
- Mass m=6kg
- Acceleration due to gravity g=10m/s2
The weight W of the block is:
W=m⋅g=6kg⋅10m/s2=60N
Step 3: Resolve the Weight into Components
The weight can be resolved into two components:
1. Perpendicular to the inclined plane: W⊥=W⋅cos(θ)=60⋅cos(30∘)
2. Parallel to the inclined plane: W∥=W⋅sin(θ)=60⋅sin(30∘)
Calculating these components:
- cos(30∘)=√32
- sin(30∘)=12
Thus,
W⊥=60⋅√32=30√3N
W∥=60⋅12=30N
Step 4: Apply the Condition for Equilibrium
For the block to remain at rest, the net force acting along the inclined plane must be zero. The forces acting along the incline are:
- The frictional force Ff=μ⋅N
- The component of the weight down the incline W∥
- The applied force F
The equation for equilibrium along the incline is:
F+Ff=W∥
Substituting Ff:
F+μ⋅N=W∥
Step 5: Calculate the Normal Force
The normal force N is equal to the perpendicular component of the weight:
N=W⊥=30√3N
Step 6: Substitute Known Values
Given that the maximum applied force F=40N and substituting N:
40+μ⋅(30√3)=30
Step 7: Solve for the Coefficient of Friction (μ)
Rearranging the equation:
μ⋅(30√3)=30−40
μ⋅(30√3)=−10
μ=−1030√3=−13√3
However, since the frictional force cannot be negative, we need to consider the absolute values and the correct direction of forces. The correct equation should be:
μ⋅(30√3)=40−30
μ⋅(30√3)=10
μ=1030√3=13√3=√39
Final Answer
The coefficient of friction μ is:
μ=13√3≈0.192
The forces acting on the block include:
1. The gravitational force (weight) →W=m⋅g
2. The normal force →N
3. The applied horizontal force →F
4. The frictional force →Ff
Given:
- Mass m=6kg
- Acceleration due to gravity g=10m/s2
W=m⋅g=6kg⋅10m/s2=60N
The weight can be resolved into two components:
1. Perpendicular to the inclined plane: W⊥=W⋅cos(θ)=60⋅cos(30∘)
2. Parallel to the inclined plane: W∥=W⋅sin(θ)=60⋅sin(30∘)
- cos(30∘)=√32
- sin(30∘)=12
W⊥=60⋅√32=30√3N
W∥=60⋅12=30N
For the block to remain at rest, the net force acting along the inclined plane must be zero. The forces acting along the incline are:
- The frictional force Ff=μ⋅N
- The component of the weight down the incline W∥
- The applied force F
F+Ff=W∥
F+μ⋅N=W∥
The normal force N is equal to the perpendicular component of the weight:
N=W⊥=30√3N
Given that the maximum applied force F=40N and substituting N:
40+μ⋅(30√3)=30
Rearranging the equation:
μ⋅(30√3)=30−40
μ⋅(30√3)=−10
μ=−1030√3=−13√3
μ⋅(30√3)=40−30
μ⋅(30√3)=10
μ=1030√3=13√3=√39
The coefficient of friction μ is:
μ=13√3≈0.192
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.24
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.25
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.22
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
A block of mass 10 kg is placed on rough inclined plane of inclination angle 37∘ .Coefficient of friction between block & plane is 0⋅8, find minimum magnitude of F so that block remains at rest .

View SolutionA block of mass 5 kg is at rest on a rough inclined surface. If angle of inclination of plane is 60∘, then force applied by it on block is
View SolutionA horizontal force F is applied to a block of mass m on a smooth fixed inclined plane of inclination θ to the horizontal as shown in the figure. Resultant force on the block up the plane is:

View SolutionA body is placed on a smooth inclined plane of inclination 1 in x. The horizontal acceleration to be given to the inclined plane so that the body on it remains at rest with respect to inclined plane is
View SolutionA block of mass 4kg rests on an an inclined plane. The inclination of the plane is gradually increased. It is found that when the inclination is 3 in 5 (sin=θ35) the block just begins to slide down the plane. The coefficient of friction between the block and the plane is.
View SolutionA block is placed on a smooth inclined plane as shown . For what value of horizontal force F, the block will remain at rest ?
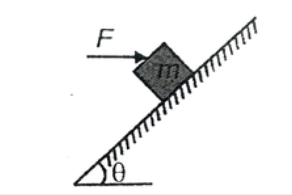
View SolutionA block of mass 2kg is pushed down an inclined plane of angle 37∘ above horizontal with a force parallel to inclined plane. The coefficient of friction is 3/4. The block moves 10m in 2 seconds starting form rest. The magnitude of the force is
View SolutionA block of mass 10 kg is kept on a fixed rough (μ=0.8) inclined plane of angle of inclination 30∘. The frictional force acting on the block is

View SolutionA block of mass 2 kg rests on an inclined plane of inclination angle 37∘ , then calculate the force of friction acting on the block [ take g = 10m/s2)
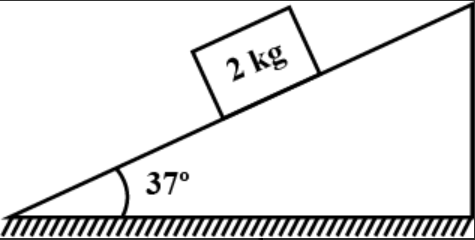
View SolutionFind the minimum value of horizontal force (F) required on the block of mass m to keep it at rest on the wall. Given the coefficient of friction between the surfaces is μ.
View Solution
