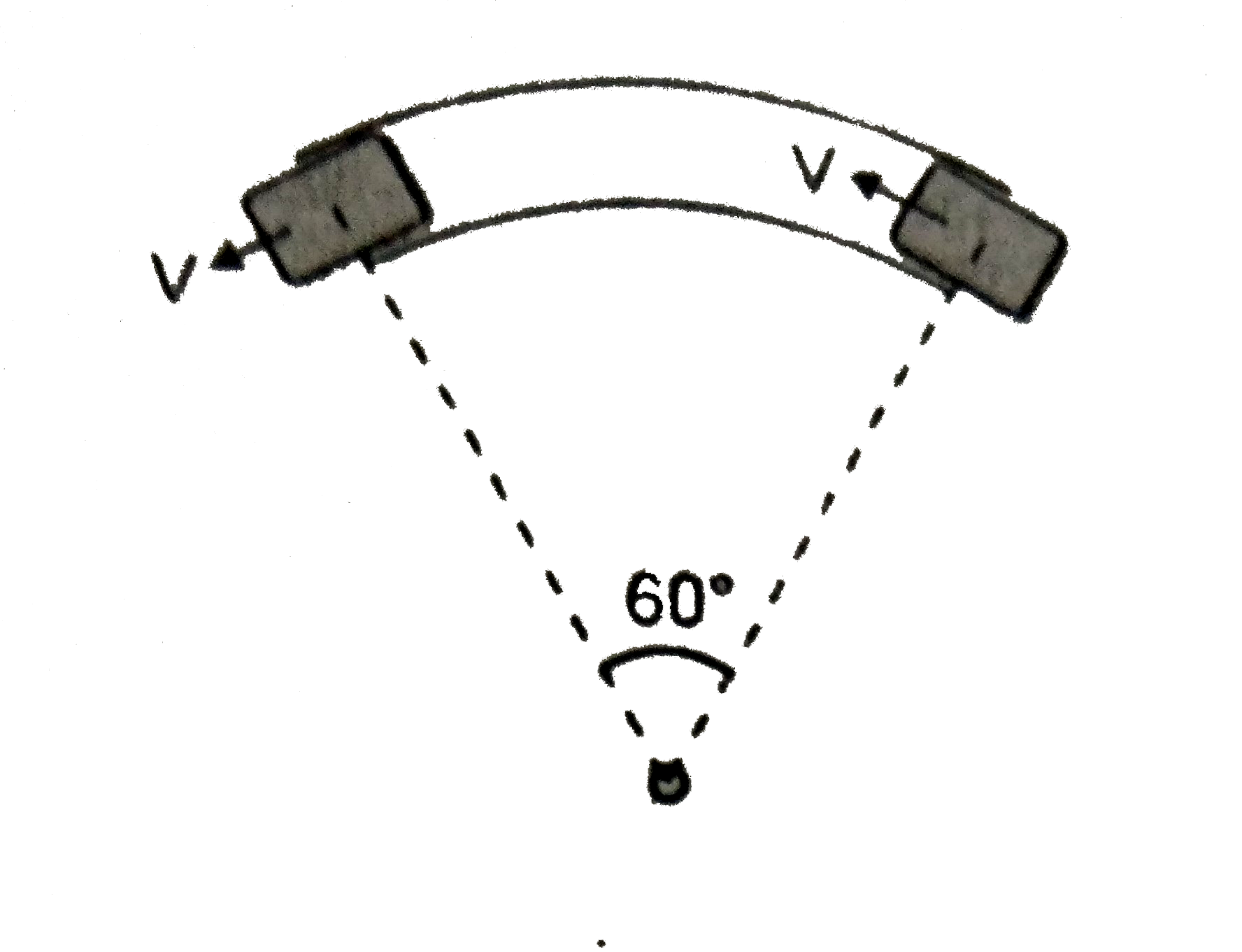
A car moves around a curve at a constant speed. When the car goes around the arc subtending $60^{\circ}$ at the centre, then the ratio of magnitude of instantaneous acceleration to average acceleration over the $60^{\circ}$ arc is $:$

A

$\frac{π}{3}$

B

$\frac{π}{6}$

C

$\frac{2 π}{3}$

D

$\frac{5 π}{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$| Δ V | = \sqrt{v^{2} + v^{2} - 2 v^{2} {cos 60}^{\circ}}$
$= v a_{a v} = \frac{| Δ \to v |}{Δ t} = \frac{v}{t} = \frac{3 v^{2}}{π R}$
$\Rightarrow a = \frac{v^{2}}{R}, \frac{a_{i}}{a_{a v}} = \frac{v^{2} π R}{R \times 3 v^{2}} = \frac{π}{3}$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-DAILY PRACTICE PROBLEM-DPP No.21

Home
Ask Doubt
Profile