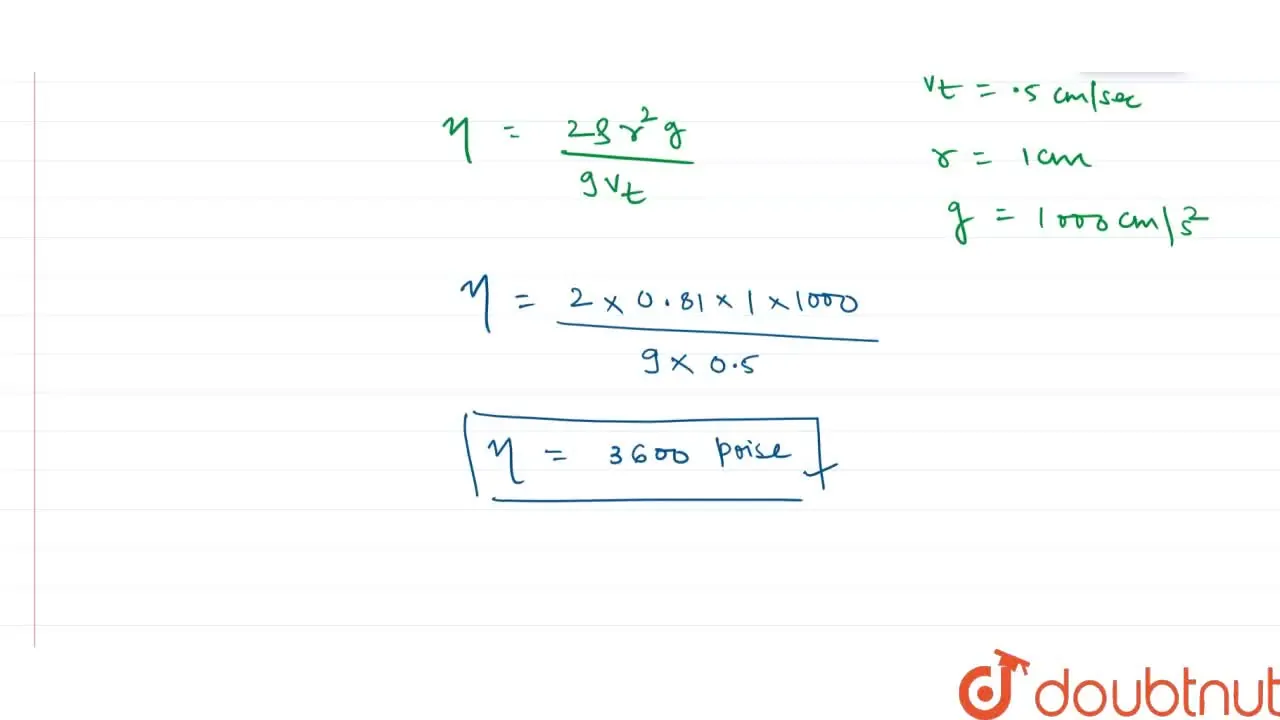
An air bubble of 1cm radius is rising at a steady rate of 0.5cms−1 through a liquid of density 0.81gcm−3. Calculate the coefficient of viscosity of the liquid. Neglect the density of air. ( Take g=10m/s2)
The correct Answer is:399 poise
Ans.360 poise
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 89 illustration5videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 90 Illustration2videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 887videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise53videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems9videos
ELASTICITY AND VISCOCITY
Similar Questions
An air bubble of 1 cm radius is rising at a steady rate of 2.00ms−1 through a liquid of density 1.5gcm−3. Neglect density of air. If g=1000cms−2, then the coeffieciet of viscosity of the liquid is
View SolutionAn air bubble of 1 cm radius is rising at a steady rate of 2.00ms−1 through a liquid of density 1.5gcm−3. Neglect density of air. If g=1000cms−2, then the coeffieciet of viscosity of the liquid is
View SolutionAn air bubble of radius 10−2 m is rising up at a steady rate of 2×10−3ms−1 through a liquid of density 1.5×103kgm−3 , the coefficient of viscosity neglecting the the density of air, will be (g=10ms−2)
View SolutionA gas bubble of diameter 002 m rises steadily at the rate of2.510−2ms−1 through a solution of density 2.5×103kgm−3. Calculate the coefficient of viscosity of the solution. Neglect the density of the gas.
View SolutionAn air bubble of diameter 2mm rises steadily through a solution of density 1750kgm−3 at the rate of 0.35cms−1 .Calculate the coefficient of viscosity of the solution (in Pascal×Sec).The density of air is negligible. (g=9.8ms2)
View SolutionA gas bubble of 2 cm diameter rises through a liquid 1.75gmcm−3 with a fixed speed of 0.35cms−1.Naglect the density of the gas. The cofficient of viscosity of the liquid is
View SolutionA lead sphere of 1.0mm diameter and relative density 11.20 attains a terminal velocity of 0.7cms−1 in a liquid of relative density 1.26.
Determine the coefficient of dynamic viscosity of the liquid.View SolutionA solid weighs 1.5 kgf in air and 0.9 kgf in a liquid of density 1.2×103kgm−3. Calculate R.D. of solid.
View SolutionAn air bubble of radius 5mm rises through a vat of syrup at a steady speed 2mm/s. If the syrup has a density of 1.4×103kg/m3, what is its viscosity ?
View SolutionA sphere of diameter 7.0 cm and mass 266.5 g float in a bath of liquid. As the temperature is raised, the sphere begins to sink at a temperature of 35∘C. If the density of liquid is 1.527gcm−3 at0∘C, find the coefficient of cubical expansion of the liquid. Neglect the expansion of the sphere.
View Solution