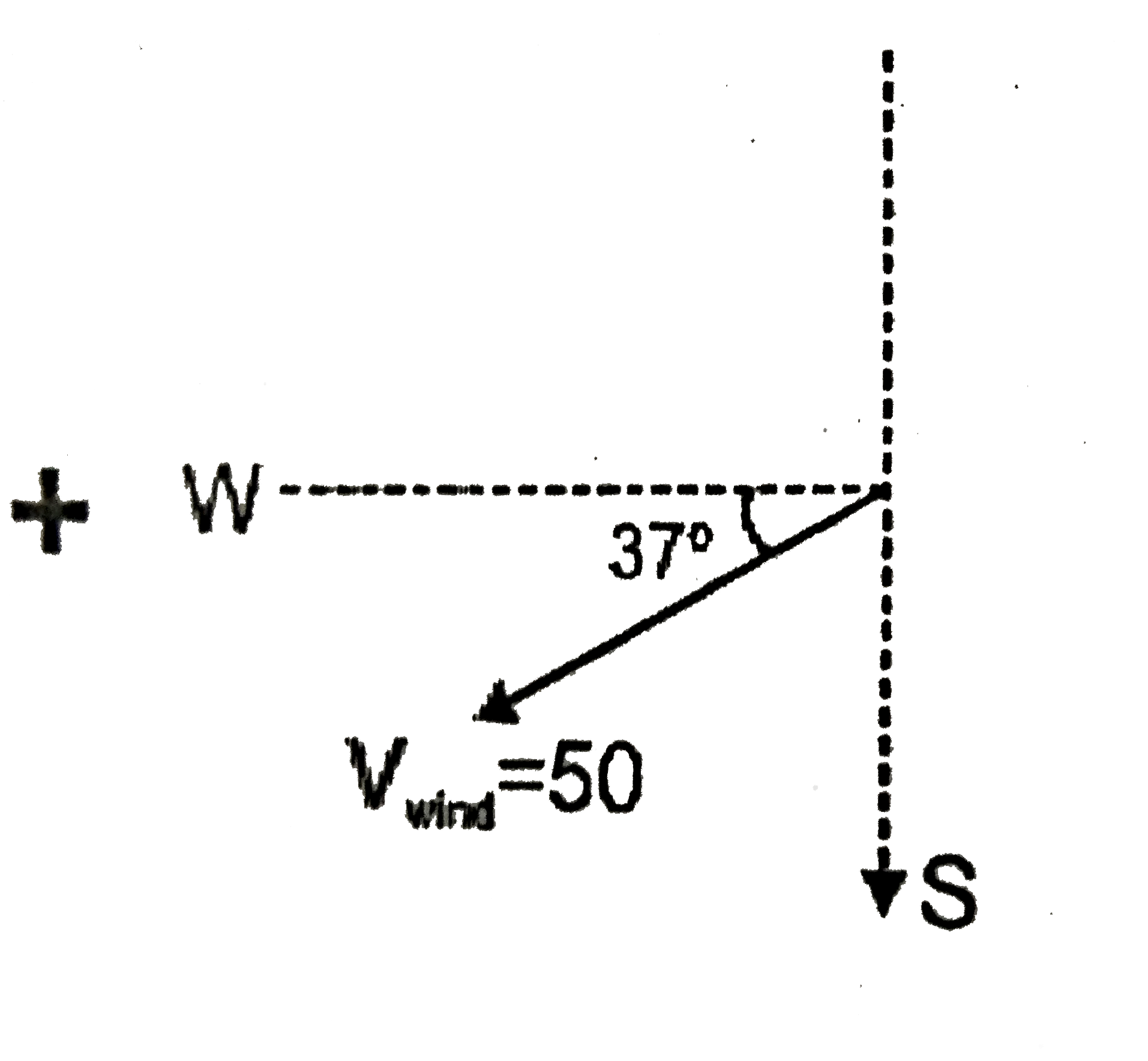
A man is on ship which is moving in east direction with speed 60km/hr. Waves of ocean is taking ship towards west with speed 20km/hr. Man start running on ship with flag in his hand in north direction with speed 30km/hr and wind is blowing with 50km/hr,37∘ towards south of west then find the direction of flutter the flag as seen by man on ground.
37∘ south to west
53∘ south of west
37∘ west of north
flag will not flutter
The correct Answer is:A
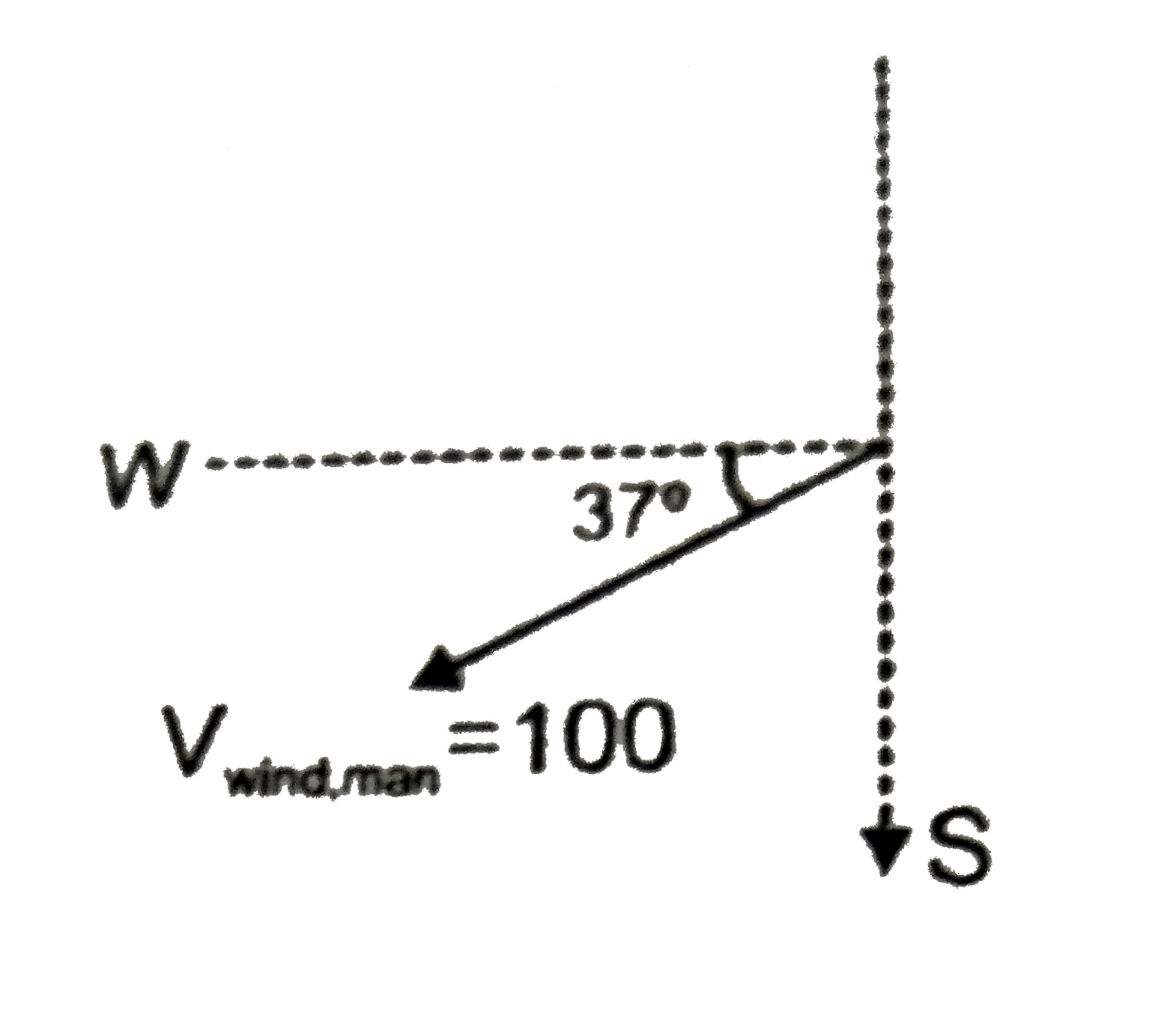
Flag will further in the direction of wind and opposite to the direction of velocity of man
i.e. in the direction of Vwm

Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 22.8videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp238videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 208videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise53videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems9videos
ELASTICITY AND VISCOCITY
Similar Questions
A man is moving due east with a speed 1 km/hr and rain is falling vertically with a speed √3 km/hr. At what angle from vertical the man has to hold his umbrella to keep the rain away. Also find the speed of rain drops w.r.t. man.
View SolutionA man rows a boat with a speed of 18 km/hr in northwest direction. The shoeline makes an angle of 15∘ south of west. Obtain the component of the velocity of the boat along the shoreline:
View SolutionTwo cars are moving in the same direction with the same speed 30km/hr. They are separated by a distance of 5km, the speed of a car moving in the opposite direction of it meets these two cars at an interval of 4 minutes, will be.
View SolutionTwo cars are moving in the same direction with the same speed 30km/hr. They are separated by a distance of 5km, the speed of a car moving in the opposite direction of it meets these two cars at an interval of 4 minutes, will be.
View SolutionA car moves with speed 60km/h for 1 hour in east direction and with same speed for 30 min in south direction. The displacement of car from initial posilion is
View SolutionA train moves in north direction with a speed of 54 km/hr. and a monkey running on the roof of the train, against its motion with a velocity of 18km/hr. with respect to the train, then the velocity of monkey as observed by a man standing on the ground:-
View SolutionA train moves in north direction with a speed of 54 km/hr. and a monkey running on the roof of the train, against its motion with a velocity of 18km/hr. with respect to the train, then the velocity of monkey as observed by a man standing on the ground:-
View SolutionIn a harbour, wind is blowing at the speed of 72km/h and the flag on the mast of a boat anchored in the harbour flutters along the N-Edirection. If the boat starts moving at a speed of 51km/h to the North, what is the direction of flag on the mast of the bat ?
View Solution2 km wide river flowing with a rate of 5 km/hr. A man can swim in the still water with10 km/hr. He wants to cross the river along shortest path find
In which direction person should be swimView SolutionA man sitting in a bus travelling in a direction from west to east with a speed of 40km/h observes that the rain-drops are falling vertically down. To the another man standing on ground the rain will appear
View Solution


