A particle is projected in a smooth fixed square tube from point A. The tube is in vertical plane and D and B are at same horizontal level as shown in figure. Then : (Assume velocity of particle changes smoothly at corners)
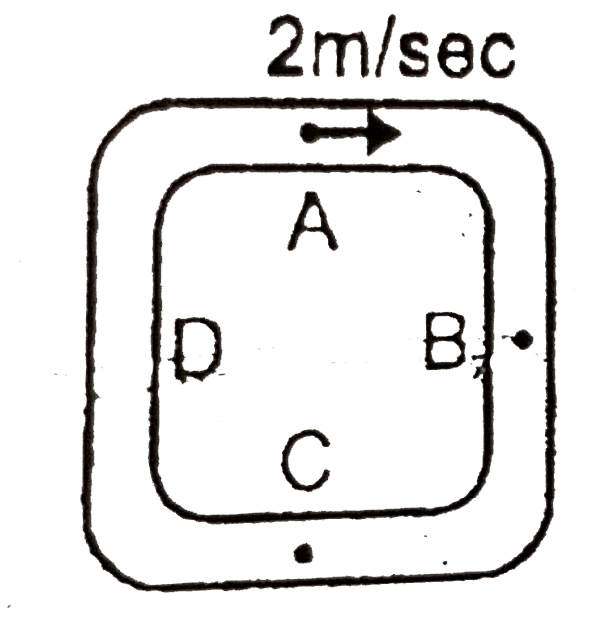
velocity at A is equal to velocity at C
velocity at B is equal to velocity at D
velocity at A is equal to negative of velocity at C
speed ast B is equal to speed at D
The correct Answer is:D
A particle is ………..
At B and D magnitude of velocity are same but direactions are different.
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
A particles is projected horizontally with a speed v from the top of a plane inclined at an angle tehta to the horizontal as shown in the figure.
(a) Hwo far from the point of projection will the particle strike the plane ?
(b) Find the time taken by the particel to hit the plane.
(c) What is the velocity of particle just before it hits the plane ?
View SolutionA particle is projected from a point A with velocity √2u an angle of 45∘ with horizontal as shown in figure. It strikes the plane BC at right angles. The velocity of the particle at the time of collision is

View SolutionTwo particles A and B moving in a plane have velocities as shown in figure .Relative velocity of A w.r.t B
View SolutionA particle in projected with velocity √3gL at point A (lowest point of the circle) in the vertical plane. Find the maximum height about horizontal level of point A if the string slacks at the point B as shown in figure.

View SolutionA particle is projected vertically upward with velocity u from a point A, when it returns to the point of projection .
View SolutionA particle of mass 0.1kg is launched at an angle of 53∘ with the horizontal . The particle enters a fixed rough hollow tube whose length is slightly less than 12.5m and which is inclined at an angle of 37∘ with the horizontal as shown in figure. It is known that the velocity of ball when it enters the tube is parallel to the axis of the tube. The coefficient of friction betweent the particle and tube inside the tube is μ=38[ Take g=10m/g2]

The distance from the point of projection where the particle will land on the horizontal plane after coming out from the tube is approximately equal to :
View SolutionA particle of mass 0.1kg is launched at an angle of 53∘ with the horizontal . The particle enters a fixed rough hollow tube whose length is slightly less than 12.5m and which is inclined at an angle of 37∘ with the horizontal as shown in figure. It is known that the velocity of ball when it enters the tube is parallel to the axis of the tube. The coefficient of friction betweent the particle and tube inside the tube is μ=38[ Take g=10m/g2]

The velocity of the particle as it enters the tube is :
View SolutionA particle of mass 0.1kg is launched at an angle of 53∘ with the horizontal . The particle enters a fixed rough hollow tube whose length is slightly less than 12.5m and which is inclined at an angle of 37∘ with the horizontal as shown in figure. It is known that the velocity of ball when it enters the tube is parallel to the axis of the tube. The coefficient of friction betweent the particle and tube inside the tube is μ=38[ Take g=10m/g2]

The kinetic energy of the particle when it comes out of the tube is approximately equal to :
View SolutionTwo particles are projected simultaneously from the same point, with the same speed, in the same vertical plane, and at different angles with the horizontal in a uniform gravitational field acting vertically downwards. A frame of reference is fixed to one particle. The position vector of the other particle , as observed from this frame, is →r. Which of the following statement is correct?
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View Solution
