In a certain gravity free space, the piston of an injection is being pushed so that the water jet comes out with a speed v. The area of the piston is much greater than the orifice of the injection.
The force required to be applied on the piston is proportional to v2.
The power developed by the force pushing the piston is proportional to v3.
The time for emptying the injection is proportional to v−1.
The total work done in emptying the injection is proportional to v2.
To solve the problem, we will analyze the situation step by step, applying the principles of fluid dynamics and mechanics.
Step 1: Understand the System
We have a piston in a cylinder that is pushing water through an orifice. The area of the piston (A) is much greater than the area of the orifice (a). The water exits the orifice with a speed v.
Step 2: Apply the Continuity Equation
According to the continuity equation, the flow rate must be constant throughout the system. Therefore, we can write:
A1V1=A2V2
Where:
- A1 is the area of the piston (A).
- V1 is the velocity of the piston.
- A2 is the area of the orifice (a).
- V2 is the velocity of the water jet (v).
Since the area of the piston is much greater than the area of the orifice, we can assume that V1 is much smaller than v. Thus, we can neglect V1 in our calculations.
Step 3: Relate Velocities
From the continuity equation, we have:
AV1=av
Rearranging gives us:
V1=aAv
Since A≫a, V1 will be very small.
Step 4: Apply Bernoulli's Equation
Using Bernoulli's equation between the piston and the orifice, we have:
P1+12ρV21=P2+12ρv2
Assuming P2 is atmospheric pressure (which we can treat as zero gauge pressure), we can write:
P1+12ρV21=12ρv2
Step 5: Express Pressure in Terms of Force
The pressure P1 can be expressed in terms of the force F applied on the piston:
P1=FA
Substituting this into the Bernoulli equation gives:
FA+12ρV21=12ρv2
Step 6: Solve for Force
Neglecting V1 (since it is very small), we find:
FA=12ρv2
Thus, rearranging gives us:
F=12ρv2A
Step 7: Calculate Power
Power P is defined as the product of force and velocity:
P=F⋅V1
Substituting F:
P=(12ρv2A)⋅(aAv)=12ρav3
Step 8: Calculate Time for Emptying
The time T to empty a volume V can be calculated using:
T=VQ
Where Q is the flow rate Q=av:
T=Vav
Thus, T is directly proportional to 1v.
Step 9: Work Done
The work done W can be calculated from the change in kinetic energy:
W=ΔKE=12mv2
Where m is the mass of water flowing out, which is constant.
Summary of Results
1. Force: F=12ρv2A
2. Power: P=12ρav3
3. Time for emptying: T∝1v
4. Work done: W=12mv2
We have a piston in a cylinder that is pushing water through an orifice. The area of the piston (A) is much greater than the area of the orifice (a). The water exits the orifice with a speed v.
According to the continuity equation, the flow rate must be constant throughout the system. Therefore, we can write:
- A1 is the area of the piston (A).
- V1 is the velocity of the piston.
- A2 is the area of the orifice (a).
- V2 is the velocity of the water jet (v).
From the continuity equation, we have:
Using Bernoulli's equation between the piston and the orifice, we have:
The pressure P1 can be expressed in terms of the force F applied on the piston:
Neglecting V1 (since it is very small), we find:
Power P is defined as the product of force and velocity:
The time T to empty a volume V can be calculated using:
The work done W can be calculated from the change in kinetic energy:
1. Force: F=12ρv2A
2. Power: P=12ρav3
3. Time for emptying: T∝1v
4. Work done: W=12mv2
Topper's Solved these Questions
Similar Questions
A long cylindrical tank of cross-sectional area 0.5m2 is filled with water. It has a small hole at a height 50cm from the bottom. A movable piston of cross-sectional area almost equal to 0.5m2 is fitted on the top of the tank such that it can slide in the tank freely. A load of 20kg is applied on the top of the water by piston, as shown in the figure. Calculate the speed of the water jet with which it hits the surface when piston is 1m above the bottom. (Ignore the mass of the piston)

View SolutionFigure shows a liquid being pushed out of a tube by pressing a piston. The area of cross section of the piston is 1.0 cm^2 and that of the tube at the outlet is 20mm2. If the piston is pushed at a speed of 2cms−1. What is the speed of the outgoing liquid?

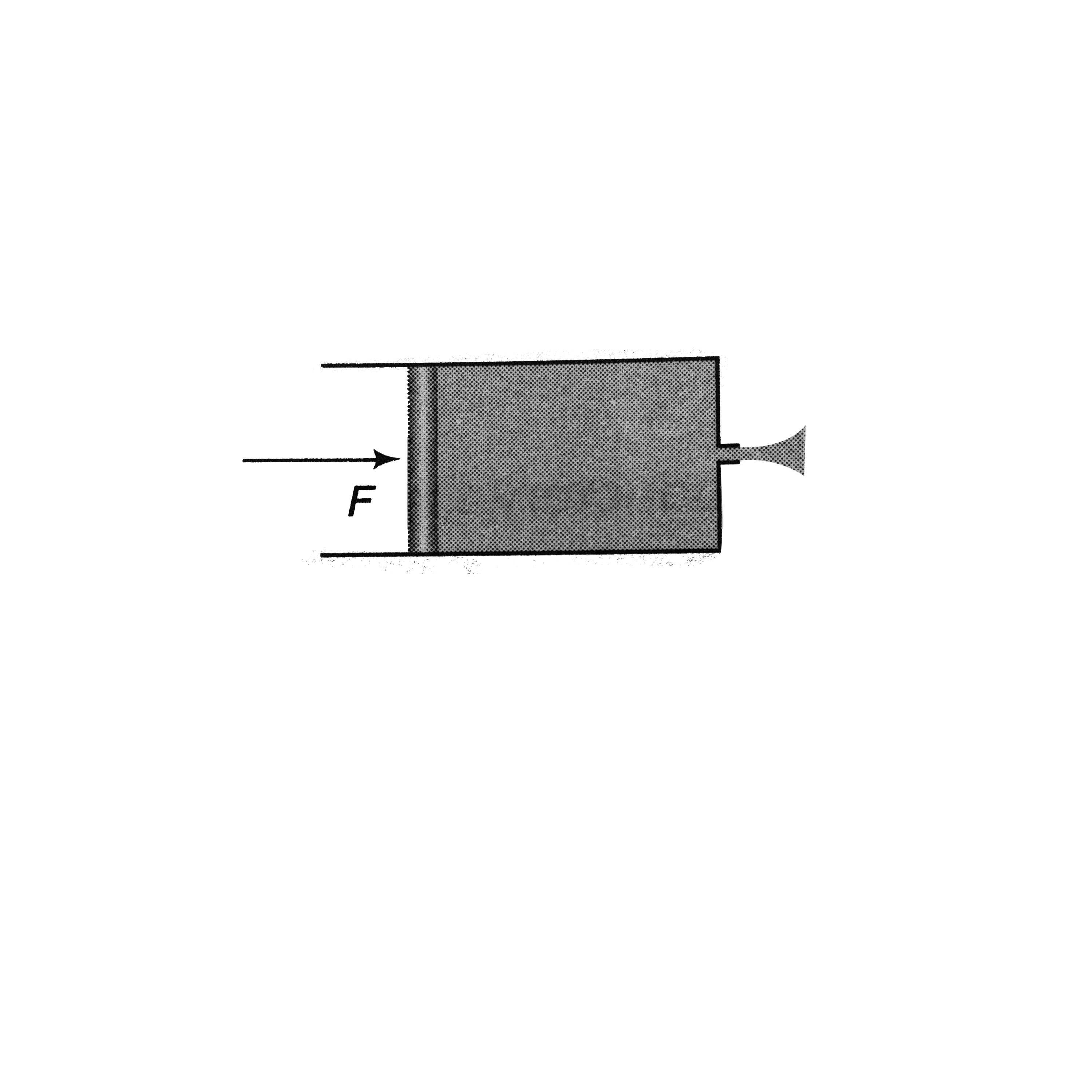
View SolutionWhat work should be done in order to squeeze all water from a horizontally located cylinder (figure) during the time t by means of a constant force acting on the piston? The volume of water in the cylinder is equal to V, the cross-sectional area of the orifice is s, with s being considerably less than the piston area. The friction and viscosity are negligibly small. Density of water is ρ
View SolutionA spray gun is shown in the figure where a piston pushes air out of a nozzle. A thin tube of uniform cross section is connected to the nozzle. The other end of the tube is connected with a small liquid container. As the piston pushes air through the nozzle, the liquid from the container rises into the nozzle and is sprayed out. For the spray gun shown, the radii of the piston and the nozzle are 20 mm and 1 mm respectively. The upper end of the container is open to the atmosphere.
If the piston is pushed at a speed of 5mms−1 the air comes out of the nozzle with a speed of :
View Solution_E01_216_Q01.png)
A syringe containing water is held horizontally with its nozzle at a height h above the ground as shown in fig the cross sectional areas of the piston and the nozzle are A and a respectively the piston is pushed with a constant speed v. Find the horizontal range R of the stream of water on the ground.
View SolutionA water fountain on the ground sprinkles water all around it. If the speed of water coming out of the fountains is v, the total area around the fountain that gets wet is:
View SolutionA water fountain on the ground sprinkles water all around it . If the speed of water coming out of the fountain is v , the total area around the fountain that gets wet is :
View SolutionA cylindrical tube with a frictionless piston ends in a small hole of area of cross–section 2×10−6m2, which is very small as compared to the area of cross-section of the tube. The tube is filled with a liquid of density 104kg/m3. The tube is held horizontal and the piston is pushed at a constant speed so that liquid comes out of the hole at a constant rate 'Q'm3/s. No liquid leaks around the piston. The material of the tube can bear a maximum pressure 6×105 Pa. The maximum possible value of Q so that the tube does not break is __________ ×10−5m3/s. [Atmospheric pressure =105 Pa]

View SolutionAn inquistive student, determined to test the law of gravity for himself, walks to the top of a building og 145 floors, with every floor of height 4 m, having a stopwatch in his hand (the first floor is at a height of 4 m from the ground level). From there he jumps off with negligible speed and hence starts rolling freely. A rocketeer arrives at the scene 5 s later and dives off from the top of the building to save the student. The rocketeer leaves the roof with an initial downward speed v0. In order to catch the student at a sufficiently great height above ground so that the rocketeer and the student slow down and arrive at the ground with zero velocity. The upward acceleration that accomplishes this is provided by rocketeer's jet pack, which he turns on just as he catches the student, before the rocketeer is in free fall. To prevent any discomfort to the student, the magnitude of the acceleration of the rocketeer and the student as they move downward together should not exceed 5 g.
Just as the student starts his free fall, he presses the button of the stopwatch. When he reaches at the top of 100th floor, he has observed the reading of stopwatch as 00:00:06:00 (hh:mm:ss:100 th part of the second). Find the value of g. (correct upt ot two decimal places).
View SolutionThe word fluid means a substance having particles which readily of its magnitude (a small shear stress, which may appear to be of negligible will cause deformation in the fluid). Fluids are charactrised by such properties as density. Specific weight, specific gravity, viscosity etc. Density of a substance is defined as mass per unit volume and it is denoted by. The specific gravity represents a numerical ratio of two densities, and water is commonly taken as a reference substance. Specific gravity of a substance in written as the ratio of density of substance to the density of water. Specific weight represents the force exerted by gravity on a unit volume of fluid. It is related to the density as the product of density of a fluid and acceleration due to gravity. Viscosity is the most important and is recognized as the only single property which influences the fluid motion to a great extent. The viscosity is the property by virtue of which a fluid offers resistance to deformation under the influenece if shear force. The force between the layers opposing relative motion between them are known as forces of viscosity. When a boat moves slowly on the river remains at rest. Velocities of different layers are different. Let v be the velocity of the level at a distance y from the bed and V+dv be the velocity at a distance y+dy. The velocity differs by dv in going through a distance by perpendicular to it. The quantity dvdy is called velocity gradient. The force of viscosity between two layers of a fluid is proportional to velocity gradient and Area of the layer.
F∝A & F∝dvdy
F=−ηAdvdy (−ve sign shown the force is frictional in nature and opposes relative motion. η coefficient of dynamic viscosity
Shear stress FA=−ηdvdy
and simultaneously kinematic viscosity is defined as the dynamic viscosity divided by the density. If is denoted as v. The viscosity of a fluid depends upon its intermolecular structure. In gases, the molecules are widely spaced resulting in a negligible intermolecular cohesion, while in liquids the molecules being very close to each other, the cohesion is much larger with the increases of temperature, the cohesive force decreases rapidly resulting in the decreases of viscosity. In case of gases, the viscosity is mainly due to transfer of molecular momentum in the transerve direction brought about by the molecular agitation. Molecular agitation increases with rise in temperature. Thus we conclude that viscosity of a fluid may thus be considered to be composed of two parts, first due to intermolecuar cohesion and second due to transfer of molecular momentum.
If the velocity profile is given by
v=23y−y2v is velocity in m/secy is in meter above the bad. Determine shear stress at y=0.15m, & η=0.863Ns/m2
View Solution
